Saturation of atomic transitions

Saturation

The saturated transition rate

Consider an ensemble of $n$ two-lvel atoms with levels $|a{\rangle }$ and $|b{\rangle }$:

$\Gamma$ is the spontaneous emission rate, and $R^{U}$ is the transition rate given by the coupling between incident photons and the atoms. We call $R^{u}$ the unsaturated transition rate, because the actual net transfer rate from $|a{\rangle }$ to $|b{\rangle }$, is close to $R^{u}$ only when most atoms start in state $|a{\rangle }$. When a sizable fraction of the $n$ atoms are already excited, the net transfer rate slows down. Eventually, upon sufficient excitation, the number of atoms in the two states, $n_{a}$ and $n_{b}$ no longer change. Between these two extremes, it is useful to define a saturated transition rate $R^{s}$, which gives the net transfer rate from $|a{\rangle }$ to $|b{\rangle }$ caused by incident photons, per atom in the ensemble. What is a mathematical expression for $R^{s}$? The rate equations governing the atomic populations are:

${\begin{array}{rcl}{\dot {n}}_{b}&=&-n_{b}(R^{u}+\Gamma )+n_{a}R^{u}\\{\dot {n}}_{a}&=&n_{b}(R^{u}+\Gamma )-n_{a}R^{u}\,.\end{array}}$

In the absence of spontaneous emission, these eqautions show that the net transition rate between the levels is given by $R^{u}(n_{a}-n_{b})$, so that the saturated transition rate is

${\begin{array}{rcl}R^{s}&=&R^{u}{\frac {n_{a}-n_{b}}{n_{a}+n_{b}}}\\&=&R^{u}{\frac {1-{\frac {n_{b}}{n_{a}}}}{1+{\frac {n_{b}}{n_{a}}}}}\,.\end{array}}$

The steady state populations, obtained by setting ${\dot {n}}_{a}$ and ${\dot {n}}_{b}$ to zero, satisfy

${\frac {n_{b}}{n_{a}}}={\frac {R^{u}}{\Gamma +R^{u}}}\,,$

which can be conveneintly re-expressed as

${\frac {n_{b}}{n_{a}}}={\frac {s}{2+s}}\,,$

by defining the saturation parameter

$s={\frac {2R^{u}}{\Gamma }}\,.$

We will return later to the physical meaning of the saturation parameter. Subsituting this into the definition of $R^{s}$ gives

${\begin{array}{rcl}R^{s}&=&{\frac {\Gamma s}{2}}{\frac {1-s/(2+s)}{1+s/(2+s)}}\\&=&{\frac {\Gamma s}{2}}{\frac {2+s-s}{2+s+s}}\\&=&{\frac {\Gamma s}{2}}{\frac {2}{2+2s}}\\&=&{\frac {\Gamma }{2}}{\frac {s}{1+s}}\,.\end{array}}$

This is a very useful expression. In the limit of $R^{u}\ll \Gamma$ (small $s$), far from saturation, $R^{s}\rightarrow R^{u}$, and in the limit of $R^{u}\gg \Gamma$ ($s\rightarrow \infty$), complete saturation, $R^{s}\rightarrow \Gamma /2$.

Monochromatic Radiation

An important case is when the incident radiation is monochromatic, and has a Lorentzian frequency distribution, $f(\delta )$, where $\delta$ is the detuning from resonance. Recall that the unsaturated transition rate is then given by

$R^{u}(\delta )={\frac {\pi }{2}}\omega _{R}^{2}f(\delta )\,,$

where $\omega _{R}$ is the Rabi frequency. Substituting for $f(\delta )$ a normalized Lorentzian distribution,

$f(\delta )={\frac {2}{\pi }}\,{\frac {1/\Gamma }{1+(2\delta /\Gamma )^{2}}}\,,$

we obtain

$R^{u}(\delta )={\frac {\omega _{R}^{2}}{\Gamma }}\,{\frac {1}{1+(2\delta /\Gamma )^{2}}}\,,$

and this expression for the saturation parameter

$s(\delta )={\frac {2\omega _{R}^{2}}{\Gamma ^{2}}}{\frac {1}{1+(2\delta /\Gamma )^{2}}}\,.$

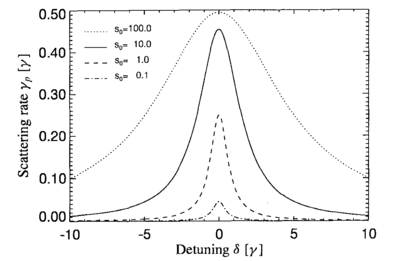
The saturated transition rate per atom, as a function of detuning, is thus

${\begin{array}{rcl}R^{s}(\delta )={\frac {\omega _{R}^{2}}{\Gamma }}{\frac {1}{1+(2\delta /\Gamma )^{2}+2(\omega _{R}/\Gamma )^{2}}}\,.\end{array}}$

This is an important and useful expression. It tells us what the transition rate is, as measured in an experiment, for example, by absorption. Note that this is a Lorentzian distribution, with a width which depends on $\omega _{R}$. Defining the on-resonance saturation parameter

$s_{0}={\frac {2\omega _{R}^{2}}{\Gamma ^{2}}}\,,$

such that the saturation parameter is $s=s_{0}/(1+(2\delta /\Gamma )^{2})$, we find that the full width at half maximum of $R^{s}(\delta )$ is given by

$\Gamma '=\Gamma {\sqrt {1+s_{0}}}\,.$

indicating that the transition broadens in frequency as the incident intensity of light (which determines $\omega _{R}$) increases. Physically, this happens because of the effective shortening of the lifetime of atoms in $|b{\rangle }$ due to the stimulated emission. The saturated transition rate per atom, expressed in terms of $s_{0}$, is

${\begin{array}{rcl}R^{s}(\delta )={\frac {s_{0}\Gamma /2}{1+s_{0}+(2\delta /\Gamma )^{2}}}\,.\end{array}}$

and can be re-expressed using the power broadened linewidth $\Gamma '$ as

${\begin{array}{rcl}R^{s}(\delta )={\frac {s_{0}}{1+s_{0}}}{\frac {\Gamma /2}{1+(2\delta /\Gamma ')}}\,.\end{array}}$

Note that in the limit that $s_{0}\rightarrow \infty$, $R^{s}\rightarrow \Gamma /2$. A plot of this is shown below (figure from page 26 of {\em Laser Cooling and Trapping}, by Metcalf and van der Straten; $\gamma _{p}=R^{s}$):

Note that the transition rate from $|a{\rangle }$ to $|b{\rangle }$, given in terms of the absorption crosssection $\sigma _{\rm {abs}}^{u}$, and the incident intensity $I_{0}$, is

$W_{a\rightarrow b}=\sigma _{\rm {abs}}^{u}{\frac {1}{1+s}}{\frac {I_{0}}{\hbar \omega }}\,,$

where we may identify as the saturated absorption crossection

$\sigma _{\rm {abs}}^{s}=\sigma _{\rm {abs}}^{u}{\frac {1}{1+s}}\,.$

As $s\rightarrow \infty$, $\sigma _{\rm {abs}}^{s}\rightarrow 0$. This process is known as bleaching.

Saturation Intensity

The on-resonance saturation parameter has a useful physical meaning. At $s_{0}=1$, the unsaturated transition rate becomes

$R^{u}=\Gamma /2=\sigma _{\rm {abs}}{\frac {I_{\rm {sat}}}{\hbar \omega }}\,,$

were we have identified as the sauration intensity

$I_{\rm {sat}}={\frac {\hbar \omega ^{3}}{12\pi c^{2}}}\Gamma \,.$

This is a very useful quantity in practice. For the sodium D line, $I_{\rm {sat}}=6$ mW/cm$^{2}$, and that value is typical of most widely used atomic transitions. In terms of $I_{\rm {sat}}$, we may give

${\begin{array}{rcl}s_{0}&=&{\frac {I}{I_{\rm {sat}}}}\\\omega _{R}^{2}&=&{\frac {\Gamma ^{2}}{2}}{\frac {I}{I_{\rm {sat}}}}\,.\end{array}}$

This extrodinary $\sim 10$ mW/cm$^{2}$ value of $I_{\rm {sat}}$ can be apprreciated by comparing the monochromatic excitation case, which we have just analyzed, with the case of broadband excitation. For broadband excitation,

${\begin{array}{rcl}W_{a\rightarrow b}&=&\sigma _{0}{\frac {\pi }{2}}f(\delta )I{\frac {\Gamma }{\hbar \omega }}\\&\approx &{\frac {\Gamma }{2}}\,,\end{array}}$

where the approximation is valid in the limit that the average intensity over the spectral density ${\bar {I}}=f(\delta )I\approx {\bar {I}}_{\rm {sat}}$ (a useful approximation which gives a result independent of the details of the transition). Here,

${\bar {I}}_{\rm {sat}}={\frac {\hbar \omega ^{3}}{6\pi ^{2}c^{2}}}\,,$

which, for visible frequencies, is approximately $12$ W/cm$^{2}$ per inverse centimeter of bandwidth.