Difference between revisions of "Single photons"
imported>Ichuang |
imported>Ichuang |
||
| (15 intermediate revisions by 2 users not shown) | |||
| Line 27: | Line 27: | ||
a different velocity. For example: | a different velocity. For example: | ||
::[[Image:chapter2-quantum-light-part-3-phasesh2.png|thumb|500px|none|]] | ::[[Image:chapter2-quantum-light-part-3-phasesh2.png|thumb|500px|none|]] | ||
| − | |||
depicts two modes, in which the top photon has its phase shifted by | depicts two modes, in which the top photon has its phase shifted by | ||
<math>\pi</math> relative to the bottom one. It is clear that any relative phase | <math>\pi</math> relative to the bottom one. It is clear that any relative phase | ||
| Line 49: | Line 48: | ||
\,. | \,. | ||
</math> | </math> | ||
| + | |||
We may depict this as: | We may depict this as: | ||
::[[Image:chapter2-quantum-light-part-3-qbs.png|thumb|500px|none|]] | ::[[Image:chapter2-quantum-light-part-3-qbs.png|thumb|500px|none|]] | ||
| − | |||
Note the use of a <math>\cdot</math> to distinguish the ports; this is needed | Note the use of a <math>\cdot</math> to distinguish the ports; this is needed | ||
because we have adopted a phase convention for the beamsplitter which | because we have adopted a phase convention for the beamsplitter which | ||
| Line 139: | Line 138: | ||
Omitting the vacuum state <math>|00{\rangle}</math>, the two-mode state space we shall | Omitting the vacuum state <math>|00{\rangle}</math>, the two-mode state space we shall | ||
consider thus has a basis state spanned by <math>|01{\rangle}</math>, and <math>|10{\rangle}</math>. We | consider thus has a basis state spanned by <math>|01{\rangle}</math>, and <math>|10{\rangle}</math>. We | ||
| − | call this the dual-rail photon state space. Note that an | + | call this the dual-rail photon state space (this Hilbert space behaves much like a [[Quantized spin in a magnetic field|a two-level system]]). Note that an |
arbitrary state in this space as <math>|\psi \rangle = \alpha |01 \rangle + \beta | arbitrary state in this space as <math>|\psi \rangle = \alpha |01 \rangle + \beta | ||
|10{\rangle}</math>, where <math>\alpha</math> and <math>\beta</math> are complex numbers satisfying | |10{\rangle}</math>, where <math>\alpha</math> and <math>\beta</math> are complex numbers satisfying | ||
<math>|\alpha|^2 +|\beta|^2 = 1</math>. | <math>|\alpha|^2 +|\beta|^2 = 1</math>. | ||
| − | From | + | From Bloch's Theorem, it follows that any dual-rail photon state can |
be generated using phase shifters and beamsplitters. Specifically, | be generated using phase shifters and beamsplitters. Specifically, | ||
if we write <math>|\psi{\rangle}</math> as a two-component vector, then the action of <math>B</math> | if we write <math>|\psi{\rangle}</math> as a two-component vector, then the action of <math>B</math> | ||
| Line 162: | Line 161: | ||
</math> | </math> | ||
where the overall phase <math>e^{i\theta/2}</math> is irrelevant and can be | where the overall phase <math>e^{i\theta/2}</math> is irrelevant and can be | ||
| − | dropped in the following. Let us define the Pauli matrices as | + | dropped in the following. |
| + | |||
| + | ===== Arbitrary single qubit operations with phase shifters and beam splitters ===== | ||
| + | |||
| + | ====== Employing Pauli matrices ====== | ||
| + | |||
| + | Let us define the Pauli matrices as | ||
:<math>\begin{array}{rcl} | :<math>\begin{array}{rcl} | ||
X & = & \left[ \begin{array}{cc}{0}&{1}\\{1}&{0}\end{array}\right] \\ | X & = & \left[ \begin{array}{cc}{0}&{1}\\{1}&{0}\end{array}\right] \\ | ||
| Line 169: | Line 174: | ||
\,. | \,. | ||
\end{array}</math> | \end{array}</math> | ||
| + | |||
| + | ====== Phase shifter and Beamsplitter operators ====== | ||
| + | |||
In terms of these, we find that the phase shifter and beamsplitter | In terms of these, we find that the phase shifter and beamsplitter | ||
operators may be expressed as | operators may be expressed as | ||
| Line 177: | Line 185: | ||
\,. | \,. | ||
\end{array}</math> | \end{array}</math> | ||
| + | |||
| + | ====== Rotations of a two-level quantum system ====== | ||
| + | |||
These are rotations of a two-level system, about the axes | These are rotations of a two-level system, about the axes | ||
<math>\hat{y}</math> and <math>\hat{z}</math>, by angles <math>2\theta</math> and <math>\phi</math>, | <math>\hat{y}</math> and <math>\hat{z}</math>, by angles <math>2\theta</math> and <math>\phi</math>, | ||
| Line 204: | Line 215: | ||
\end{array}</math> | \end{array}</math> | ||
| − | ==== Bloch's Theorem ==== | + | ====== Bloch's Theorem ====== |
In terms of these, Bloch's Theorem states the following: | In terms of these, Bloch's Theorem states the following: | ||
| − | + | ||
| + | {| border="1" style="width:75%; color:blue" align="center" | ||
| + | | Theorem: (<math>Z</math>-<math>Y</math> decomposition of rotations) | ||
Suppose <math>U</math> is a unitary operation on a two-dimensional Hilbert | Suppose <math>U</math> is a unitary operation on a two-dimensional Hilbert | ||
space. Then there exist real numbers <math>\alpha,\beta,\gamma</math> and | space. Then there exist real numbers <math>\alpha,\beta,\gamma</math> and | ||
| Line 214: | Line 227: | ||
U = e^{i\alpha} R_z(\beta) R_y(\gamma) R_z(\delta). | U = e^{i\alpha} R_z(\beta) R_y(\gamma) R_z(\delta). | ||
</math> | </math> | ||
| − | + | |} | |
| − | + | ||
| − | + | Proof:~ | |
Since <math>U</math> is unitary, the rows and columns of <math>U</math> are orthonormal, from which | Since <math>U</math> is unitary, the rows and columns of <math>U</math> are orthonormal, from which | ||
it follows that there exist real numbers <math>\alpha,\beta,\gamma</math>,and <math>\delta</math> | it follows that there exist real numbers <math>\alpha,\beta,\gamma</math>,and <math>\delta</math> | ||
| Line 232: | Line 245: | ||
Equation (\ref{eqtn:alg:qubit_decomp}) now follows immediately from the | Equation (\ref{eqtn:alg:qubit_decomp}) now follows immediately from the | ||
definition of the rotation matrices and matrix multiplication. | definition of the rotation matrices and matrix multiplication. | ||
| − | |||
What we have just done, expressed in modern language, is to introduce | What we have just done, expressed in modern language, is to introduce | ||
| Line 238: | Line 250: | ||
single qubit operations ("gates") can be performed using | single qubit operations ("gates") can be performed using | ||
phase-shifters and beamsplitters. | phase-shifters and beamsplitters. | ||
| + | |||
For example, one widely useful single-qubit transform is the Hadamard | For example, one widely useful single-qubit transform is the Hadamard | ||
gate, | gate, | ||
| Line 260: | Line 273: | ||
interferometers, which will be ubiquitous through our treatment of | interferometers, which will be ubiquitous through our treatment of | ||
atoms and quantum information. | atoms and quantum information. | ||
| + | |||
Let us begin by developing a model for the Mach-Zehnder | Let us begin by developing a model for the Mach-Zehnder | ||
interferometer, which is constructed from two beamsplitters. Recall | interferometer, which is constructed from two beamsplitters. Recall | ||
that two beamsplitters <math>B</math> and <math> {B}^\dagger </math>, configured as | that two beamsplitters <math>B</math> and <math> {B}^\dagger </math>, configured as | ||
| − | ::[[Image:chapter2-quantum-light-part-3-qbsiden.png|thumb| | + | ::[[Image:chapter2-quantum-light-part-3-qbsiden.png|thumb|500px|none|]] |
| − | |||
naturally leave the output identical to the input, as <math> {B}^\dagger B = I</math>. | naturally leave the output identical to the input, as <math> {B}^\dagger B = I</math>. | ||
If a phase shifter <math>P(\phi)</math> is placed inbetween two <math>50/50</math> | If a phase shifter <math>P(\phi)</math> is placed inbetween two <math>50/50</math> | ||
beamsplitters, | beamsplitters, | ||
| − | ::[[Image:chapter2-quantum-light-part-3-qbsintrf.png|thumb| | + | ::[[Image:chapter2-quantum-light-part-3-qbsintrf.png|thumb|500px|none|]] |
| − | |||
then the input is transformed by | then the input is transformed by | ||
:<math>\begin{array}{rcl} | :<math>\begin{array}{rcl} | ||
| Line 282: | Line 294: | ||
It is convenient to visualize this sequence of three rotations on the | It is convenient to visualize this sequence of three rotations on the | ||
Bloch sphere: | Bloch sphere: | ||
| − | ::[[Image:chapter2-quantum-light-part-3-blochsphere2.png|thumb| | + | ::[[Image:chapter2-quantum-light-part-3-blochsphere2.png|thumb|500px|none|]] |
| − | |||
The first <math>R_y(-\pi/2)</math> rotates <math>\hat{z}</math> into <math>\hat{x}</math>, and | The first <math>R_y(-\pi/2)</math> rotates <math>\hat{z}</math> into <math>\hat{x}</math>, and | ||
<math>-\hat{x}</math> into <math>\hat{z}</math>. The system is then rotated around | <math>-\hat{x}</math> into <math>\hat{z}</math>. The system is then rotated around | ||
| Line 300: | Line 311: | ||
(the interferometer is "balanced"), the input is unchanged, and when | (the interferometer is "balanced"), the input is unchanged, and when | ||
<math>\phi=\pi</math>, the two modes are swapped. | <math>\phi=\pi</math>, the two modes are swapped. | ||
| + | |||
=== Nonlinear Mach-Zehnder interferometer === | === Nonlinear Mach-Zehnder interferometer === | ||
The two components we have studied so far, phase shifters and | The two components we have studied so far, phase shifters and | ||
| Line 310: | Line 322: | ||
for creating quantum states such as squeezed light. What do nonlinear | for creating quantum states such as squeezed light. What do nonlinear | ||
optical elements do to single photons? | optical elements do to single photons? | ||
| + | |||
| + | ==== Optical Kerr media ==== | ||
| + | |||
Consider a material with <math>\chi\sim \chi^{(3)}\neq 0</math>, which we may | Consider a material with <math>\chi\sim \chi^{(3)}\neq 0</math>, which we may | ||
model as having the Hamiltonian | model as having the Hamiltonian | ||
| Line 327: | Line 342: | ||
modulation Hamiltonian, and the nonlinear crystal as being a Kerr | modulation Hamiltonian, and the nonlinear crystal as being a Kerr | ||
medium. | medium. | ||
| + | |||
| + | ==== Interferometer with Kerr medium inside ==== | ||
| + | |||
Interesting non-classical behavior can be obtained using | Interesting non-classical behavior can be obtained using | ||
interferometers constructed with Kerr media used as nonlinear phase | interferometers constructed with Kerr media used as nonlinear phase | ||
| Line 337: | Line 355: | ||
\,. | \,. | ||
\end{array}</math> | \end{array}</math> | ||
| + | |||
Let us take <math>\chi L = \pi</math>, such that <math>K|11 \rangle = -|11{\rangle}</math>. | Let us take <math>\chi L = \pi</math>, such that <math>K|11 \rangle = -|11{\rangle}</math>. | ||
Suppose we now place the Kerr medium inside a Mach-Zehnder | Suppose we now place the Kerr medium inside a Mach-Zehnder | ||
interferometer in this manner: | interferometer in this manner: | ||
| − | ::[[Image:chapter2-quantum-light-part-3-fredkin-gate-15nov94.png|thumb| | + | ::[[Image:chapter2-quantum-light-part-3-fredkin-gate-15nov94.png|thumb|500px|none|]] |
\noindent | \noindent | ||
Intuitively, we expect that when no photons are input into <math>c</math>, then | Intuitively, we expect that when no photons are input into <math>c</math>, then | ||
| Line 347: | Line 366: | ||
modulation due to <math>K</math> is sufficiently large (<math>\sim \pi</math>), then the | modulation due to <math>K</math> is sufficiently large (<math>\sim \pi</math>), then the | ||
inputs are swapprd, producing <math>a'=b</math> and <math>b'=a</math>. | inputs are swapprd, producing <math>a'=b</math> and <math>b'=a</math>. | ||
| + | |||
Mathematically, we may write the transform performed by this nonlinear | Mathematically, we may write the transform performed by this nonlinear | ||
Mach-Zehnder interferometer as the unitary transform <math>U = {B}^\dagger K B</math>, | Mach-Zehnder interferometer as the unitary transform <math>U = {B}^\dagger K B</math>, | ||
| Line 355: | Line 375: | ||
:<math>\begin{array}{rcl} | :<math>\begin{array}{rcl} | ||
U &=& | U &=& | ||
| − | \exp \left[ { i\xi {c}^\dagger c \left( { \frac{ b^\dagger - a^\dagger }{2} } \right) | + | \exp \left[ { i\xi {c}^\dagger c \left( { \frac{ b^\dagger - a^\dagger }{\sqrt{2} }} \right) |
| − | \left( { \frac{ b - a }{2} } \right) | + | \left( { \frac{ b - a }{\sqrt{ 2} }} \right) } \right] |
\\ | \\ | ||
&=& | &=& | ||
| Line 364: | Line 384: | ||
\, e^{i\frac{\xi}{2} {a}^\dagger a \, {c}^\dagger c } | \, e^{i\frac{\xi}{2} {a}^\dagger a \, {c}^\dagger c } | ||
\, e^{i\frac{\xi}{2} {b}^\dagger b \, {c}^\dagger c } | \, e^{i\frac{\xi}{2} {b}^\dagger b \, {c}^\dagger c } | ||
| + | \,. | ||
\,. | \,. | ||
\end{array}</math> | \end{array}</math> | ||
| Line 380: | Line 401: | ||
controlled-beamsplitter operator, where the rotation angle is <math>\xi | controlled-beamsplitter operator, where the rotation angle is <math>\xi | ||
{c}^\dagger c </math>. | {c}^\dagger c </math>. | ||
| + | |||
| + | ==== Squeezing with nonlinear interferometer ==== | ||
| + | |||
How does this nonlinear interferometer produce non-classical behavior? | How does this nonlinear interferometer produce non-classical behavior? | ||
Well, one thing it can be used for is to create a state very much like | Well, one thing it can be used for is to create a state very much like | ||
| Line 385: | Line 409: | ||
photons. Consider this setup, with two dual-rail qubits, and one Kerr | photons. Consider this setup, with two dual-rail qubits, and one Kerr | ||
medium: | medium: | ||
| − | ::[[Image:chapter2-quantum-light-part-3-kerr-mzi3.png|thumb| | + | ::[[Image:chapter2-quantum-light-part-3-kerr-mzi3.png|thumb|500px|none|]] |
| − | + | ||
This has two Mach-Zehnder interferometers coupled with a Kerr medium, | This has two Mach-Zehnder interferometers coupled with a Kerr medium, | ||
which we shall take to have <math>\chi L = \pi</math>. If the input state is | which we shall take to have <math>\chi L = \pi</math>. If the input state is | ||
| Line 407: | Line 431: | ||
<math>b</math>, is | <math>b</math>, is | ||
:<math>\begin{array}{rcl} | :<math>\begin{array}{rcl} | ||
| − | |\phi_3 \rangle &=& \frac{|1001 \rangle | + | |\phi_3 \rangle &=& \frac{|1001 \rangle +|0110{\rangle}}{\sqrt{2}} |
\,. | \,. | ||
\end{array}</math> | \end{array}</math> | ||
| + | |||
Compare this with the two-mode infinitely squeezed state <math>\sum_n f(n) | Compare this with the two-mode infinitely squeezed state <math>\sum_n f(n) | ||
| − | |n \rangle _1 |n \rangle _2</math> which we used | + | |n \rangle _1 |n \rangle _2</math> which we used [[Non-classical_states_of_light#Teleportation_of_light|in the discussion of teleportation]]. This state has exactly the same feature that when mode <math>a</math> has a single |
| − | state has exactly the same feature that when mode <math>a</math> has a single | ||
photon, mode <math>d</math> does also, and vice versa. The same is true also for | photon, mode <math>d</math> does also, and vice versa. The same is true also for | ||
modes <math>b</math> and <math>c</math>. This state has an extra spatial correlation that | modes <math>b</math> and <math>c</math>. This state has an extra spatial correlation that | ||
the two-mode infinitely squeezed state did not. But is is not hard to | the two-mode infinitely squeezed state did not. But is is not hard to | ||
| − | imagine that they have similar properties. | + | imagine that they have similar properties. In the section on [[Entangled Photons]] we |
| − | + | show that both are entangled quantum states, which have | |
correlations beyond what is possible with classical states. | correlations beyond what is possible with classical states. | ||
| + | |||
=== Deutsch-Jozsa algorithm === | === Deutsch-Jozsa algorithm === | ||
Nonlinear Mach-Zender interferometers are also useful for implementing | Nonlinear Mach-Zender interferometers are also useful for implementing | ||
| Line 424: | Line 449: | ||
of these is known as the Deutsch-Jozsa algorithm, which solves the | of these is known as the Deutsch-Jozsa algorithm, which solves the | ||
following problem. | following problem. | ||
| + | |||
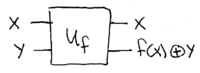
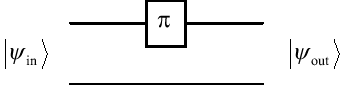
Suppose you are given the following box, which accepts two inputs <math>x</math> | Suppose you are given the following box, which accepts two inputs <math>x</math> | ||
and <math>y</math>, and produces two outputs, <math>x</math> and <math>y\oplus f(x)</math>, where | and <math>y</math>, and produces two outputs, <math>x</math> and <math>y\oplus f(x)</math>, where | ||
<math>\oplus</math> denotes addition modulo two: | <math>\oplus</math> denotes addition modulo two: | ||
::[[Image:chapter2-quantum-light-part-3-djfun.png|thumb|200px|none|]] | ::[[Image:chapter2-quantum-light-part-3-djfun.png|thumb|200px|none|]] | ||
| − | + | ||
Each signal is a single bit, and the box is promised to implement one | Each signal is a single bit, and the box is promised to implement one | ||
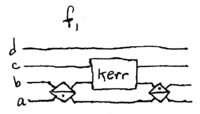
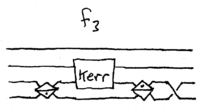
of four functions, computing either <math>f_0</math>, <math>f_1</math>, <math>f_2</math>, or <math>f_3</math>: | of four functions, computing either <math>f_0</math>, <math>f_1</math>, <math>f_2</math>, or <math>f_3</math>: | ||
| Line 448: | Line 474: | ||
odd functions. How many queries to the box must you perform to | odd functions. How many queries to the box must you perform to | ||
determine whether it is implementing an even or odd function? | determine whether it is implementing an even or odd function? | ||
| + | |||
If <math>0</math> and <math>1</math> are the only two values you can input for <math>x</math> and <math>y</math>, | If <math>0</math> and <math>1</math> are the only two values you can input for <math>x</math> and <math>y</math>, | ||
then at least two queries to the box are needed to answer this | then at least two queries to the box are needed to answer this | ||
| Line 479: | Line 506: | ||
odd is given by <math>f(0)\oplus f(1)</math>, and this expression clearly needs | odd is given by <math>f(0)\oplus f(1)</math>, and this expression clearly needs | ||
two evaluations of <math>f</math> to be computed, in general. | two evaluations of <math>f</math> to be computed, in general. | ||
| + | |||
If quantum superpositions are allowed as inputs, but the outputs are | If quantum superpositions are allowed as inputs, but the outputs are | ||
simply measured in the usual "computational" basis, then the problem | simply measured in the usual "computational" basis, then the problem | ||
still takes two queries to be solved. | still takes two queries to be solved. | ||
| + | |||
However, if quantum superpositions are allowed as inputs, and outputs | However, if quantum superpositions are allowed as inputs, and outputs | ||
can also be intefered, then only one query is needed. This is | can also be intefered, then only one query is needed. This is | ||
| Line 492: | Line 521: | ||
what is inside the <math>U_f</math> box for the four possible functions. These | what is inside the <math>U_f</math> box for the four possible functions. These | ||
are | are | ||
| − | + | {| border=1 | |
| − | + | |[[Image:chapter2-quantum-light-part-3-djinside-f0.png|thumb|200px|none|]] | |
| − | + | |[[Image:chapter2-quantum-light-part-3-djinside-f2.png|thumb|200px|none|]] | |
| − | + | |- | |
| + | |[[Image:chapter2-quantum-light-part-3-djinside-f1.png|thumb|200px|none|]] | ||
| + | |[[Image:chapter2-quantum-light-part-3-djinside-f3.png|thumb|200px|none|]] | ||
| + | |} | ||
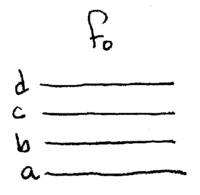
Note how <math>f_0</math> is trivial, since <math>f_0(x)\oplus y = y</math>; also | Note how <math>f_0</math> is trivial, since <math>f_0(x)\oplus y = y</math>; also | ||
straighforward is <math>f_2(x)\oplus y = 1\oplus y</math>, since this is just an | straighforward is <math>f_2(x)\oplus y = 1\oplus y</math>, since this is just an | ||
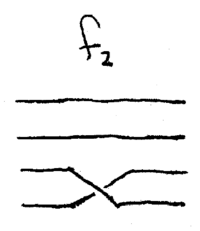
| Line 505: | Line 537: | ||
swapped if mode <math>c</math> has a photon, or left alone if mode <math>c</math> has no | swapped if mode <math>c</math> has a photon, or left alone if mode <math>c</math> has no | ||
photon. | photon. | ||
| + | |||
Inserting these into the algorithm, we find that if the input state is | Inserting these into the algorithm, we find that if the input state is | ||
<math>|1010{\rangle}</math> (designating modes as <math>|dcba{\rangle}</math>), the outputs are | <math>|1010{\rangle}</math> (designating modes as <math>|dcba{\rangle}</math>), the outputs are | ||
| Line 529: | Line 562: | ||
When the function is <math>f_0</math> or <math>f_2</math>, the <math>ba</math> modes completely | When the function is <math>f_0</math> or <math>f_2</math>, the <math>ba</math> modes completely | ||
decouple from the <math>cd</math> modes, so the output is trivially obtained. | decouple from the <math>cd</math> modes, so the output is trivially obtained. | ||
| + | |||
Thus, for those two cases, modes <math>dc</math> end up in <math>|10{\rangle}</math>, so a photon | Thus, for those two cases, modes <math>dc</math> end up in <math>|10{\rangle}</math>, so a photon | ||
is found in mode <math>d</math>. When the function is <math>f_1</math>, then the two | is found in mode <math>d</math>. When the function is <math>f_1</math>, then the two | ||
Latest revision as of 18:46, 31 January 2017
A single photon, mathematically represented by the number eigenstate , physically describes the electromagnetic field corresponding to the lowest nonzero energy eigenstate of a single mode cavity. is the vacuuum state. A great deal of physics can be understood by considering what happens to just the and states, through a variety of optical components. This section uses such an approach to explore three of the most basic components -- two linear components: phase shifters, beam splitters, and one nonlinear component: Kerr cross-phase modulation. These are the building blocks of linear and non-linear interferometers; their physical behavior provides helpful intuition for quantum behavior.
Contents
Beamsplitters and phase shifters
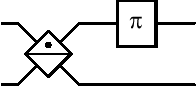
The number state evolves through propagation in free space to become after time . In a medium with a different index of refraction, however, light propagates at a different velocity, giving, for example, . Such a phase difference is only physically meaningful, however, when compared with a reference. Let us therefore introduce a pair of modes, each with either or photons, depicted as two lines, and using a box to indicate a segment through which one mode propagates at a different velocity. For example:
depicts two modes, in which the top photon has its phase shifted by relative to the bottom one. It is clear that any relative phase shift can be imparted between the two modes, by an appropriate experimental setup; experimentally, this can be accomplished with different thicknesses of glass, or by lengthening one path versus the other.
Two modes of light can be mixed by a beamsplitter (as we have previously seen). A beamsplitter acts with Hamiltonian
on the two modes, with corresponding operators and . Transformation of light through this beamsplitter is given by , where is the angle of the beamsplitter, giving the unitary operation
We may depict this as:
Note the use of a to distinguish the ports; this is needed because we have adopted a phase convention for the beamsplitter which obviates the need to keep track of an extra factor of . In the Heisenberg picture, transforms and as
This can be verified using the the Baker--Campbell--Hausdorf formula,
where is a complex number, , , and are operators, and is defined recursively as the sequence of commutators , , , , , .
Since it follows from and that and , for , we obtain for the expansion of the series coefficients , , , , which in general are
From this, our desired result follows straightforwardly:
The transform is trivially found by swapping and in the above solution.
Beamsplitters with single photon inputs
How does act on a single photon input? On input , letting the modes be on the right, and on the left, we get
Similarly, . This indicates that corresponds to a beamsplitter. Note that does not destroy any photons; it can only move them between the two modes. Mathamatically, this arises from the fact that it commutes with the total photon number operator, .
If both input modes contain photons, the output state does not have as simple a form as above. In particular, we find that
so it is possible for the output to be found to have both photons in one mode. Since we'd like to avoid such cases in this section, let us restrict our attention here to the case when inputs to beampsplitters have a total of one photon at most.
Dual-rail photon states and two-level systems
Omitting the vacuum state , the two-mode state space we shall consider thus has a basis state spanned by , and . We call this the dual-rail photon state space (this Hilbert space behaves much like a a two-level system). Note that an arbitrary state in this space as , where and are complex numbers satisfying .
From Bloch's Theorem, it follows that any dual-rail photon state can be generated using phase shifters and beamsplitters. Specifically, if we write as a two-component vector, then the action of is
Similarly, the action of a phase shifter of phase is
where the overall phase is irrelevant and can be dropped in the following.
Arbitrary single qubit operations with phase shifters and beam splitters
Employing Pauli matrices
Let us define the Pauli matrices as
Phase shifter and Beamsplitter operators
In terms of these, we find that the phase shifter and beamsplitter operators may be expressed as
Rotations of a two-level quantum system
These are rotations of a two-level system, about the axes and , by angles and , respectively. The standard rotation operator definitions are
Bloch's Theorem
In terms of these, Bloch's Theorem states the following:
| Theorem: (- decomposition of rotations)
Suppose is a unitary operation on a two-dimensional Hilbert space. Then there exist real numbers and such that |
Proof:~ Since is unitary, the rows and columns of are orthonormal, from which it follows that there exist real numbers ,and such that
Equation (\ref{eqtn:alg:qubit_decomp}) now follows immediately from the definition of the rotation matrices and matrix multiplication.
What we have just done, expressed in modern language, is to introduce an optical quantum bit, a "qubit," and showed that arbitrary single qubit operations ("gates") can be performed using phase-shifters and beamsplitters.
For example, one widely useful single-qubit transform is the Hadamard gate,
This operation can be performed by doing:
where the beamsplitter has . From inspection, it is easy to verify that it transforms and up to an overall phase, as desired. Up to a phase shift, a beamsplitter can thus be thought of as being a Hadamarad gate, and vice-versa.
Mach-Zehnder interferometer
The reason we have introduced the dual-rail photon representation of a qubit is because this will allow us to clarify the universality of certain quantum optical ideas, namely interference and interferometers, which will be ubiquitous through our treatment of atoms and quantum information.
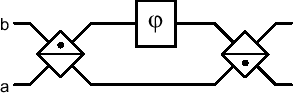
Let us begin by developing a model for the Mach-Zehnder interferometer, which is constructed from two beamsplitters. Recall that two beamsplitters and , configured as
naturally leave the output identical to the input, as . If a phase shifter is placed inbetween two beamsplitters,
then the input is transformed by
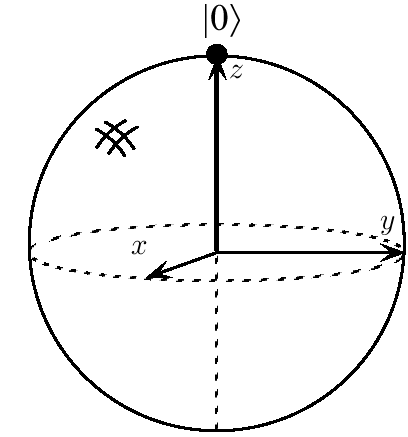
It is convenient to visualize this sequence of three rotations on the Bloch sphere:
The first rotates into , and into . The system is then rotated around by angle . Then the last rotates back to . The overall sequence is thus a rotation by about :
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} {B}^\dagger (\pi/4) P(\phi) B(\pi/4) &= & R_x(-\phi) \,. \end{array}}
If the input is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |01{\rangle}} , then the output will thus be Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \cos(\phi/2)|01{\rangle}-i\sin(\phi/2)|10{\rangle}} , so the photon is found in mode with probability Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \cos^2(\phi/2)} , and in mode Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} with probability Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \sin^2(\phi/2)} . This is exactly what a classical interferometer should do. Two important limits are that when (the interferometer is "balanced"), the input is unchanged, and when Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \phi=\pi} , the two modes are swapped.
Nonlinear Mach-Zehnder interferometer
The two components we have studied so far, phase shifters and beamsplitters, are linear optics elements. Such elements have an electric polarization which is linear with the applied electric field, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{P} = \epsilon_0 \chi \vec{E}} . Nonlinear optical elements, have Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{P} = \epsilon_0 (\chi^{(1)} \vec{E} + \chi^{(2)} \vec{E}^2 + \chi^{(3)} \vec{E}^3 + \cdots)} . Previously, we have seen that an optical parametric oscillator (with ) can be used for creating quantum states such as squeezed light. What do nonlinear optical elements do to single photons?
Optical Kerr media
Consider a material with Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \chi\sim \chi^{(3)}\neq 0} , which we may model as having the Hamiltonian
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H_{xpm} = -\chi a^\dagger a b^\dagger b \,, }
where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} and describe two modes propagating through the medium. For a crystal of length Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L} we obtain the unitary transform
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle K = e^{i \chi L a^\dagger a b^\dagger b} \,. }
Here, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \chi} parametrizes the third order nonlinear susceptibility coefficient. We will refer to as the Kerr cross-phase modulation Hamiltonian, and the nonlinear crystal as being a Kerr medium.
Interferometer with Kerr medium inside
Interesting non-classical behavior can be obtained using interferometers constructed with Kerr media used as nonlinear phase shifters. For single photon states, we find that
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} K |00 \rangle &=& |00{\rangle} \\ K |01 \rangle &=& |01{\rangle} \\ K |10 \rangle &=& |10{\rangle} \\ K |11 \rangle &=& e^{i\chi L} |11{\rangle} \,. \end{array}}
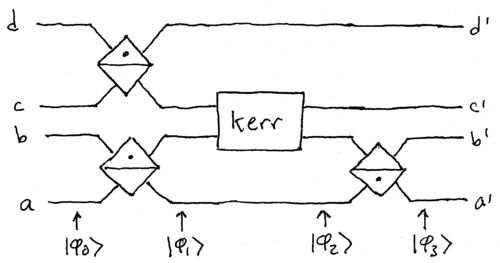
Let us take Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \chi L = \pi} , such that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle K|11 \rangle = -|11{\rangle}} . Suppose we now place the Kerr medium inside a Mach-Zehnder interferometer in this manner:
\noindent Intuitively, we expect that when no photons are input into , then the Mach-Zehnder interferometer is balanced, leading to Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a'=a} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b' = b} . But when a photon is input into Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle c} , if the cross-phase modulation due to Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle K} is sufficiently large (), then the inputs are swapprd, producing Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a'=b} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b'=a} .
Mathematically, we may write the transform performed by this nonlinear Mach-Zehnder interferometer as the unitary transform Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle U = {B}^\dagger K B} , where is a 50/50 beamsplitter, is the Kerr cross phase modulation operator , and is the product of the coupling constant and the interaction distance. The transform simplifies to give
The first and third exponentials are constant phase shifts, and the last two phase shifts come from cross phase modulation. All those effects are not fundamental, and can be compensated for. The interesting term is the second exponential, which we define as
For Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \xi=\pi} , when no photons are input at Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle c} , then Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a' = a} and , but when a single photon is input at Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle c} , then Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a' = b} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b' = a} , as we expected. We may also interpret as being like a controlled-beamsplitter operator, where the rotation angle is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \xi {c}^\dagger c } .
Squeezing with nonlinear interferometer
How does this nonlinear interferometer produce non-classical behavior? Well, one thing it can be used for is to create a state very much like two-mode squeezed light, as we now show in the limit of single photons. Consider this setup, with two dual-rail qubits, and one Kerr medium:
This has two Mach-Zehnder interferometers coupled with a Kerr medium, which we shall take to have Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \chi L = \pi} . If the input state is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\phi_0 \rangle = |0101{\rangle}} , using mode labeling , then the state after the first two 50/50 beamsplitters is
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} |\phi_1 \rangle &=& (|01{\rangle}+|10 \rangle )(|01{\rangle}+|10 \rangle ) \\ &=& |0101 \rangle + |0110 \rangle +|1001 \rangle + |1010{\rangle} \,, \end{array}}
up to a normalization factor which we shall suppress for clarity. The Kerr medium takes Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle K|11{\rangle}\rightarrow -|11{\rangle}} and leaves all other basis states unchanged. Thus,
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} |\phi_2 \rangle &=& |0101 \rangle - |0110 \rangle +|1001 \rangle + |1010{\rangle} \,. \end{array}}
Finally, the output state, given by applying to modes Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} , is
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} |\phi_3 \rangle &=& \frac{|1001 \rangle +|0110{\rangle}}{\sqrt{2}} \,. \end{array}}
Compare this with the two-mode infinitely squeezed state which we used in the discussion of teleportation. This state has exactly the same feature that when mode Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} has a single photon, mode Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d} does also, and vice versa. The same is true also for modes Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} and . This state has an extra spatial correlation that the two-mode infinitely squeezed state did not. But is is not hard to imagine that they have similar properties. In the section on Entangled Photons we show that both are entangled quantum states, which have correlations beyond what is possible with classical states.
Deutsch-Jozsa algorithm
Nonlinear Mach-Zender interferometers are also useful for implementing and understanding simple quantum algorithms. One of the most elementary of these is known as the Deutsch-Jozsa algorithm, which solves the following problem.
Suppose you are given the following box, which accepts two inputs Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y} , and produces two outputs, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} and , where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \oplus} denotes addition modulo two:
Each signal is a single bit, and the box is promised to implement one of four functions, computing either Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_0} , Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_1} , , or Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_3} :
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_0(x)} Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_2(x)} Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_3(x)} 0 0 0 1 1 1 0 1 1 0
Call Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_0} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_2} the even functions, and and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_3} the odd functions. How many queries to the box must you perform to determine whether it is implementing an even or odd function?
If Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 0} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1} are the only two values you can input for and , then at least two queries to the box are needed to answer this question. This can be seen by direct examination of the full input-output table:
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_2(x)\oplus y} Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_3(x)\oplus y} 0 0 0 0 1 1 0 1 0 1 1 0 1 0 1 1 0 0 1 1 1 0 0 1
and by observing that (1) changing Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y} gives no additional information about whether implements an even or odd function, and (2) for any single input value of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} , there are both even and odd functions which give the same output. Indeed, whether the function is even or odd is given by Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f(0)\oplus f(1)} , and this expression clearly needs two evaluations of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} to be computed, in general.
If quantum superpositions are allowed as inputs, but the outputs are simply measured in the usual "computational" basis, then the problem still takes two queries to be solved.
However, if quantum superpositions are allowed as inputs, and outputs can also be intefered, then only one query is needed. This is done using the following procedure. Let us use dual-rail photon qubits, and choose to represent Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 0} , and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |10{\rangle}} to represent Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1} . The optical setup implementing the quantum algorithm to solve the Deutsch-Jozsa problem is:
The key to understanding how this works is to explicitly write down what is inside the box for the four possible functions. These are
Note how Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_0} is trivial, since Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_0(x)\oplus y = y} ; also straighforward is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_2(x)\oplus y = 1\oplus y} , since this is just an inversion of , that is accomplished by swapping modes Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} . The two odd functions involve an interaction between modes Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ba} and , because Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_1(x)\oplus y = x\oplus y} , and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_3(x)\oplus y = 1\oplus x\oplus y} . These two are implemented with nonlinear Mach-Zehnder interferometers, which cause modes Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} and to be swapped if mode Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle c} has a photon, or left alone if mode Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle c} has no photon.
Inserting these into the algorithm, we find that if the input state is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |1010{\rangle}} (designating modes as ), the outputs are
function output state Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_0} Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |10 \rangle ( |01{\rangle}+|10 \rangle ) } Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_1} Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_2} Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |10 \rangle ( |10{\rangle}-|01 \rangle ) } Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_3}
When the function is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_0} or Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f_2} , the Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ba} modes completely decouple from the modes, so the output is trivially obtained.
Thus, for those two cases, modes Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle dc} end up in Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |10{\rangle}} , so a photon is found in mode Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d} . When the function is , then the two initial beamsplitters on Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ba} cancel, leaving a photon in mode . This photon then causes the nonlinear Mach-Zehnder interferometer in modes to flip the photons between those modes. A similar thing happens for function , leaving modes in state , so a photon is found in mode Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle c} . The measurement of whether a photon ends up in mode Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle c} or in mode Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d} thus determines whether the function is even or odd.
The main insight given by this example, which generalizes to more complex quantum algorithms, is that phases and interference are central to their operation. Another important insight is that quantum algorithms are somewhat of like a kind of spectroscopy: just as the standard Mach-Zehnder interferometer may be used to measure the index of refraction of an unknown crystal, nonlinear, coupled Mach-Zehnder interferometers can be used to measure periods of certain functions. Indeed, it is through period measurement that Shor's quantum factoring algorithm works.
Another important insight gained by this example is that quantum algorithms are likely complex and difficult to implement, if they require a multitude of coupled interferometers. This is because well balanced, stable interferometers are experimentally challenging to realize. Nonlinear optical Kerr media that have no loss, and can impart a cross phase modulation between single photons, are also rather exotic.
Finally, it is worthwhile considering exactly what we used which was quantum-mechanical in implementing the Deutsch-Jozsa algorithm. Would this implementation have worked with coherent states, instead of single photons?














![{\displaystyle B=\exp \left[{\theta \left({a^{\dagger }b-ab^{\dagger }}\right)}\right]\,.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/cde964c0c37431a84260835508da295a53bd5290)
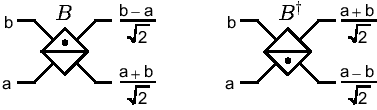









![{\displaystyle C_{1}=[G,C_{0}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f83df0933d6a3c3706b351c36fb81538515a85b9)
![{\displaystyle C_{2}=[G,C_{1}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6d6157b6fd985b0ff55033671895f6dccd4a5d81)
![{\displaystyle C_{3}=[G,C_{2}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/cb7b38ae279f6651995a6a493bacfe81b34c5880)

![{\displaystyle C_{n}=[G,C_{n-1}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b9d20f4ef4def8f2300f808179588f8bfdbf739f)
![{\displaystyle [a,a^{\dagger }]=1}](https://wikimedia.org/api/rest_v1/media/math/render/svg/671da8b1eb59fe1c04e601cc7963a6511d88c74e)
![{\displaystyle [b,b^{\dagger }]=1}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2ffc8deb8c5daa77fae70826f05172239777e632)
![{\displaystyle [G,a]=b}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c839dfe2562400194bd40409990af8c8105a447f)
![{\displaystyle [G,b]=-a}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3e66ece5d36dd8598163277d2bf53cc526ae88e9)



![{\displaystyle C_{1}=[G,a]=b}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a3abeae2e5e7f5457bbed75f80bbf6ed33383864)
![{\displaystyle C_{2}=[G,C_{1}]=-a}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7ad248c8f33c48531a9cc5c09f1b481560f63e05)
![{\displaystyle C_{3}=[G,C_{2}]=-[G,C_{0}]=-b}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b328c9d224959a15fe187a32c21e8acd51a7ffd0)

















![{\displaystyle B(\theta )\left[{\begin{array}{c}{\alpha }\\{\beta }\end{array}}\right]=\left[{\begin{array}{cc}{\cos \theta }&{-\sin \theta }\\{\sin \theta }&{\cos \theta }\end{array}}\right]\left[{\begin{array}{c}{\alpha }\\{\beta }\end{array}}\right]\,.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/bbd7073849d13faa33b118bd924076ccde2ef741)


![{\displaystyle P(\phi )\left[{\begin{array}{c}{\alpha }\\{\beta }\end{array}}\right]=e^{-i\theta /2}\left[{\begin{array}{cc}{e^{i\theta /2}}&{0}\\{0}&{e^{-i\theta /2}}\end{array}}\right]\left[{\begin{array}{c}{\alpha }\\{\beta }\end{array}}\right]\,,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/23b28130466753154f3e728eff05df125e6f33ac)

![{\displaystyle {\begin{array}{rcl}X&=&\left[{\begin{array}{cc}{0}&{1}\\{1}&{0}\end{array}}\right]\\Y&=&\left[{\begin{array}{cc}{0}&{-i}\\{i}&{0}\end{array}}\right]\\Z&=&\left[{\begin{array}{cc}{1}&{0}\\{0}&{-1}\end{array}}\right]\,.\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2f4c4dc132ee998872269c6b90b2cac55933dcdc)
![{\displaystyle {\begin{array}{rcl}B(\theta )&=&\exp \left[{i\theta Y}\right]\\P(\phi )&=&\exp \left[{i\phi Z/2}\right]\,.\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8ed1c798b58ec2107ccfe2fb5b482d0433eb05ea)



![{\displaystyle {\begin{array}{rcl}R_{x}(\theta )&\equiv &e^{-i\theta X/2}=\cos {\frac {\theta }{2}}I-i\sin {\frac {\theta }{2}}X=\left[{\begin{array}{cc}\cos {\frac {\theta }{2}}&-i\sin {\frac {\theta }{2}}\\-i\sin {\frac {\theta }{2}}&\cos {\frac {\theta }{2}}\end{array}}\right]\\R_{y}(\theta )&\equiv &e^{-i\theta Y/2}=\cos {\frac {\theta }{2}}I-i\sin {\frac {\theta }{2}}Y=\left[{\begin{array}{cc}\cos {\frac {\theta }{2}}&-\sin {\frac {\theta }{2}}\\\sin {\frac {\theta }{2}}&\cos {\frac {\theta }{2}}\end{array}}\right]\\R_{z}(\theta )&\equiv &e^{-i\theta Z/2}=\cos {\frac {\theta }{2}}I-i\sin {\frac {\theta }{2}}Z=\left[{\begin{array}{cc}e^{-i\theta /2}&0\\0&e^{i\theta /2}\end{array}}\right]\,.\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/87225207af366d9d68d1998719ba6d411b5dace3)






![{\displaystyle {\begin{array}{rcl}U=\left[{\begin{array}{cc}e^{i(\alpha -\beta /2-\delta /2)}\cos {\frac {\gamma }{2}}&-e^{i(\alpha -\beta /2+\delta /2)}\sin {\frac {\gamma }{2}}\\e^{i(\alpha +\beta /2-\delta /2)}\sin {\frac {\gamma }{2}}&e^{i(\alpha +\beta /2+\delta /2)}\cos {\frac {\gamma }{2}}\end{array}}\right].\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2cdffa723507b330398ac45f72d11c6d7247cbd5)
![{\displaystyle {\begin{array}{rcl}H={\frac {1}{\sqrt {2}}}\left[{\begin{array}{cc}{1}&{1}\\{1}&{-1}\end{array}}\right]\,.\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/66f26d09a9191a1aa422af968868ed8ff0ea03c7)






![{\displaystyle {\begin{array}{rcl}{B}^{\dagger }(\pi /4)P(\phi )B(\pi /4)&=&\exp \left[{-i\pi Y/4}\right]\exp \left[{i\phi Z/2}\right]\exp \left[{i\pi Y/4}\right]\\&=&R_{y}(\pi /2)R_{z}(-\phi )R_{y}(-\pi /2)\,.\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7d6da722c294cc51f364863de15b94d011bd159f)













![{\displaystyle {\begin{array}{rcl}U&=&\exp \left[{i\xi {c}^{\dagger }c\left({\frac {b^{\dagger }-a^{\dagger }}{\sqrt {2}}}\right)\left({\frac {b-a}{\sqrt {2}}}\right)}\right]\\&=&e^{i{\frac {\pi }{2}}{b}^{\dagger }b}\,e^{{\frac {\xi }{2}}{c}^{\dagger }c(a^{\dagger }b-b^{\dagger }a)}\,e^{-i{\frac {\pi }{2}}{b}^{\dagger }b}\,e^{i{\frac {\xi }{2}}{a}^{\dagger }a\,{c}^{\dagger }c}\,e^{i{\frac {\xi }{2}}{b}^{\dagger }b\,{c}^{\dagger }c}\,.\,.\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/87d22ba1cdcfbbccfd04e39c3597547b5ec76326)
![{\displaystyle F(\xi )=\exp \left[{{\frac {\xi }{2}}{c}^{\dagger }c\,(a^{\dagger }b-b^{\dagger }a)}\right]\,.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b4437ff0a1688cced07348cbd6429a496328cb18)