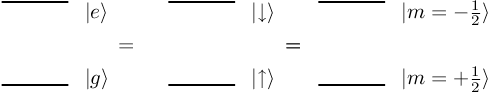Equation of Motion for the Expectation Value
For the system we have been considering, the Hamiltonian is

Recalling the Heisenberg equation of motion for any operator
 is
is
![{\displaystyle {\frac {d}{dt}}{\hat {O}}={\frac {i}{\hbar }}\left[{\hat {H}},{\hat {O}}\right]+{\frac {\partial {\hat {O}}}{\partial t}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ae5069cad777556e25ef2674f2ed7f1fbdc641a0)
where the last term refers to operators with an explicit time dependence, we
have in this instance
![{\displaystyle {\frac {d}{dt}}{\hat {\mu }}_{k}=\gamma {\frac {d}{dt}}{\hat {L}}_{k}={\frac {i\gamma }{\hbar }}\left[{\hat {H}},{\hat {L}}_{k}\right]=-{\frac {i\gamma ^{2}}{\hbar }}B_{0}\left[{\hat {L}}_{z},{\hat {L}}_{k}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/31c5220d2321caf92920986550a99c6ba69fb523)
Using ![{\displaystyle \left[{\hat {L}}_{k},{\hat {L}}_{l}\right]=i\hbar \epsilon _{klm}{\hat {L}}_{m}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5b0dce00bc29fe901e80ab62151d3d46ed0adb58) with the
Levi-Civita symbol
with the
Levi-Civita symbol  , we have
, we have

or in short

These are just like the classical equations of motion
\ref{eq:classical_precession_in_static_field}, but here they describe the
precession of the operator for the magnetic moment  or for the
angular momentum
or for the
angular momentum  about the magnetic field at the (Larmor)
angular frequency
about the magnetic field at the (Larmor)
angular frequency  .
.
Note that:
- Just as in the classical model, these operator equations are exact; we have not neglected any higher order terms.
- Since the equations of motion hold for the operator, they must hold for the expectation value

- We have not made use of any special relations for a spin-
 system, but just the general commutation relation for angular momentum. Therefore the result, precession about the magnetic field at the Larmor frequency, remains true for any value of angular momentum
system, but just the general commutation relation for angular momentum. Therefore the result, precession about the magnetic field at the Larmor frequency, remains true for any value of angular momentum  .
.
- A spin-
 system has two energy levels, and the two-level problem with coupling between two levels can be mapped onto the problem for a spin in a magnetic field, for which we have developed a good classical intuition.
system has two energy levels, and the two-level problem with coupling between two levels can be mapped onto the problem for a spin in a magnetic field, for which we have developed a good classical intuition.
- If coupling between two or more angular momenta or spins within an atom results in an angular momentum
 , the time evolution of this angular momentum in an external field is governed by the same physics as for the two-level system. This is true as long as the applied magnetic field is not large enough to break the coupling between the angular momenta; a situation known as the Zeeman regime. Note that if the coupled angular momenta have different gyromagnetic ratios, the gyromagnetic ratio for the composite angular momentum is different from those of the constituents.
, the time evolution of this angular momentum in an external field is governed by the same physics as for the two-level system. This is true as long as the applied magnetic field is not large enough to break the coupling between the angular momenta; a situation known as the Zeeman regime. Note that if the coupled angular momenta have different gyromagnetic ratios, the gyromagnetic ratio for the composite angular momentum is different from those of the constituents.
- For large magnetic field the interaction of the individual constituents with the magnetic field dominates, and they precess separately about the magnetic field. This is the Paschen-Back regime.
- An even more interesting composite angular momentum arises when
 two-level atoms are coupled symmetrically to an external field. In this case we have an effective angular momentum
two-level atoms are coupled symmetrically to an external field. In this case we have an effective angular momentum  for the symmetric coupling:
for the symmetric coupling:
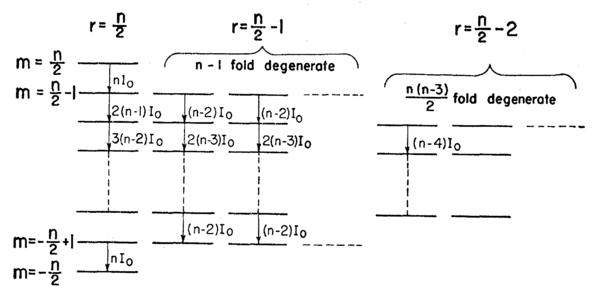
Level structure diagram for  two-level atoms in a basis of symmetric states \cite{Dicke1954}. The leftmost column corresponds to an effective spin-
two-level atoms in a basis of symmetric states \cite{Dicke1954}. The leftmost column corresponds to an effective spin- object. Other columns correspond to manifolds of symmetric states of the
object. Other columns correspond to manifolds of symmetric states of the  atoms with lower total effective angular momentum.
atoms with lower total effective angular momentum.
- Again the equation of motion for the composite angular momentum
 is a precession. This is the problem considered in Dicke's famous paper \cite{Dicke1954}, in which he shows that this collective precession can give rise to massively enhanced couplings to external fields ("superradiance") due to constructive interference between the individual atoms.
is a precession. This is the problem considered in Dicke's famous paper \cite{Dicke1954}, in which he shows that this collective precession can give rise to massively enhanced couplings to external fields ("superradiance") due to constructive interference between the individual atoms.
The Two-Level System: Spin-1/2
Let us now specialize to the two-level system and calculate the time evolution
of the occupation probabilities for the two levels.
{fig:two_level_spin_half} Equivalence of two-level system with spin- . Note that for
. Note that for
 the spin-up state (spin aligned with field) is the ground state. For an electron, with
the spin-up state (spin aligned with field) is the ground state. For an electron, with  , spin-up is the excited state. Be careful, as both conventions are used in the literature.
, spin-up is the excited state. Be careful, as both conventions are used in the literature.
We have that

where in the last equation we have used the fact that  . The signs
are chosen for a spin with
. The signs
are chosen for a spin with  , such as a proton (Figure
\ref{fig:two_level_spin_half}). For an electron, or any other spin with
, such as a proton (Figure
\ref{fig:two_level_spin_half}). For an electron, or any other spin with
 , the analysis would be the same but for the opposite sign of
, the analysis would be the same but for the opposite sign of  and the corresponding exchange of
and the corresponding exchange of  and
and  . If the system is
initially in the ground state,
. If the system is
initially in the ground state,  (or the spin along
(or the spin along
 ,
,  ), the expectation value obeys the classical
equation of motion \ref{eq:classical_rabi_flopping}:
), the expectation value obeys the classical
equation of motion \ref{eq:classical_rabi_flopping}:
- {eqn:rabi_transition_probability}

Equation \ref{eqn:rabi_transition_probability} is the probability to find system
in the excited state at time  if it was in ground state at time
if it was in ground state at time  .
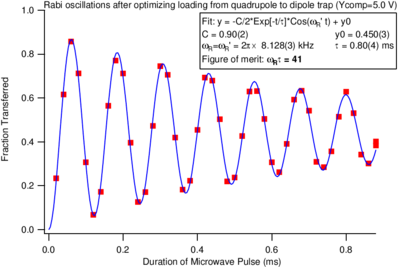
Figure \ref{fig:rabi_signal} shows a real-world example of such an oscillation.
.
Figure \ref{fig:rabi_signal} shows a real-world example of such an oscillation.
{fig:rabi_signal} Rabi oscillation signal taken in the Vuleti\'{c} lab shortly after this
topic was covered in lecture in 2008. The amplitude of the oscillations decays
with time due to spatial variations in the strength of the drive field (and
hence of the Rabi frequency), so that the different atoms drift out of phase
with each other.
Matrix form of Hamiltonian
With the matrix representation

we can write the Hamiltonian  associated with the static
field
associated with the static
field  as
as

where  is the Larmor frequency, and
is the Larmor frequency, and

is a Pauli spin matrix. The eigenstates are  ,
,  with
eigenenergies
with
eigenenergies  . A spin initially
along
. A spin initially
along  , corresponding to
, corresponding to

evolves in time as

which describes a precession with angluar frequency  .
The field
.
The field  , rotating at
, rotating at  in the
in the  plane corresponds to
plane corresponds to

where have used the Pauli spin matrices  ,
,  . The full
Hamiltonian is thus given by
. The full
Hamiltonian is thus given by
- {eq:dressed_atom_hamiltonian}

This is the famous "dressed atom" Hamiltonian in the so-called "rotating wave
approximation". However, note that in the treatment here there are no approximations; it is exact. Its eigenstates and eigenvalues provide a very elegant, very
intuitive solution to the two-state problem.
Solution of the Schrodinger Equation for Spin-1/2 in the Rotating Frame
Solution of the Schrodinger Equation for Spin-1/2 in the Interaction Representation
The interaction representation consists of expanding the state  in terms of the eigenstates
in terms of the eigenstates  ,
,  of the Hamiltonian
of the Hamiltonian  ,
including their known time dependence
,
including their known time dependence  due to
due to  .
That means we write here
.
That means we write here

Substituting this into the Schrodinger equation

then results in the equations of motion for the coefficients

Where we have used the matrix form of the Hamiltonian,
\ref{eq:dressed_atom_hamiltonian}. Introducing the detuning
 , we have
, we have

The explicit time dependence can be eliminated by the sustitution

As you will show (or have shown) in the problem set, this leads to solutions for
 given by
given by

with two constants that are determined by the initial conditions.
For  we find
we find

as already derived from the fact that the expectation value for
the magnetic moment obeys the classical equation.
"Rapid" Adiabiatic Passage (quantum treatment)
(in the works)
The quantum mechanical treatment yields a probability for non-adiabatic transition (probability for the
magnetic moment not following the magnetic field) given by

in agreement with the qualitative classical criterion derived above.


![{\displaystyle {\frac {d}{dt}}{\hat {O}}={\frac {i}{\hbar }}\left[{\hat {H}},{\hat {O}}\right]+{\frac {\partial {\hat {O}}}{\partial t}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ae5069cad777556e25ef2674f2ed7f1fbdc641a0)
![{\displaystyle {\frac {d}{dt}}{\hat {\mu }}_{k}=\gamma {\frac {d}{dt}}{\hat {L}}_{k}={\frac {i\gamma }{\hbar }}\left[{\hat {H}},{\hat {L}}_{k}\right]=-{\frac {i\gamma ^{2}}{\hbar }}B_{0}\left[{\hat {L}}_{z},{\hat {L}}_{k}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/31c5220d2321caf92920986550a99c6ba69fb523)
![{\displaystyle \left[{\hat {L}}_{k},{\hat {L}}_{l}\right]=i\hbar \epsilon _{klm}{\hat {L}}_{m}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5b0dce00bc29fe901e80ab62151d3d46ed0adb58)