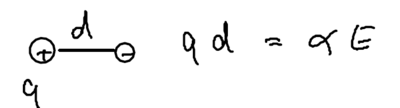Dipole forces within the dressed atom approach
In this section, we visit the physics of stimulated light forces, using the the dressed atom picture. Just to whet your appetite for the following - we will understand, below, why it is blue detuned light you choose for laser cooling at high intensities, rather than red detuned light. Also, recall that in the case of the stimulated force, fluctuations of the stimulated force cause heating. There is a special nature to the fluctuations of the stimulated force, due to the radiative cascade picture in the dressed atom approach. In switching from one dressed level to the next, the force switches sign! The atom thus get kicked back and forth. We will therefore see how these fluctuations of the stimulated force lead to momentum diffusion. The stimulated force has application mostly to dipole traps and optical lattices. Understanding the cooling and heating due to the stimulated force really broadens your understanding of what light forces are about. It is essentially different from the spontaneous light force, involved in the optical molasses.
The insights from this section cover dipole traps, blue molasses, heating from dipole forces, the electromagnetic origin of the dipole forces, and energy conservation.
Contents
- 1 Review of the dressed atom picture
- 2 Mean dipole force at zero velocity
- 3 Mean dipole force at non-zero velocity
- 4 Atomic momentum diffusion in the dressed atom picture
- 5 Atoms moving in a standing wave: Sisyphus cooling
- 6 Dressed atoms in a perturbative limit: cooling in a standing wave
- 7 Four physical pictures of the dipole force
- 8 Electric and magnetic components of the dipole force
- 9 Energy conservation and dipole forces
- 10 References
Review of the dressed atom picture
Let us begin with a reminder about three things we need from the dressed atom picture: energy levels, populations, and relaxation times.
Energies
Recall that there is a splitting between dressed states, giving the energies
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hbar\Omega (r) = \hbar\sqrt{\Omega_1^2(r) + \delta_L^2} \,. }
Populations
We also want to know the populations Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Pi_1} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Pi_2} of the dressed states and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2} ; these came from diagonalizing the coupling matrices and introducing rate equations, giving
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Pi_1^{st} = \frac{\sin^4\theta}{\cos^4\theta+\sin^4\theta} }
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Pi_2^{st} = \frac{\cos^4\theta}{\cos^4\theta+\sin^4\theta} }
where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \tan2\theta = -\Omega_1/\delta_L} .
Relaxation rates
Finally, recall that if we are not in steady state, there are relaxation rates involved which drive the system to the steady state. These rates are proportional to the spontaneous emission rate:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Gamma_{pop} = \Gamma(\cos^4\theta+\sin^4\theta) \,. }
Mean dipole force at zero velocity
Let us begin by considering what force is seen by an atom in a tightly focused laser beam, when the light is detuned to the red or to the blue of the atomic transition.
The dipole force
If you have a laser beam which is spatially focused, then the energy of the atom as a function of position is a function of where the atom is located relative to the beam:
Assume the light is intense, so that we may use the dressed atom picture. The splitting of the energies of the upper and lower dressed level leads to energies Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \pm\hbar\Omega(r)/2} , so the forces seen by the upper and lower levels are
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} F_1 &= -\frac{\hbar}{2}\nabla \Omega(r) \\F_2 &= +\frac{\hbar}{2}\nabla \Omega(r) \end{align}}
Note that in these expressions, the Rabi frequency is a function of space, because of the spatial dependence of the light intensity. The average force is thus
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} \langle F_{dip} \rangle &= F_1 \Pi_1^{st} + F_2 \Pi_2^{st} \\ &= -\frac{\hbar\delta_L}{2} \frac{\Omega_1^2}{\frac{\Omega_1^2}{2} +\delta_L^2}\vec{\alpha} \end{align}}
Note that this is only valid in several limits, imposed by the basic constraint that the dressed atom picture is valid (ie strong light intensity).
Dipole forces seen with blue and red detuned light
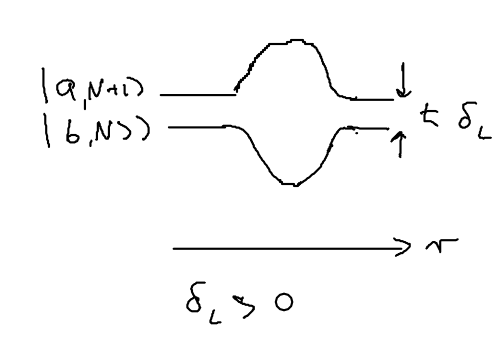
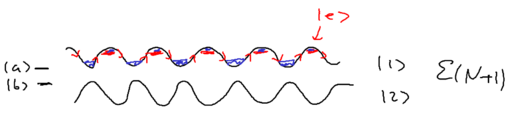
We may compare what happens when we have blue detuned light versus red detuned light. Consider two choices. What we couple with the light is the ground state with photons, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |a,N+1{\rangle}} , and the excited state, with photons, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |b,N{\rangle}} . Which of these two states have higher energy for positive (blue) detuning? The Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |a,N+1{\rangle}} state, because the photon has more energy than the atomic transition. Therefore, the proper labels on the energy levels when blue detuned are:
On the other hand, when red detuned, the energy levels should be labeled as:
Keep in mind, however, that spontaneous emission drives the atom towards its ground state. Eventually, therefore, in the blue detuned state the upper energy level eventually has more population, whereas when red detuned the lower energy level has more population.
The dipole force is repulsive for blue detuning, and the dipole force is attractive for red detuning.
What happens when we are on resonance, and the detuning is zero? In this case, the upper and lower states see equal force, so the average force is zero.
Mean dipole force at non-zero velocity
As a second application, let us discuss the mean dipole force seen by an atom which is not at rest, but is slowly moving. This will let us understand how blue detuned light cools an atom, in the dressed atom picture.
We have to go one step beyond what we did for the mean forces, because the derivations above stem from a picture of forces arising from a potential, and motion in a potential is conservative. Recall that the average force is
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \langle F_{dip} \rangle = F_1 \Pi_1^{st} + F_2 \Pi_2^{st} }
Now factor in the fact that the populations are not {\em instantaneously} in the steady state, but rather, there is a time lag of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1/\Gamma_{pop}} before the populations come into equilibrium.
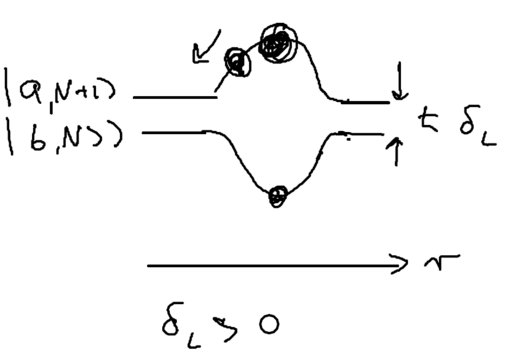
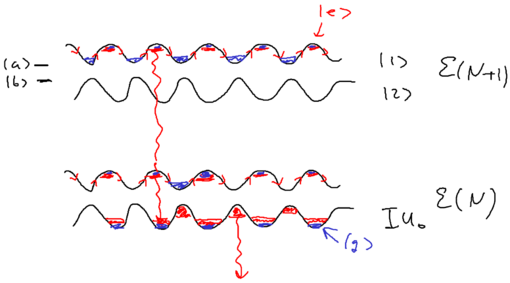
Consider the picture of an atom going through a laser beam; note that this could be a standing wave, as in an optical lattice. Let's figure out what the atom has to do as it traverses the beam. Originally, the atom is purely in the ground state, and at positive detuning the ground state is the upper energy level. To reach the top of the energy hump, there must be some admixture of the excited state added:
The size of the blobs in this picture reflect the population in the level. Assume the atom is moving towards the right. What is the extra force seen because the populations lag behind that which it should have in steady state, as the atom is moving? The more the population is in the upper level, the more force there is to the left. So if the actual population (dotted red lines) is larger than the steady state (solid lines) there is an extra force to the left.
As the atom leaves the middle of the beam, the populations continue to lag behind. But due to the population lag, the upper level population is a bit smaller than it would be in steady state. This causes the atoms to continue seeing a force to the left.
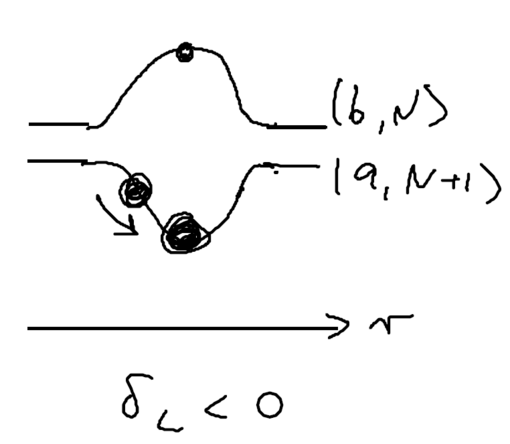
Now imaging that an atom rides a standing wave, going up and down. Because of the time lag in the population, the atoms now see a force in the opposite direction from its movement. Let us emphasize this point: the time lag leads to a hysteresis, which gives rise to dissipation.
More formally, the populations are the steady state values, but not at the present position of the atom, but rather, at a time given by time before:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Pi_i = \Pi_i^{st} (\vec{r}-\vec{v} \tau_{pop}) \,. }
Thus, the dipole force as a function of position and velocity is
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{F}_{dip}(\vec{r},v) = \vec{F}_{dip}^{st} - \frac{2\hbar\delta_L}{\Gamma} \left( \frac{\Omega_1^2(r)}{\Omega_1^2(r)+2\delta_L^2} \right) (\vec{\alpha}\cdot \vec{v}) \vec{\alpha} }
where is the force at Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle v=0} , and is the friction coefficient obtained.
Now suppose the light is a one-dimensional standing wave Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Omega_1(x) = 2\Omega_1 \cos kx} . Averaging over one cycle of the standing wave, we find that the force seen by the atom is
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \langle F_{dip} \rangle = -\alpha v \,, }
noting that averages to zero.
Some limits of this behavior are very useful. In the limit of large detuning Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \delta_L\gg \Omega_1} , in the standing wave case, we note that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{\alpha} \approx \vec{k}} , so the friction coefficient in this situation is approximately
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha \approx \frac{\hbar\delta_L}{\Gamma} \frac{\Omega_1^6}{\delta_L^6} k^2 \,. }
This will be derived later, when we strip the dressed atom picture to its bare bones and look at it for physical insight.
Atomic momentum diffusion in the dressed atom picture
We've understood that the dynamics of the radiative cascade leads to population lag, which leads to dissipation. The next thing we want to discuss is that there is also a heating mechanism, which is due to fluctuating forces. Let us see how this arises in the dressed atom picture, for an atom in a standing wave.
Momentum diffusion from force fluctuations
Recall that in the molasses the final temperature was given by Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha/D_p} , where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha} was the friction force describing how well we cool, and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D_p} was the momentum diffusion rate due to spontaneous emission. The momentum diffusion coefficient is
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2D_p = \frac{d}{dt} \left( \langle p^2 \rangle - \langle p{\rangle}^2 \right) \,. }
Note that such diffusion arises from fluctuating forces. Physically, the momentum is the time integral of all the forces which have been acting on the particle. We can write Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle p^2} as Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{p} \cdot \vec{p}} . Thus,
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} 2D_p &= 2 \langle \vec{p}\cdot \vec{F}(0) \rangle - {\langle}\vec{p} \rangle {\langle}\vec{F}(0){\rangle} \\ &= 2\int_{-\infty}^0 \left( {\langle}\vec{F}(t)\vec{F}(0){\rangle} - {\langle}\vec{F}(t){\rangle}{\langle}\vec{F}(0) \rangle \right) dt \,. \end{align}}
Thus, the momentum diffusion coefficient can be written as an integral over the force correlations:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} D_p &= \int_0^\infty \left( {\langle}\vec{F}(t)\vec{F}(0){\rangle} - {\langle}\vec{F}(0){\rangle}^2 \right) dt \end{align}}
When we discussed momentum diffusion from absorbed light, we had a clear picture of where randomness of momentum came from: the absorbed photons. In this case, we don't have such a clear picture in the absorbed momentum, but we have a clear picture of the forces experienced by the atom. It this thus useful to relate momentum diffusion to the randomness of forces experienced. We will then get a heating rate from
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle {\langle}\dot{E}_{\rm heat} \rangle = \frac{D_p}{M} }
and the final temperature will come from Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k_BT = \frac{D_p}{\alpha}} .
Momentum diffusion in the dressed atom picture
In the radiative cascade picture, as the atom cascades through the dressed levels, it experiences a force which is the same in magnitude, but reverses direction from left to right. Thus, we want to write down a statistical average over these fluctuating forces to get the momentum diffusion rate we want.
On resonance, the correlation time, the time range over which forces at time Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \tau} is related to the force at time , is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2/\Gamma} . Thus, the integral we have for the momentum diffusion coefficient should only go over this time period. It means we have that the momentum diffusion rate due to dipole forces is
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D_{dipole} \approx \frac{\hbar^2 (\nabla \Omega_1)^2}{2\Gamma} \,. }
This is the momentum diffusion rate due to the simulated light force, on resonance.
Away from resonance, the correlation time changes, and there are additional complications, but the basic physics stay the same (see JOSAB paper). The result for arbitrary detuning Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \delta_L} is
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D_{dipole} \approx \frac{\hbar^2 (\nabla \Omega_1)^2}{2\Gamma} \left(\frac{\Omega_1^2}{\Omega_1^2 + 2\delta_L^2}\right)^3 \,. }
Atoms moving in a standing wave: Sisyphus cooling
The stimulated force is a force which we can fully understand with the two-level atom. It is conceptually simple, and we have analytical expressions for the forces and energy levels. Now let us see what happens when the velocity is not just infinitesimal, but is very large. Instead of just a time lag, the atom is now pictured as flying like a roller coaster. This picture will let us see a cooling mechanism known as sisyphus cooling, that is in practice important. In practice, the multi-level nature of real atoms leads to stronger cooling than expected for two levels, but we can see the necessary insight for this mechanism with sisyphus cooling of two-level atoms.
Let us take the atom velocity to satisfy
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k v \ge \Gamma \,, }
meaning that the atom moves through several optical wavelengths within its spontaneous emission lifetime.
The nice thing about the dressed atom picture is that you can show wonderful drawings and diagrams, so let us draw a nice picture of a standing wave and show it for the manifold of dressed atom levels Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2} within Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E(N+1)} What happens is that the standing wave modulates the energy of the atom in the two dressed states:
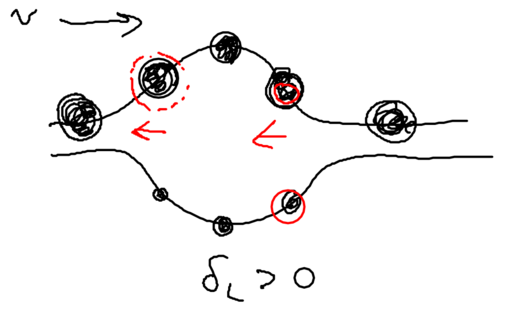
We assume that when we have no light, the ground state (Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |a{\rangle}} , or Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |g{\rangle}} ) is the upper sate, and the excited state (, or Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |e{\rangle}} ) is the lower state. Suppose we start from the left, in this picture, and we go along the standing wave with the atom, which starts in its ground state. Where will the atom emit a photon, with highest probability, switching to the lower state through the radiative cascade? Will this happen at a node, or at an anti-node of the standing wave? The atom has the largest excited state admixture in the anti-nodes of the standing wave, so it is there where the atom emits.
So where the atom end up after emitting a photon? It can end in an upper state, at which point nothing changes with respect to forces on it, since it has stayed in the same dressed level. More interesting is what happens when the radiative cascade leads the atom to the lower dressed level:
When an atom ends up in the lower dressed level, when is it most likely to emit the next photon? The lower level is correlated with the excited state, so when there is no light, it atom is purely in its excited state. As it enters an anti-node, the atom evolves into a superposition of the excited and ground states. So it is most likely to emit when it is purely in the dark, meaning where the splitting of states is minimal.
This happens at the top of the hill. Thus, the physical picture is
that the atom has to climb up a potential hill again whereupon it can
once again emit a photon. In Greek mythology, King Sisyphus did
something bad, so he had to roll a stone up a hill, and when it
reached the top it fell down again, and he then had to roll it up the
hill again. This is what happens with the atoms in this standing
wave: it continuously climbs a hill.
The result we have is that for both dressed states Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1} and , we have a preferential spontaneous emission at the top of the hill. This implies cooling, at the rate proportional to
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle U_0 \Gamma_{1\rightarrow 2} \Pi_1 \,, }
where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle U_0} is the kinetic energy difference between the top and bottom of the standing wave potential hill. is the rate of the switching between sidebands of the radiative cascade.
Experimental implementation
See <refbase>5253</refbase>
This experiment did laser cooling of an atomic beam, and showed that with a blue detuned standing wave the beam velocity distribution narrowed (cooling the atoms), and with a red detuned standing wave it broadened (heating the atoms).
Dressed atoms in a perturbative limit: cooling in a standing wave
Let us now use the dressed atom picture to give, in the simplest limit (a perturbative limit), a summary of concepts of laser cooling in a standing wave. We take Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \delta_L \gg \Omega_1 \gg \Gamma} , neglect factors of the order of unity, and take Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hbar=1} .
If we have a standing wave,
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Omega_1(x) = 2\Omega_1 \cos kx }
the two dressed levels are, to leading order,
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} |1 \rangle &= |a \rangle + \frac{\Omega_1(x)}{\delta_L} |b{\rangle} \\ |2 \rangle &= |b \rangle - \frac{\Omega_1(x)}{\delta_L} |a{\rangle} \,. \end{align}}
These simple expressions give us the transition rate from Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1} to Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2} ,
since the atom must start in the excited state and end up in the ground state. The transition rate from Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2} to Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1} is just
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Gamma_{2\rightarrow 1} = \Gamma \,, }
in this perturbative limit.
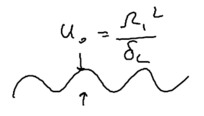
The energy of the dressed states change in the standing wave, giving us what we can call the "dressed state potential," which is physically nothing more than the AC stark shift
with potential height Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle U_0 = \Omega_1^2/\delta_L} .
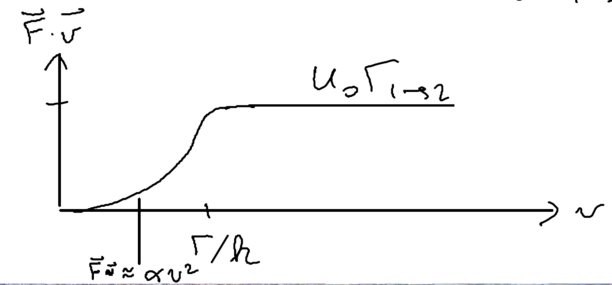
In this picture, we can go back to look at Sisyphus cooling once more, staring with the assumption Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle v\ge \Gamma/k} , but relaxing this to look at intermediate velocities. The cooling rate is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{F}\cdot\vec{v} = U_0 \Gamma_{1\rightarrow 2}} (note that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Gamma_{2\rightarrow 1} = \Gamma} which happens much faster and thus is not limiting). When the velocity of that atom is much smaller, it cannot climb up and down many hills. But we know that the friction force is linear, so we expect what we have in intermediate velocity regimes is that there is some energy loss rate Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha v^2} :
Using the model that we have an initial parabola followed by a straight line, we obtain an estimate for the friction force
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} \alpha &\approx \lim_{v\rightarrow 0} \frac{F(v)}{v} \approx \frac{U_0 \Gamma_{1\rightarrow 2}}{(\Gamma/k)^2} \\ &= \frac{\Omega_1^2}{\delta}\frac{\Gamma\Omega_1^4}{\delta_L^4} \frac{k^2}{\Gamma^2} \\ &= \frac{\Omega_1^6}{\delta_L^5}\frac{k^2}{\Gamma} \,. \end{align}}
Thus, we find very nicely that the perturbative approach gives all the essential physics of what we derived above in a more rigorous manner.
Diffusion
It turns out that the limit of cooling is always higher than the depth of the optical lattice, and to understand this we need to return to the diffusion coefficient. Building on our perturbative expressions, recalling that
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D = \int \langle \Delta F(0)\Delta F(t) \rangle dt \,, }
we may use the fact that the atoms are mainly in state . The interesting situation is when the atoms are on the slope of the standing wave, where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F_1 = -\nabla U_1(v)\approx kU_0} . For a duration , at rate Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Gamma_{1\rightarrow 2}} , the atom jumps and the force also jumps, to the opposite value .
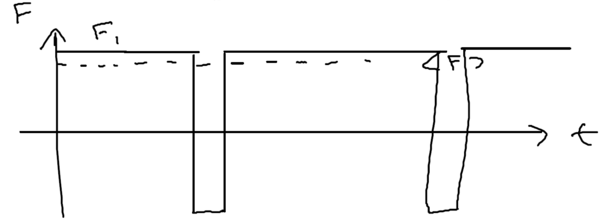
Graphically, we may see what happens by plotting the force seen by a typical atom as a function of time:
In the initial dressed level, the atom sees a constant force, then emits a photon, going to the other dressed level (essentially the excited state) for a short time Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1/\Gamma} , before returning. The dominant contribution to the fluctuation in the force comes from these brief spikes, so the momentum diffusion coefficient can be written down by inspection as
where the expression on the right is the probability that the atom is in state Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |2{\rangle}} , in our picture.
Plugging in numbers, we get
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D = k^2 U_0^2 \Gamma_{1\rightarrow 2}/\Gamma^2 }
The ultimate temperature limit achievable from this cooling is thus
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k_B T = \frac{D}{\alpha} = U_0 \,. }
This means that, according to the perturbative approach, there is competition between cooling and heating, resulting in a minimum temperature set by the depth of the lattice. Keep in mind that cooling to zero temperature cannot be accomplished either, using this mechanism, since the analysis assumes that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle U_0>\Gamma} . Two-level atoms therefore cannot be localized in an optical lattice by stimulated laser cooling. The only reason why localization of atoms in a lattice is possible is through other mechanisms, such as polarization gradient cooling.
Four physical pictures of the dipole force
Let us recall four different physical pictures for deriving the dipole force:
- Optical Bloch equations.
- Dressed atom picture.
- Classical dipole model of atom, in electric field.
- Macroscopic dielectric object.
Classical dipole
The classical dipole model is based on the fact that the force seen by the dipole depends on whether the electric dipole is in-phase or out-of-phase with the driving field Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E} . This gives either an attractive or repulsive force.
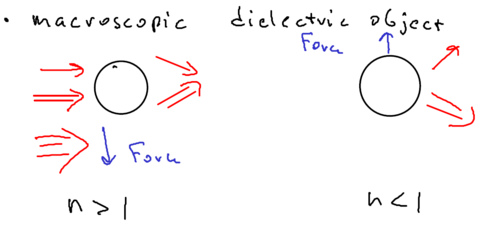
Macroscopic dielectric object
The picture of a macroscopic dielectric object assumes, for example, that you have a microscopic sphere of glass, with a laser beam which is stronger in part of space than another:
So if the microsphere were an atom, with index of refraction greater than one, then the sphere moves toward the field maximum. This is because if you imagine the microsphere is a prism (with index greater than one), then the upper beam is deflected downwards and the lower beam is deflected upwards, such that the sphere acts like a lens. The sphere then is forced to move to compensate for the imbalance in the change of photon momentum of the two refracted beams.
Optical tweezers have made an impact on biology almost as large as the impact of laser cooling on atomic physics. With optical tweezers, one can now manipulate objects within a cell. The people who demonstrated optical tweezers first were the same people who demonstrated laser cooling first: Art Ashkin, who teamed up with Steven Chu, at Bell Labs, in the late 1980's.
Electric and magnetic components of the dipole force
Now a question. We have discussed the stimulated light force, also called the dipole force, or the reactive force. Is this force an {\em electric} force (the force on an electric dipole), a magnetic force (Lorentz force), or both? In other words, imagine an atom is in the potential of the standing wave in an optical lattice. Is the potential due to electric forces or magnetic forces? Intuition says that the force is electric, because the electric force is generally larger than the magnetic one. However, that turns out to be incorrect, and indeed it is the Lorentz force which gives rise to the electric dipole potential. At this point, you may completely appreciate the electric dipole potential, since by writing Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{d}\cdot \vec{E}} you actually include magnetic forces.
Static fields
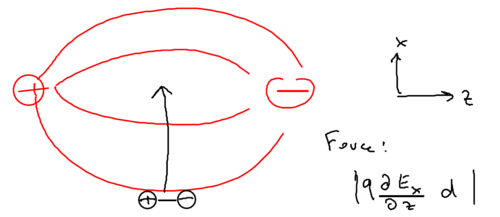
In the limit of DC fields, everything does have to be electric, so let us begin with electrostatics. Consider two charges separated by distance :
The energy of this configuration is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha E^2/2} , due to the polarizability of the atom. Now imagine the charge is placed in an inhomogeneous field:
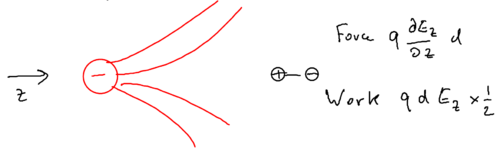
There is a force on the two halves of the dipole, and the two would cancel unless there is a gradient, so the force on the dipole is
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle q \frac{\partial E_z}{\partial z} d }
so the work done is
When we move the dipole not along the electric field, but rather, perpendicular to the electric field, consider what happens:
Now, what is the force on the dipole? What matters is the Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hat{x}} component of the force, which is caused by the component of the electric field, or more specifically, it matters that the Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hat{x}} component changes by the distance separating the two charges in the dipole.
Now, we want to use the fact that in electrostatics, the curl of the electric field is zero, and therefore by vector calculus,
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|q\frac{\partial E_z}{\partial x} d \right| = \left| q \frac{\partial E_z}{\partial z} d \right| }
and the work done by moving the dipole in from infinity is . This derivation relied on there being no magnetic fields, because otherwise the curl of the electric field would not be zero.
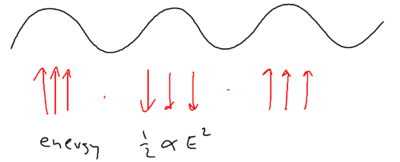
Oscillating fields
Now consider an oscillating dipole in a standing wave, created by counter-propagating laser fields, so that the electric field is perpendicular:
Recall that the potential from the AC Stark shift is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha E^2/2} . Let us understand what the force is, which provides this periodic potential. Well, if you have a plane standing wave, that means you can draw the above picture extended up and down. What is the electric force on the dipole in that case? The dipole moment and the gradient of the electric field are orthogonal, in this configuration. There is thus no gradient in the field along the direction of the dipole. Thus, in a plane standing wave, the electric force is identically zero, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{p} \perp \vec{\nabla}E} . So where does the force come from?
It must originate from the Lorentz force. In this scenario, recall that the Lorentz force is . There is an oscillating dipole in the scenario. If you take the derivative of the optical dipole potential, the Lorentz force is
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F_{Lorentz} = \frac{d}{dz}\left(\frac{1}{2} \alpha E^2 \right) \,. }
The standing wave has both electric and magnetic components,
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} \vec{E} &= A_0 \hat{e}_x \cos kz \sin \omega t \\ \vec{B} &= -A_0 \hat{e}_y \sin kz \cos \omega t \end{align}}
The dipole potential, averaged over a cycle, is
Recall that the dipole moment is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle p=\alpha E} . Thus, the Lorentz force is
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle q \frac{\vec{v}}{c} \times \vec{B} = \frac{\dot{\vec{p}}}{c}\times \vec{B} \,. }
Inserting the expression above for the electric field, we thus obtain this expression for the Lorentz force:
Now averaging, we find
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} \bar{F}_{lorentz} &= -\frac{1}{2} \alpha A_0^2 k \cos kz \sin kz \\ &= \frac{d}{dz} \left(\frac{1}{4} A_0^2 \cos^2 kz\right) \\ &= \frac{d}{dt} \left(\frac{1}{2} \alpha \bar{E}^2\right) \,, \end{align}}
where we now recognize the term on the right in the parentheses as the dipole potential.
Thus, we see that the stimulated force can be either electrical, that is of the form Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle p_z\nabla E_z} , or magnetic, that is, of the form , in nature. Each of the two forces may not be curl free, but the sum of both can be.
Energy conservation and dipole forces
When we considered the dipole force earlier, we looked at how it arises from a redistribution of photons. If you have a monochromatic array of beams, the dipole does not arise due to spontaneous emission, but rather, because photons Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hbar\omega} are taken from one beam and put into another beam. So how can this result in a change of energy for the atom?
Energy conservation in blue molasses picture
Well, energy exchange does occur, because as we saw atoms flying along a blue standing wave, in the picture of "blue molasses," sees a damping force as it walks up the potential hills:
When atoms slow down due to this viscous force, where has the kinetic energy of the atoms gone?
If you have an ordinary molasses, the energy is radiated away. The basic ingredients available, therefore, are photon absorption, stimulated emission, and spontaneous emission. Recall that in Sisyphus cooling, an atom does emit a photon after climbing up a hill. From the upper dressed level, it emits a blue detuned photon, when the sideband splitting is larger. From the lower dressed level, it emits at the top of the potential, at which the sideband splitting is smaller. The upper sideband is emitted at a larger Rabi frequency than the lower sideband.
The principle behind this observation is that spontaneous emission is once again responsible for the removal of entropy involved in the laser cooling process, and energy is removed along with the entropy change.
One way for providing dissipation is thus radiation into the sidebands.
Transient energy conservation picture
Another scenario for understanding energy conservation is the following transient experiment. Imagine that you have an atom in the harmonic potential of an optical dipole trap.
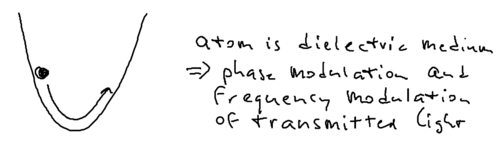
In extremely far detuned optical lattices, there is essentially no spontaneous emission. The atom oscillating in the dipole trap, say at 1 Hz, going from high to low energy. It is clear this energy must be transformed into the light field, and the question is what actually happens. But it turns out the energy does not emit through spontaneous emission.
What happens is that the moment the atoms reach the bottom of the trap, they have a maximum in energy. The photons which pass through the atom at that point are red shifted. When the atom climbs up the potential hill, the photons are blue shifted. While the atom oscillates, the photons which arrive are blue and red shifted. There is no spontaneous emission in this transient picture, but there is phase modulation of the laser which shifts the laser frequencies.
In principle, this frequency shift should be observable in an experiment. In practice, this is complicated by the fact that real experiments involve spatially inhomogeneous beams, so there is some momentum change as well as phase change of photons involved.
References
- Important paper: J. Dalibard and C. Cohen-Tannoudji, J. Opt. Soc. Am .B 2, 1707 (1985) Download