Laser cooling of trapped ions

A simple comparison of potential depths provides useful perspective for understanding the experimental convenience of trapped ions versus neutral atoms. Recall that the depth of a dipole force trap is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hbar\Omega^2/4\delta} ; for a $1$ Watt laser at Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1.06} $\mu$m focused to a Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 10} Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mu} m waist used to trap atomic sodium, the trap depth is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 260} Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mu} K; this is not much more than the Doppler cooling limit. A magneto-optical trap is much deeper; for typical laboratory magnetic field gradients, depths of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \sim 10^{-2}} K can be obtained. In contrast, a singly charged ion in an electric field of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \sim 100} V/mm, which is easily obtained in the laboratory, the depth of an RF ion trap can be over Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \sim 10^4} K. This allows hot ions to be readily trapped, and subsequently laser cooled.

A unique aspect of trapped ions, versus neutral atoms, is the importance of the role of the trapping potential in the laser cooling process. As the motion of ions is reduced to the characteristic size of their confining potential, quantum aspects of their motion become accessible. This allows a form of laser cooling known as {\em resolved sideband cooling} to be performed, through which ions can be cooled to their motional ground states.

Physical system

Two kinds of ion traps are widely used. The Penning trap employs static magnetic and electric fields to confine ions. The Paul trap employs just oscillating electric fields, and is the configuration we shall study here.

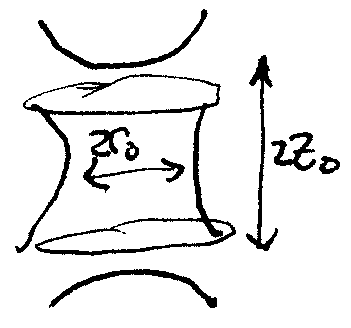
Consider this electrode configuration:

The inner surfaces are hyperboloids, the top and bottom electrodes are tied together, and the drive voltage oscillates sinusoidally at frequency $\Omega$, such that near the center of the electrodes, the potential is

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V(t) = \frac{V_0\cos\Omega t}{2r_0^2}(x^2+y^2-2z^2) \,. }

For a singly charged ion of mass $M$ located in this trap, the solutions to the equations of motion balancing the force of the electric field against ion motion are

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \ddot{x_k} + 2 q_k \frac{\Omega^2}{4} x_k \cos\Omega t = 0 \,. }

This is a Mathieu equation, where

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle q_x = q_y = \frac{2e V_0}{M r_0^2 \Omega^2} }

and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle q_z = -2q_x} is a non-dimensional parameter. When Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle q\leq 0.9} , stable solutions exist. These solutions describe a fast oscillation known as micromotion, superposed on top of a slow harmonic motion with secular frequency

$\nu _{x}={\frac {q_{x}\Omega }{2{\sqrt {2}}}}\,.$

The amplitude of micromotion, in a well designed experiment, is very small, and thus can be neglected. The dominant physics of a trapped ion system are thus described by an atom coupled to a simple harmonic oscillator.

Typically, RF frequencies are Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Omega \sim 10} MHz or higher, and secular frequencies are $\nu \sim 1$ MHz. These may be compared with typical spontaneous emission rates of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Gamma \sim 1} MHz, and the recoil energy from a single photon emission of

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \omega_R = \frac{\hbar k^2}{2M} \sim 100~{\rm kHz} \,, }

where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \omega_L = ck} is the frequency of the laser. The three systems involved in this scenario are thus the trapped ion, with its atomic levels, the harmonic oscillator, and incident electromagnetic radiation on the atom. That is, we have a system of atom + photon + phonon, where the phonon represents quantized vibrational modes of the trapped atom.

Hamiltonian: classical field + 2-level atom

Let us model the trapped ion system initially using a classical field, and neglect spontaneous emission. Let us also approximate the atom to be a two-level system. The Hamiltonian for such a system has the interaction

${\begin{array}{rcl}H_{I}&=&{\vec {d}}\cdot {\vec {E}}\\&=&\left[{d{\vec {S}}}\right]\cdot \left[{E{\hat {x}}\cos(kz-\omega t)}\right]\,,\end{array}}$

where we use Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{S}} to denote the spin of the atom, and assume the electric field is along the Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hat{x}} direction. Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z} is the position of the ion in the field; we quantize this degree of freedom by representing it as motion in a harmonic oscillator, letting

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z = z_0 (a+ a^\dagger ) \,, }

where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z_0} is the characteristic length scale of the harmonic motion. The interaction Hamiltonian can thus be written as

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H_I = dE S_x \cos \left[ { kz_0 (a+ a^\dagger )-\omega t } \right] \,. }

The Lamb-Dicke parameter

When the ions are well-confined, a natural small parameter arises, in which this interaction can be expanded. This parameter describes the extent to which the ion is localized in the trap, relative to the incident light. We define this Lamb-Dicke parameter as

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \eta = k z_0 = \frac{2\pi z_0}{\lambda} = \frac{\omega_R}{\nu} \,. }

It is the ratio of the size of the ground state wavefunction of the motion in the harmonic oscillator, to the incident laser wavelength:

\noindent Note that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \eta} can also be understood as being the ratio of the recoil frequency to the vibrational frequency.

Expansion in the Lamb-Dicke parameter

Let us now expand Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H_I} to leading order in Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \eta} . Recall that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S_x = (S_+ + S_-)/2} , and let $\Omega =(d\times E)/2\hbar$ be the Rabi frequency. In terms of these,

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} H_I &=& \hbar\Omega \left[ { S_+ + S_- } \right] \cos \left[ { \eta(a+ a^\dagger )-\omega t } \right] \\ &=& \frac{\hbar\Omega}{2} \left[ { S_+ + S_- } \right] \left[ { e^{i\eta(a+ a^\dagger )-\omega t} + e^{-i\eta(a+ a^\dagger )+\omega t} } \right] \,. \end{array}}

The exponentials can be expanded to leading order in $\eta$, resulting in terms of the form Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left[ { 1+i\eta(a+ a^\dagger )} \right] e^{-i\omega t}} , such that

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} H_I &=& \frac{\hbar\Omega}{2} \left[ { S_+ e^{-i\omega t} + S_- e^{i\omega t}} \right] + \frac{i\eta\hbar\Omega}{2}(S_+ + S_-) \left[ {a+ a^\dagger } \right] \left( { e^{-i\omega t} - e^{i\omega t} } \right) \,. \end{array}}

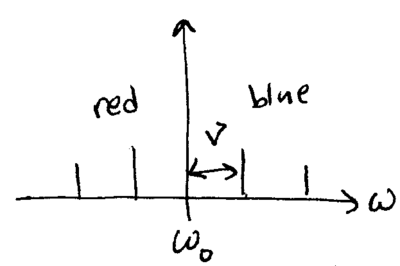
In the above expansion, it is assumed that the laser's frequency is close to that of the atomic transition, such that to good approximation, terms oscillating as $\sim 2\omega$ can be dropped (the rotating wave approximation). The first term in this expression describes the carrier transition, in which the light changes the internal atomic state, and the second term describes sideband transitions, in which the light changes both the internal atomic state as well as its motional state. These transitions have a frequency spectrum which looks like:

Moving into the rotating frame defined by the atom's internal and motional states simplifies this Hamiltonian, providing a time-independent form. Let this frame be defined by the system Hamiltonian

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H_0 = \hbar\omega_0 S_z + \hbar\nu a^\dagger a \,, }

such that in the rotating frame, operators are transformed according to

${\begin{array}{rcl}S_{\pm }&\rightarrow &S_{\pm }e^{\pm i\omega _{0}t}\\a&\rightarrow &ae^{-i\nu t}\\a^{\dagger }&\rightarrow &a^{\dagger }e^{i\nu t}\,.\end{array}}$

The interaction Hamiltonian in this frame is approximated by

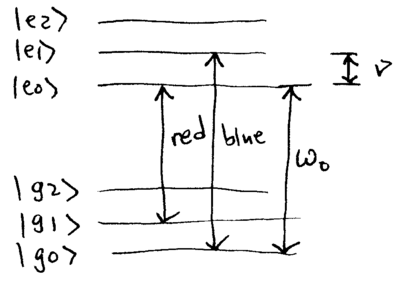
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} H'_I &=& e^{iH_0 t/\hbar} H_I e^{-iH_0 t/\hbar} \\ &=& \left\{ \begin{array}{lr} \frac{\hbar\Omega}{2} \left[ { S_+ + S_- } \right] & {\omega\approx \omega_0 ~~{\rm carrier}} \\ \frac{i\eta\hbar\Omega}{2}(S_+ a^\dagger - S_- a) & {\rm \omega\approx \omega_0+\nu ~~{\rm blue~sideband}} \\ \frac{i\eta\hbar\Omega}{2}(S_+ a - S_- a^\dagger ) & {\rm \omega\approx \omega_0-\nu ~~{\rm red~sideband}} \end{array} \right. \,. \end{array}}

The first few energy levels are diagrammed here:

Resolved sideband cooling

When the ion is hot, doppler shifts will dominate, and the sidebands will be unresolvable. However, for many ions, standard doppler cooling is sufficient to reach the point at which the motional sidebands become resolvable. In that regime, a different cooling method can be applied; this method is known as {\em resolved sideband cooling}.

The basic idea of this method is analogous to optical pumping: the laser is detuned to cause transitions on the red sideband of the ion, removing one quantum of motion for each photon absorbed. Just as for laser cooling of neutral atoms, let us calculate the cooling limit of such a procedure.

Let Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |n{\rangle}} be an eigenstate of the harmonic motion of the ion, with motional quantum number $n$, and recall that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a|n{\rangle}=\sqrt{n}|n-1{\rangle}} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a^\dagger |n{\rangle}=\sqrt{n+1}|n+1{\rangle}} . The transition amplitude between motional states $|n{\rangle }$ and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |n\pm 1{\rangle}} is given by this matrix element of the interaction Hamiltonian:

${\begin{array}{rcl}\langle n\pm 1|H'_{I}|n\rangle \approx \langle n\pm 1|i\eta {\frac {\hbar \Omega }{2}}\left[{{\sqrt {n}}|n-1\rangle \langle n|-{\sqrt {n+1}}|n+1\rangle \langle n|}\right]|n{\rangle }\,,\end{array}}$

in the limit that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \eta} is small, and we're interested only in terms which couple $n$ and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n+1} . Let us define

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle W(\Delta) = \frac{1}{\left(\frac{2\Delta}{\Gamma}\right)^2 + 1} }

as the lineshape function; it captures the frequency dependent response of the atom to a laser detuned by Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta = \omega_L-\omega_0} from the atomic resonance.

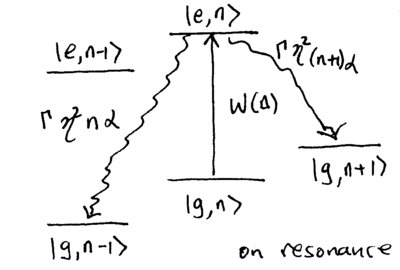
The incident laser light can connect three basic transitions: the carrier, the blue sideband, and the red sideband. How do those transitions contribute to changing the motional quantum number Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n} ? Excitations of the carrier transition couple $|g,n{\rangle }$ (using Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |{\rm atom},{\rm motion}{\rangle}} ) to the state Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |e,n{\rangle}} , with rate proportional to Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle W(\Delta)} . The excited state can then decay to Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |g,n-1{\rangle}} or Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |g,n+1{\rangle}} , changing the motional quantum number; these transitions occur with rates proportional to $\Gamma \eta ^{2}n\alpha$ and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Gamma \eta^2(n+1)\alpha} , respectively, where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha} is a geometric factor describing the probability for spontaneous emission to change Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n} . For dipole emission into free space, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha\approx 2/5} . These transitions are diagrammed as follows:

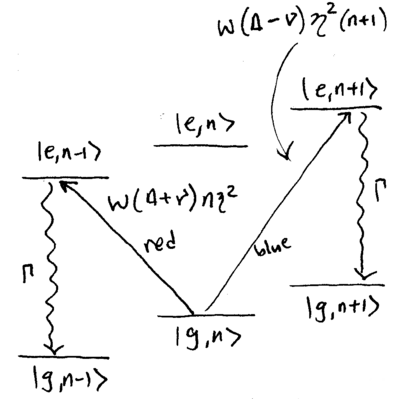
On the blue and red sidebands, absorption happens at a rate proportional to Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n\eta^2 W(\Delta+\nu)} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (n+1)\eta^2 W(\Delta-\nu)} , while spontaneous emission happens at rate proportional to $\Gamma$, all-together connecting Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |g,n{\rangle}} with Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |g,n\pm 1{\rangle}} . These transitions are diagrammed as follows:

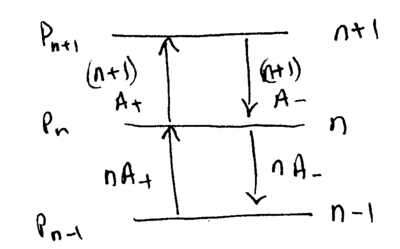
To obtain the cooling limits of resolved sideband cooling, we need to write down the rate equations for these phonon-number changing carrier and sideband excitation processes, and solve for the steady state average phonon number. For the transitions

where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle P_n} is the probability of being in the Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n} phonon state, the transition rate coefficients are

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle A_\pm = \frac{\Omega^2}{\Gamma} \eta^2 \left[ { W(\Delta\mp \nu)+\alpha W(\Delta) } \right] \,. }

The first term in the brackets comes from the sideband excitations, and the second from the carrier. The rate equations for the populations are thus

${\dot {P}}_{n}=\left[{nP_{n-1}-(n+1)P_{n}}\right]A_{+}+\left[{(n+1)P_{n+1}-nP_{n}}\right]A_{-}\,.$

The average phonon number Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \bar{n}} is

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \bar{n} = \sum_{n=0}^\infty n P_n \,, }

which evolves as

${\begin{array}{rcl}{\frac {d}{dt}}{\bar {n}}&=&A_{-}\left[{\sum _{n}n(n+1)P_{n+1}-n^{2}P_{n}}\right]+A_{+}\left[{\sum _{n}n^{2}P_{n-1}-n(n+1)P_{n}}\right]\\&=&-{\bar {n}}A_{-}+({\bar {n}}+1)A_{+}\,.\end{array}}$

This differential equation has a solution in which Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \bar{n}(t)} decays exponentially as Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle e^{(A_- - A_+)t}} to an equilibrium average phonon number ${\bar {n}}_{eq}$,

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \bar{n}_{eq} = \frac{A_+}{A_- - A_+} \,. }

The corresponding equilibrium temperature is given by

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{P_{n+1}}{P_n} = e^{-\hbar\nu/k_BT} = \frac{A_+}{A_-} \,. }

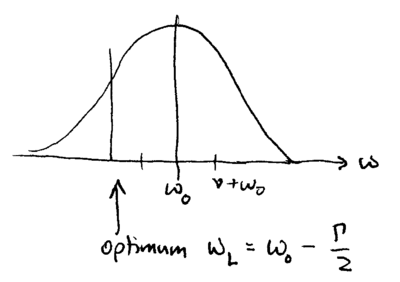
It is insightful to evaluate these expressions in two limits. When the ion is weakly confined, such that $\Gamma \gg \nu$, then the sidebands are poorly resolved:

The optimum detuning is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta = \omega_L-\omega_0 = -\Gamma/2} , and cooling corresponds to standard Doppler cooling of free particles, giving

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \bar{n} = \frac{\Gamma}{2\nu} \,, }

with a final temperature of around

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k_BT = \hbar\nu \left( { \bar{n}+\frac{1}{2} } \right) \approx \frac{\hbar\Gamma}{2} \,. }

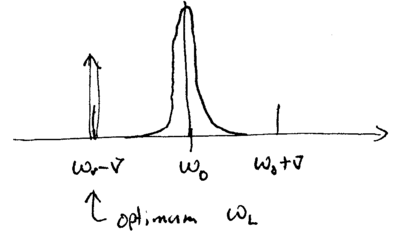
Including geometric factors reduces this slightly, by Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (1+\alpha)/2} . The strong confinement limit is reached when Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Gamma\ll \nu} , so that individual sidebands are well resolved:

This is the case of resolved sideband cooling, as mentioned above. For this case, the laser is detuned to Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \omega_L = \omega_0-\nu} , such that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle A_+ \sim \alpha W(-\nu)} , and $A_{-}\sim W(0)=1$, such that the equilibrium average phonon number, given by

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \bar{n} = \frac{1}{\frac{A_-}{A_+}-1} \,, }

is approximately Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \bar{n} \approx (\alpha \Gamma/2\nu)^2} , which is much less than one. This corresponds to a temperature of

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k_BT = \frac{\hbar\nu}{-\log \frac{A_+}{A_-}} = \frac{\hbar\nu}{2\log \left( { \frac{2\nu}{\alpha\Gamma} } \right) } \,. }

Essentially, the final temperature is set by zero-point motion of the ion in the harmonic trap.