Atoms in Magnetic Fields

In this section we treat the interaction of the electron's orbital and spin angular momentum with external static magnetic fields. Previously, in the chapter on fine structure, we have considered the spin-orbit interaction: the coupling of electron spin to the magnetic field generated by the nucleus (which appears to move about the electron in the electron's rest frame). The spin orbit interaction causes the orbital and spin angular momenta of the electron to couple together to produce a total spin which then couples to the external field; the magnitude of this coupling is calculated here, first for weak external fields.

The Land\'{e} \texorpdfstring{$g$}{g}-factor

Magnetic moment of circulating charge (classical)

The energy of interaction of a classical magnetic moment ${\bf {\mu }}$ with a magnetic field { ${\bf {B}}$ } is

$U=-{\bf {\mu }}\cdot {\bf {B}}$

indicating that the torque tends to align the moment along the field. In classical electrodynamics the magnetic moment of a moving point particle about some point in space is independent of the path which it takes, but depends only on the product of the ratio of its charge to mass $m$, and angular momentum $\ell$. This result follows from the definitions of angular momentum

${\bf {L}}\equiv {\bf {r}}\times {\bf {p}}=m[{\bf {r}}\times {\bf {v}}]$

and magnetic moment

${\bf {\mu }}\equiv {\frac {1}{2}}{\bf {r}}\times {\bf {i}}={\frac {q}{2}}[{\bf {r}}\times {\bf {v}}]$

where { ${\bf {i}}$ } is the current and { ${\bf {v}}$ } the velocity (see Jackson Ch.5). The equality of the bracketed terms implies

${\bf {\mu }}={\frac {q}{2m}}{\bf {L}}\equiv \gamma _{\ell }{\bf {L}}$

where $\gamma _{\ell }$ is referred to as the \emph{gyromagnetic ratio}. This is a general result for any turbulently rotating blob provided only that it has a constant ratio of charge to mass throughout. For an electron with orbital angular momentum ${\bf {\ell }}$

${\bf {\mu }}_{\ell }=-{\frac {e}{2m}}{\bf {L}}\equiv -\mu _{B}{\bf {L}}/\hbar$

which is the classical result, and $\mu _{B}$ is the \emph{Bohr magneton}:

Failed to parse (unknown function "\unit"): {\displaystyle \mu_B = \frac{e\hbar}{2m} = \unit{9.27408(4)\times 10^{-24}}{\joule\per\tesla} \rightarrow \unit{1.39983 \times 10^4}{\mega\hertz} \times B/(\text{Tesla}) }

Intrinsic electron spin and magnetic moment

When Uhlenbeck and Goudsmit suggested \cite{Uhlenbeck1926} that the electron had an intrinsic spin $S={\frac {1}{2}}$, it soon became apparent that it had a magnetic moment twice as large as would be expected on the basis of Eq.\ \ref{EQ_magmoment4}. (This implies that the electron cannot be made out of material with a uniform ratio of charge to mass.) This is accounted for by writing for the intrinsic electron moment

${\bf {\mu }}_{s}=-g_{e}\mu _{B}{\bf {S}}/\hbar$

where the quantity $g_{e}=2$ is called the electron $g$-factor. (The negative sign permits treating $g_{e}$ as a positive quantity, which is the convention.) This factor was predicted by the Dirac theory of the electron, probably its greatest triumph. Later, experiments by Kusch, followed by Crane et al., and then by Dehmelt and coworkers, have shown (for both electrons and positrons).

${\frac {g_{e}}{2}}=1.0011596521869(41)$

This result has been calculated from quantum electrodynamics, which gives

${\frac {g_{e}}{2}}=1+{\frac {1}{2}}{\left({\frac {\alpha }{\pi }}\right)}-0.3258{\left({\frac {\alpha }{\pi }}\right)^{2}}+0.13{\left({\frac {\alpha }{\pi }}\right)^{3}}+\cdots$

The agreement betwen the prediction of quantum electrodynamics and experiment on the electron g-factor is often cited as the most precise test of theory in all of physics.

Vector model of the Land\'{e} \texorpdfstring{$g$}{g}-factor

In zero or weak magnetic field the spin orbit interaction couples { ${\bf {S}}$ } and ${\bf {L}}$ together to form ${\bf {J}}={\bf {L}}+{\bf {S}}$, and this resultant angular momentum interacts with the applied magnetic field with an energy

$U=-g_{j}\mu _{B}{\bf {B}}\cdot {\bf {J}}/\hbar$

which defines $g_{j}$. The interaction of the field is actually with ${\bf {\mu _{s}}}$ and ${\bf {\mu _{\ell }}}$, however $g_{j}$ is not simply related to these quantities because ${\bf {\mu _{s}}}$ and ${\bf {\mu _{\ell }}}$ precess about { ${\bf {J}}$ } instead of the field. As Land\'{e} showed in investigations of angular momentum coupling of different electrons \cite{Lande1923}, it is a simple matter to find $g_{j}$ by calculating the sum of the projections of ${\bf {\mu _{s}}}$ and ${\bf {\mu _{\ell }}}$ onto { ${\bf {J}}$ }. The projection of ${\bf {\mu _{\ell }}}$ on { ${\bf {J}}$ } is

$\mu _{\ell j}={\frac {-\mu _{B}|{\bf {L}}|}{\hbar }}{\frac {{\bf {L}}\cdot {\bf {J}}}{|{{\bf {L}}|{\bf {J}}}}}$

The projection of ${\bf {\mu _{s}}}$ on { ${\bf {J}}$ } is

$\mu _{sj}=-g_{e}\mu _{B}{\frac {|{\bf {S}}|}{\hbar }}{\frac {{\bf {S}}\cdot {\bf {J}}}{\left|{\bf {S}}\right|}}{\bf {J}}|$

The definition of $g_{j}$ gives

$g_{j}=-{\frac {(\mu _{\ell j}+\mu _{sj})}{\left|{\bf {J}}\right|}}\mu _{B}/\hbar$

Taking $g_{e}=2$

${\begin{aligned}g_{j}&={\frac {{\bf {L}}\cdot ({\bf {L}}+{\bf {S}})+2{\bf {S}}\cdot ({\bf {L}}+{\bf {S}})}{\left|{\bf {J}}\right|}}^{2}\\&=1+{\frac {j(j+1)+s(s+1)-\ell (\ell +1)}{2j(j+1)}}\end{aligned}}$

using $2{\bf {L}}\cdot {\bf {S}}={\bf {J}}^{2}-{\bf {L}}^{2}-{\bf {S}}^{2}$. If a transition from a level with angular momentum $j^{\prime }$ to a level with $j^{\prime \prime }$ takes place in a magnetic field, the resulting spectral line will be split into three or more components---a phenomenon known as the Zeeman effect. For transitions with a particular $\Delta m$, say $\Delta m=-1$, the components will have shifts

$\Delta E_{z,m,-1}={\left[g_{j^{\prime }}m-g_{j^{\prime \prime }}(m-1)\right]}\mu _{B}B={\left[(g_{j^{\prime }}-g_{j^{\prime \prime }})m-g_{j^{\prime \prime }}\right]}\mu _{B}B$

If $g_{j^{\prime }}=g_{j^{\prime \prime }}$ (or if $j^{\prime }$ or $j^{\prime \prime }=0$) then $\Delta E_{z,m,-1}$ will not depend on $m$ (or there will be only one transition with $\Delta m=-1$) and there will be only 3 components of the line ($\Delta m=+1,0-1$); this is called the normal Zeeman splitting. If neither of these conditions holds, the line will be split into more than 3 components and the Zeeman structure is termed "anomalous"---it can't be explained with classical atomic models.

Hyperfine structure in an applied field

The Hamiltonian in an applied field ${\bf {B}}_{0}$ is

$H=ah{\bf {I}}\cdot {\bf {J}}-{\bf {\mu }}_{J}\cdot {\bf {B}}_{0}-{\bf {\mu }}_{I}\cdot {\bf {B}}_{0}=ah{\bf {I}}\cdot {\bf {J}}+g_{j}\mu _{B}{\bf {J}}\cdot {\bf {B}}_{0}-g_{I}\mu _{B}{\bf {I}}\cdot {\bf {B}}_{0}$

By convention, we take ${\bf {\mu }}_{J}=-g_{j}\mu _{B}{\bf {J}}$. Note that we are expressing the nuclear moment in terms of the Bohr magneton, and that $g_{I}<<g_{j}$. (The nuclear moment is often expressed in terms of the nuclear magneton, in which case ${\bf {\mu }}_{I}$ = $g_{i}^{\prime }\mu _{N}{\bf {I}}$, where $\mu _{N}$ is the nuclear magneton.) What are the quantum numbers and energies? Before discussing the general solution, let us look at the limiting cases.

Low field

The total angular momentum is ${\bf {F}}={\bf {I}}+{\bf {J}}$. In low field, $F$ and $m_{F}$ are good quantum numbers. Each level $F$ contains $(2F+1)$ degenerate states. In a weak field ${\bf {B}}_{0}$ the ($2F+1$) fold degeneracy is lifted. We can treat the terms

$H_{z}=-({\bf {\mu }}_{j}+{\bf {\mu }}_{I})\cdot {\bf {B}}_{0}$

as a perturbation. ${\bf {J}}$ and ${\bf {I}}$ are not good quantum numbers, only their components parallel to ${\bf {F}}$ are important. Thus

$\langle {{\bf {J}}\cdot {\bf {B}}_{0}}\rangle ={\frac {\langle {{\bf {J}}\rangle \cdot {\bf {F}}}{\bf {F}}\cdot {\bf {B}}_{0}}{F^{2}}}$

$H_{z}=-\mu _{B}{\left[-g_{j}({\bf {J}}\cdot {\bf {F}})+g_{I}({\bf {I}}\cdot {\bf {F}})\right]}{\frac {{\bf {F}}\cdot {\bf {B}}_{0}}{F^{2}}}$

Since $g_{I}\ll g_{j}$, we can usually neglect it. We can rewrite this result as

$H_{z}=g_{F}\mu _{B}mB_{0}$

$g_{F}={\frac {\langle {{\bf {J}}\cdot {\bf {F}}}\rangle }{F^{2}}}g_{j}={\frac {g_{j}}{2}}{\frac {F(F+1)+j(j+1)-I(I+1)}{F(F+1)}}$

For example, let $I=3/2$, $j=1/2$ and $F=1{\text{ or }}2$. Then

${\begin{aligned}F&=1:&W(1)&=-(5/4)ah&g_{F}&=-g_{j}/4\\F&=2:&W(2)&=(3/4)ah&g_{F}&=g_{j}/4\end{aligned}}$

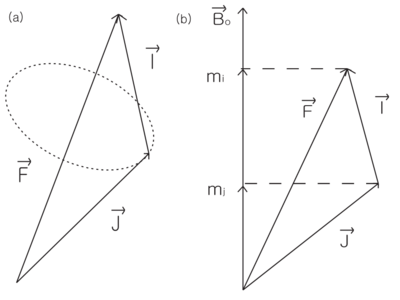
\begin{figure} \centering

\caption{Total angular momentum F=I+J. (a) In low field, only the components of J and I parallel to F are important. (b) In high field, $m_{i}$ and $m_{j}$ are good quantum numbers.}

\end{figure}

High field

If ${\bf {\mu }}_{j}\cdot {\bf {B}}_{0}\gg ah{\bf {I}}\cdot {\bf {J}}$, then { ${\bf {J}}$ } is quantized along ${\bf {B}}_{0}$. Although ${\bf {\mu }}_{I}\cdot {\bf {B}}_{0}$ is not necessarily large compared to the hyperfine interaction, the ${\bf {I}}\cdot {\bf {J}}$ coupling assures that { ${\bf {I}}$ } is also quantized along ${\bf {B}}_{0}$. Thus $m_{I}$ and $m_{j}$ are good quantum numbers. In this case, Eq.\ \ref{EQ_hsaf1} can be written

$H=ahm_{i}m_{j}+g_{j}\mu _{B}m_{j}B_{0}-g_{I}\mu _{B}m_{I}B_{0}$

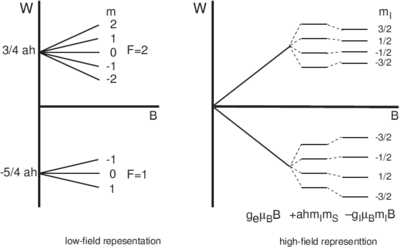
The second term on the right is largest. Usually the first term is next largest, and the nuclear terms is smallest. Figure \ref{fig:B-field-levels} shows low and high field behavior for hyperfine structure for $I=3/2,J=1/2$. \begin{figure} \centering

\caption{Energy level structure for a single-electron atom with nuclear spin 3/2 in the limits of low and high fields.}

\end{figure}

General solution

Finding eigenfunctions and eigenvalues of the hyperfine Hamiltonian for arbitrary field requires diagonalizing the energy matrix in some suitable representation. To obtain a rough idea of the expected results, one can smoothly connect the energy levels at low and high field, bearing in mind that $m=m_{I}+m_{j}$ is a good quantum number at all fields. For $J=1/2$, the eigenvalues of (Eq.\ \ref{EQ_hsaf1}) can be found exactly. The energies are given by the Breit-Rabi formula

$W(m)=-{\frac {1}{2}}{\frac {\Delta W}{2I+1}}-g_{I}\mu _{B}B_{0}m\pm {\frac {\Delta W}{2}}{\sqrt {1+{\frac {4mx}{2I+1}}+x^{2}}},$

where the $+$ sign is for $F=I+1/2$, and the $-$ sign is for $F=I-1/2$. $\Delta W$ is the zero field energy separation.

$\Delta W=W(F=I+1/2)-W(F=I-1/2)=ah{\left({\frac {2I+1}{2}}\right)}$

The parameter $x$ is given by

$x={\frac {(g_{e}+g_{I})\mu _{B}B_{0}}{\Delta W}}$

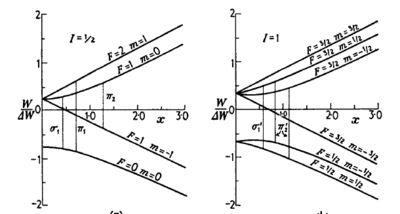
Physically, $x$ is the ratio of the paramagnetic interaction (the "Zeeman energy") to the hyperfine separation. The Breit-Rabi energy level diagram for hydrogen and deuterium are shown in figure \ref{fig:Breit-Rabi}. The units reflect current interest in atom trapping. Low-field quantum numbers are shown. It is left as an exercise to identify the high field quantum numbers. \begin{figure} \centering

\caption{Energy level structure for a single-electron atom with nucleaar spin I = 1/2, such as hydrogen (left), and I = 1, such as deuterium (right). From {\it Molecular Beams} by N.F. Ramsey \cite{Ramsey1956}.}

\end{figure} \putbib