Difference between revisions of "Casimir interaction"
imported>Ichuang |
imported>Ichuang |
||
| Line 93: | Line 93: | ||
We want to compare mode densities, so let us embed our capacitor of | We want to compare mode densities, so let us embed our capacitor of | ||
| − | spacing <math>L</math> within a bigger capacitor of spacing <math>L_0</math> | + | spacing <math>L</math> within a bigger capacitor of spacing <math>L_0</math>: |
| − | + | ::[[Image:casfig7.png|thumb|30px|none|]] | |
We compute | We compute | ||
<math>W_T = W(L) + W(L_0-L)</math>, and drop terms independent of <math>L</math>. This | <math>W_T = W(L) + W(L_0-L)</math>, and drop terms independent of <math>L</math>. This | ||
| − | gives | + | gives |
| − | + | :<math>W_T = W(L) + W(L_0-L) = \frac{a^2\hbar c}{2} \left[ \left( \frac{6 L}{\pi^2\lambda^4 + \frac{6(L_0-L)}{\pi^2\lambda^4} \right) -\frac{2}{\pi\lambda^3} - \frac{2\pi^2}{720}\left(\frac{1}{L^3}+\frac{1}{(L_0-L)^3} \right) + \cdots \right]</math> | |
| + | where the first term in parentheses <math>(\cdot)</math> is independent of <math>L</math>. | ||
The leading term gives the Casimir potential, | The leading term gives the Casimir potential, | ||
Revision as of 14:38, 27 April 2009
Contents
Semiclassical derivation of the Casimir force
Today, we will look at a completely different derivation of the Casimir force, based on zero-point fluctuations. Photon exchange and zero-point fluctuations are two equivalent ways of deriving Casimir forces. We will discuss this again at the end of this section.
Here we show that the Casimir force can be understand from the spectrum of classical fields between two conducting plates. Once we have all the electromagnetic modes, we can multiply each by then sum to get the zero point energy of the field. We will follow a derivation given by Haroche (Les Houches summer school), and for details of the modes between two parallel plates we refer to Jackson.
Counting modes between parallel plates
Consider two parallel metallic plates, separated by distance . Due to the boundary condition that the electric field must be zero at and , where describes the coordinate perpendicular to the plates. We have a discrete set of mode which can be distinguished as TE and TM modes. Their vector potentials are:
Parallel to the plates, we have propagating modes which can assume any wavevector components for free space propagation in two dimensions.
We want to count these modes, and obtain the density of states. Consider the number of modes with momentum between and . In a waveguide, the total wavenumber is . Thus, for each standing wave, there is a continuum, with wavenumber starting at the base value given by the standing wave, and increasing continuously from there. The number of modes between k and k+dk for a given m and k is
Density of modes
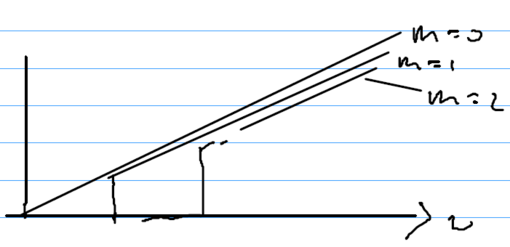
For a given m, the density of modes is linear in . For the total density of modes, we have to sum up all these linear mode densities:
The sum of those linear curves results in a trapezoid, approximating the parabolic density of modes in 3D. It is exactly the difference between the trapezoidal and parabolic densities of modes, which gives rise to the Casimir energy.
More analytically, we find, for a given , we may have an integer , such that the density of modes is
where is the Heaviside step function.
Total zero point energy
We can now use these expressions to get the total zero point energy in volume . We get
High frequency cutoff
The integrand diverges, which is unphysical. We have to introduce a high frequency cutoff needs. Since we will compare the effect of two different boundary conditions on the zero point energy, we will be subtracting two integrals, and most of the divergent parts of the integrals will cancel anyway. Physically, we can argue that the metallic boundary condition is no longer valid for X rays when the plates become transparent, and for any real material we have such a cutoff anyway.
Instead of a hard cutoff, we multiply the integrand by a convergence term , and find
with
and
Finally,
This can be expanded about , where , corresponding to letting , i.e. the limit of infinite cutoff frequency:
and
Comparison of two capacitors
We want to compare mode densities, so let us embed our capacitor of spacing within a bigger capacitor of spacing :
We compute , and drop terms independent of . This gives
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle W_T = W(L) + W(L_0-L) = \frac{a^2\hbar c}{2} \left[ \left( \frac{6 L}{\pi^2\lambda^4 + \frac{6(L_0-L)}{\pi^2\lambda^4} \right) -\frac{2}{\pi\lambda^3} - \frac{2\pi^2}{720}\left(\frac{1}{L^3}+\frac{1}{(L_0-L)^3} \right) + \cdots \right]}
where the first term in parentheses is independent of .
The leading term gives the Casimir potential,
The Casimir force, given as a pressure between the two plates, is thus
Other configurations
Such a derivation can also be done for models other than two metallic plates, but it can be mathematically quite tricky for dielectric materials. You can also derive in the same way the Casimir potential for a metal plate interacting with a single atom. This is done by recognizing that the atom is a dielectric medium, which modifies the field density near the metal plate, and therefore the mode spectrum. Similarly, for the atom-atom interaction, the two atoms can be put inside a large box; the two atoms act as tiny dielectric media, which change the field density in the box, also leading to a Casimir potential.
Interpretation of the Casimir Potential
How to interpret the Casimir potential has become an important question recently because of the realization that the origin of dark matter may arise from the physical reality of zero point energy. Such zero point energy could conceivably give rise to a gravitational force. However, the numerical value of the potential strength obtained is hundreds of orders of magnitude off from what would be needed to be consistent with the observed value of the cosmological constant.
Let us compare the two derivations we've seen. One way was to use two metallic plates and to count the number of modes in between them. Another way was to consider all the atoms in two parallel walls, and allow the atoms to exchange virtual photons. Robert Jaffe has written a beautiful treatise (Phys. Rev. D) discussing these two viewpoints, and shows these are equivalent, and that one does not even need, in principle, the concept of zero point energies. The conclusion is that the observation of the Casimir force does not prove the physical existence of zero point energies.
Note that the Casimir force (between two metallic plates) is independent of atomic properties. This is the limit in which the fine structure constant is very large. In the opposite limit , the atomic radius , in which case the Casimir force also goes to zero. Thus, by assuming our material to be metallic, we are assuming that the fine structure constant is large. What does large mean? The paper by R. Jaffe states that for distances as small as m, it is sufficient for to be able to well approximate the resulting force as being independent of atomic properties.





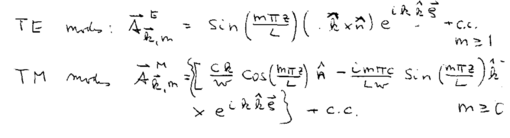







![{\displaystyle {\begin{aligned}\rho (w)\,dw&={\frac {a^{2}}{2\pi c^{2}}}\left[1+2\left\lceil {\frac {wL}{\pi c}}\right\rceil \right]w\,dw\\&={\frac {a^{2}}{2\pi c^{2}}}\left[1+2\sum _{m=1}^{\infty }\Theta \left(w-{\frac {m\pi c}{L}}\right)\right]w\,dw\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1fa1e23f8dafb6374548f0ab0b46dce561043857)


![{\displaystyle {\begin{aligned}W(L)&=\int _{0}^{\infty }{\frac {\hbar \omega }{2}}\rho (\omega )d\omega \\&={\frac {a^{2}\hbar }{4\pi c^{2}}}\left[{\int _{0}^{\infty }\omega ^{2}d\omega +2\sum _{m=1}^{\infty }\int _{m\pi c/L}^{\infty }\omega ^{2}d\omega }\right]\,.\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/fdac3bf637c4c48f14709650136e42756e5526a5)



![{\displaystyle I_{m}=c^{2}{\frac {\partial ^{2}}{\partial \lambda ^{2}}}\int _{\frac {m\pi c}{L}}^{\infty }w^{2}e^{-\lambda w/c}\,dw=c^{3}{\frac {\partial ^{2}}{\partial \lambda ^{2}}}\left[-{\frac {e^{-m\pi \lambda /L}}{\lambda }}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1d9bfb76d755acd3db0babbb3553ae73bc25fd51)
![{\displaystyle {\begin{array}{rcl}\sum _{m}I_{m}={\frac {c^{3}\pi }{L}}{\frac {\partial ^{2}}{\partial \lambda ^{2}}}\left[{{\frac {1}{(\pi \lambda /L)}}{\frac {1}{e^{\pi \Lambda /L}-1}}}\right]\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1028f463088a7c7c3c4378e7cd45286d958f80fe)




![{\displaystyle W(L)=W_{0}{\frac {a^{2}\hbar c}{2}}\left[{\frac {6L}{\pi ^{2}\lambda ^{4}}}-{\frac {1}{\pi \lambda ^{3}}}-{\frac {2\pi ^{2}}{720L^{3}}}+\cdots \right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/bbb3b40f8376635ebb60238e7ac49f011c8a0373)










