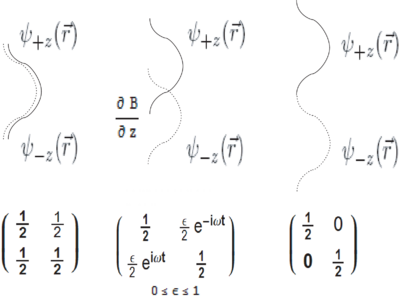imported>Rouge |
imported>Rouge |
| Line 191: |
Line 191: |
| | 475 keV/atom; H, n=3, and H, n=4 at 133 keV/atom. \cite{Andra1970}} | | 475 keV/atom; H, n=3, and H, n=4 at 133 keV/atom. \cite{Andra1970}} |
| | | | |
| − | == Clarification on coherence and dipole moment == | + | == Notes == |
| − | | |
| − | Consider the coherence of the atom after coherent excitation with a short pulse (shorter than emission rate). Let the state of the atom be <math>|\psi> = C_g |g> + C_e |e></math>.
| |
| − | Then, the coherence between <math>|e></math> and <math>|g></math> is maximum for <math>C_ e=C_ g=\frac{1}{\sqrt {2}}</math>, i.e. with <math>\frac{\pi }{2}</math> pulse. (Coherence is <math>\rho_{ab}</math> in the density matrix. For a pure state, it is <math>C_g C_e</math>). Now, let us consider a system with the atom and (external) EM mode. We consider the case where there is only a single EM mode coupled to the atom (ex, an atom strongly coupled to a cavity). Then, emission couples atomic states with photon number states: <math>|g,1></math> and <math>|e,0></math>. Thus, a <math>\frac{\pi }{2}</math> pulse also maximizes the coherence <math>|g,1></math> and <math>|e,0></math>.
| |
| − | | |
| − | On the other hand, for continuous excitation (not a short pulse), saturation of the atom leads to emission of increasingly incoherent light (Mollow triplet). For Mollow triplet, see Cohen-Tannoudji p:424.
| |
| − | | |
| − | Next, consider a coherent light which is very weak. Monochromatic, coherent light is represented by a coherent state<math>|\alpha ></math> that has a Poissonian distribution of photon numbers: <math>|\alpha >=e^{-\frac{1}{2}|\alpha|^{2}} \sum_{n = 0}^{\infty } \frac{ \alpha^{n}}{\sqrt{n+1}} |n></math>. For <math>|\alpha | \ll 1 </math>, the population of the states with <math>n > 1</math> is negligible, and the atom prepared in a state <math>|g>+\epsilon |e></math> with <math>|\epsilon | \ll 1</math> emits a coherent state of light, in agreement with what is expected for small saturation.
| |
| − | | |
| − | == First observation of coherent population trapping CPT ==
| |
| − | | |
| − | Prepare a multimode laser with regular frequency spacing (<math>v_1, \cdots</math>, in the figure).
| |
| − | | |
| − | [[Image:Fig_CPT_convert_20100416042234.jpg]]
| |
| − | | |
| − | Prepare a gas in a cylindrical volume with gradient of magnetic field in z direction, and observe fluorescence.
| |
| − | | |
| − | Dark regions show the phaces where Zeeman shift between magnetic sublevels equals frequency difference between laser modes.
| |
| − | | |
| − | == Absorption calculation by interference, goin without inversion ==
| |
| − | | |
| − | (Steve Harris, PRL 62, 1033 (1989))
| |
| − | http://prl.aps.org/abstract/PRL/v62/i9/p1033_1
| |
| − | | |
| − | It is commonly believed that we need <math>N_e > N_ g</math> for optical gain. But: Consider a V system with two unstable states that decay by coupling to the same continuum (This is a fairly special situation, e.g. different m-levels do not qualify, since they emit photons of different polarizations, thus the continue <math>|k_1></math> or <math>|k_2></math> are distinguishable.)
| |
| − | | |
| − | [[Image:Fig_darkresonance_convert_20100416034152.jpg]]
| |
| − | | |
| − | Consider three level systems as in the figure where <math>|e_1></math> and <math>|e_2></math> decay to the continuum. A surprising feature of this system is the fact that there is a frequency at which the absorption rate becomes zero. To formally confirm this, we need to compute the second-order matrix element <math>M = \sum_{i =1 ,2} \frac{<continuum| V | e_i ><e_i | V |g> }{ \Delta_i - i \frac{\Gamma_i}{2}}</math>. Then, we know that there is a frequency at which this matrix element almost cancels. Let the frequency be <math>\omega _ o</math> that corresponds to an energy between the two levels. Note <math>\omega _ o</math> depends on the two matrix elements and we assume <math>(|\Delta_1| , |\Delta_2| \gg r_1, r_2</math>.
| |
| − | | |
| − | One may understand this by considering the fact that the two-photon scattering process <math>|g>\rightarrow |continuum></math> can proceed via two pathways that are fundamentally indistinguishable. In other words, it is impossible to tell whether it came from <math>|e_1></math> or <math>|e_2></math>. Thus, we need to consider quantum interference between them.
| |
| − | | |
| − | Now assume that with some mechanism we populate, say, <math>|e_2></math> with a small number of atoms <math>N_2<N_ g</math>. These atoms have maximum stimulated emission probability on resonance, <math>|e_2>\rightarrow |g></math>, but there is also even larger absorption, since <math>N_ g>N_2</math>. However, because of the finite linewidth <math>r_2</math> of level <math>|e_2></math>, there is also stimulated emission gain at the "magical" (absorption-free) frequency <math>\omega _ o</math>. Since the <math>N_ g</math> atoms do not absorb here, there is net gain at this frequency in spite of <math>N_2<N_ g</math>, which can lead to "lasing without inversion." Note: this only works if the two excited states decay to the same continuum, such that the paths are indistinguishable. How can a system for lasing without inversion be realized?
| |
| − | | |
| − | Possibility 1: hydrogen and dc electric field
| |
| − | | |
| − | [[Image:Fig_examplessssss_convert_20100416034611.jpg]]
| |
| − | | |
| − | Possibility 2: use ac electric field to mix non-degenerate s state with p state.
| |
| − | | |
| − | == Electromagnetically induced transparency ==
| |
| − | | |
| − | "Is it possible to send a laser beam through a brick wall?"
| |
| − | | |
| − | Radio Yerevan: "In principle yes, but you need another very powerful laser..."
| |
| − | | |
| − | Steve Harris thought initially of special, ionizing excited states. However it is possible to realize the requirement of identical decay paths in a <math>\lambda</math>-system with a a(strong) coupling laser. The phenomenon is closely related to coherent population trapping.
| |
| − | | |
| − | [[Image:Fig_EIT1_convert_20100416040614.jpg]]
| |
| − | | |
| − | For resonant fields <math>\omega _1=\omega _{ge}, \omega_2=\omega _{fe}</math>, we have
| |
| − | | |
| − | [[Image:Fig_EIT2_convert_20100416040633.jpg]]
| |
| − | | |
| − | As we turn up the power of the coupling laser the transmission improves and then broadens (in the realistic case of a finite decoherence rate <math>\gamma_{gf}=0</math>, an infinitesimally small coupling Rabi frequency, but the frequency window over which transmission occurs is very narrow and given by <math>\Delta \omega =\Omega_c</math>.
| |
| − | | |
| | | | |
| | [[Category:8.421]] | | [[Category:8.421]] |
We speak of coherence if there exist well defined phases between two or more
amplitudes that can interfere. These can be, e.g., the relative phase of the
electric field in two arms of an interferometer, the relative phase of two or
more states within one atom, or the relative phase of oscillating dipole moments
in different atoms. Coherence is often a measurement tool in that the relative
phase is the time integral over the energy difference between the states. For
instance, if the atomic states have different magnetic moments, coherence
between them provides a very sensitive measurement tool for magnetic field, or
if the states have different spatial wavefunctions, the relative phase is a
sensitive measure of the gravitational energy difference. In atomic clocks,
coherence allows one to transform as energy difference between two internal
atomic states into a frequency and time standard.
Coherence in two-level systems
A two-level atom that has been prepared by a  pulse in a
superposition
pulse in a
superposition  can be viewed as
exhibiting coherence, since the phase between
can be viewed as
exhibiting coherence, since the phase between  and
and  is
well defined, and the system will evolve coherently as
is
well defined, and the system will evolve coherently as
 for times
for times
 , where
, where  is the decay rate constant for the
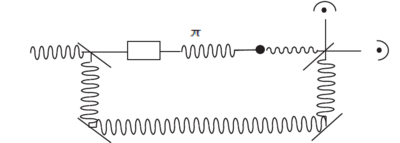
excited state. Figure \ref{fig:single-atom-coherence} displays a conceptual
experiment that can be used to test this.
\begin{figure}
\centering
is the decay rate constant for the
excited state. Figure \ref{fig:single-atom-coherence} displays a conceptual
experiment that can be used to test this.
\begin{figure}
\centering
\caption{Measurement of definite phase for light emitted by a two-level atom
prepared with a  pulse. The laser light is sent through both arms of an
interferometer; a switch in one arm selects a
pulse. The laser light is sent through both arms of an
interferometer; a switch in one arm selects a  pulse of light from the
laser with which to excite the atom. The light the atomic dipole emits is then
mixed with the other interferometer arm at the output. Averaging the output
signal over many repetitions of the experiment, the interferometer measures a
definite phase for the light emitted by the atom, defined relative to the phase
of the exciting
pulse of light from the
laser with which to excite the atom. The light the atomic dipole emits is then
mixed with the other interferometer arm at the output. Averaging the output
signal over many repetitions of the experiment, the interferometer measures a
definite phase for the light emitted by the atom, defined relative to the phase
of the exciting  pulse.}
pulse.}
\end{figure}
What about an atom prepared by a  pulse in
pulse in  ? There is no
coherence at
? There is no
coherence at  , since the atom is in a single state, but what about
, since the atom is in a single state, but what about
 ? Then the atom is in a superposition of states
? Then the atom is in a superposition of states
 . Obviously some phase must
exist, because otherwise no dipole moment
. Obviously some phase must
exist, because otherwise no dipole moment  exists that can emit (
exists that can emit ( ), but the phase
), but the phase  is completely
unpredictable, so the experiment pictured above (or an emsemble average, as in
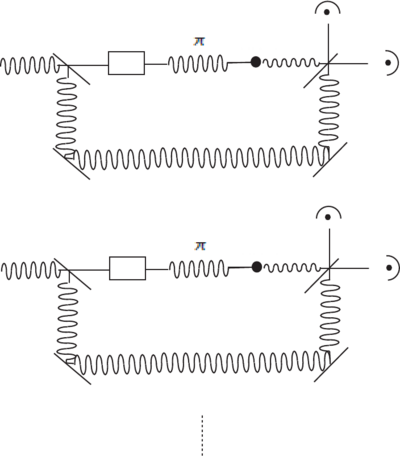
Figure \ref{fig:ensemble-average-coherence}) would yield no definite phase. We
conclude that an atom prepared in
is completely
unpredictable, so the experiment pictured above (or an emsemble average, as in
Figure \ref{fig:ensemble-average-coherence}) would yield no definite phase. We
conclude that an atom prepared in  does not exhibit coherence.
\begin{figure}
\centering
does not exhibit coherence.
\begin{figure}
\centering
\caption{Ensemble average of the phase measurement.}
\end{figure}
The ensemble average (parallel setups) or time average (repeated experiment at
same location) yields no definite phase, so we conclude that the expectation
value of the dipole moment  is zero at all times. What
is the origin of uncertain phase
is zero at all times. What
is the origin of uncertain phase  ? Vacuum fluctuations. What then
happens if we place two atoms close together and excite them at the same time?
Is the relative phase of the evolving dipole moments fixed or uncertain? If the
relative phase is fixed, how close must the atoms be for the relative phase to
be well defined? These questions about spatial coherence and Dicke superradiance
will be covered later in the chapter.
? Vacuum fluctuations. What then
happens if we place two atoms close together and excite them at the same time?
Is the relative phase of the evolving dipole moments fixed or uncertain? If the
relative phase is fixed, how close must the atoms be for the relative phase to
be well defined? These questions about spatial coherence and Dicke superradiance
will be covered later in the chapter.
Precession of a spin in a magnetic field
Precession of a spin can be viewed as an effect of coherence since
 . In a magnetic field
. In a magnetic field
 ,
so the precession is due to a coherence between the
,
so the precession is due to a coherence between the  components
of the spin. If no coherence existed, the spin would be in a statistical
mixture of
components
of the spin. If no coherence existed, the spin would be in a statistical
mixture of  and
and  .
In the density matrix formalism,
.
In the density matrix formalism,

in the z basis  .\\The expectation value of
.\\The expectation value of  ,
,
![{\displaystyle \langle {\hat {\sigma }}_{x}\rangle =Tr[\rho {\hat {\sigma }}_{x}]=Tr\left({\begin{array}{cc}{\frac {1}{2}}e^{-i\omega _{L}t}&{\frac {1}{2}}\\{\frac {1}{2}}&{\frac {1}{2}}e^{i\omega _{L}t}\end{array}}\right)=\cos \omega _{L}t.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2a9d0aea923dce7dc4a7623215831fbc0af38ef5)
If the coherences (off-diagonal elements of  ) were smaller,
) were smaller,  would be smaller. For a statistical mixture of
would be smaller. For a statistical mixture of  and
and  ,
,  and
and  .
.
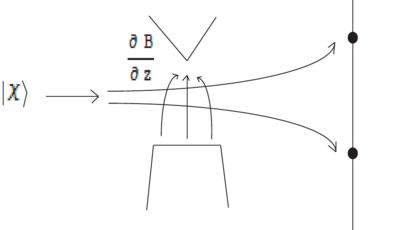
The Stern-Gerlach experiment and spatial loss of coherence
\begin{figure}
\centering
\caption{Stern-Gerlach experiment. Where in the magnet (or outside) does the projection onto  occur?}
\end{figure}
In the Stern-Gerlach experiment a particle initially spin-polarized along
occur?}
\end{figure}
In the Stern-Gerlach experiment a particle initially spin-polarized along
 has equal probability of following either the
has equal probability of following either the  trajectory or the
trajectory or the  trajectory.
So initially the particle is described by a density matrix for a pure state,
trajectory.
So initially the particle is described by a density matrix for a pure state,

after passing the Stern-Gerlach apparatus (inhomogeneous magnetic field) the
density matrix is

with no intereference possible between the two states.
Why? Because describing the full quantum state of the particle also requires
acocunting for its spatial wavefunction. The density matrix above does not
contain all the relevant degrees of freedom. Correctly, the particle should
initially be described by

with  ,
i.e. a spatial wavefunction independent of internal state,
,
i.e. a spatial wavefunction independent of internal state,
 .
In the inhomogeneous
magnetic field, the wavefuntion components
.
In the inhomogeneous
magnetic field, the wavefuntion components  evolve
differently because there is a different potential energy seen by the two spin
states
evolve
differently because there is a different potential energy seen by the two spin
states  :
:

\begin{figure}
\centering
\caption{Spatial wave function and corresponding spin density matrix in the Stern-Gerlach experiment.}
\end{figure}
The coherence (interference) between  and
and  components to
form
components to
form  exists only in the region where there is at least partial
overlap between the two wavefunctions
exists only in the region where there is at least partial
overlap between the two wavefunctions  .
When the wavefunctions do not overlap, there is no significance to a relative
phase between
.
When the wavefunctions do not overlap, there is no significance to a relative
phase between  and
and  , i.e. no interference term. (Of
course, if the wavefunctions are steered back to overlap, we can ask if there
was a well-defined relative phase between them while they were separated.)
In a more complete description, the inhomogeneous magnetic field
entangles the spatial and spin degrees of freedom. When the spatial
overlap disappears, or equivalently, when we trace over the spatial wavefunction
(by measuring the particle either at location 1 or at location 2), the
interference between
, i.e. no interference term. (Of
course, if the wavefunctions are steered back to overlap, we can ask if there
was a well-defined relative phase between them while they were separated.)
In a more complete description, the inhomogeneous magnetic field
entangles the spatial and spin degrees of freedom. When the spatial
overlap disappears, or equivalently, when we trace over the spatial wavefunction
(by measuring the particle either at location 1 or at location 2), the
interference between  and
and  giving rise to
giving rise to  disappears.
In a measurement language, the inhomogeneous magnetic field entangles the
"variable" (
disappears.
In a measurement language, the inhomogeneous magnetic field entangles the
"variable" ( ) with the "meter" (the spatial wavefunctions of the
particle). Once the spatial wavefunctions
) with the "meter" (the spatial wavefunctions of the
particle). Once the spatial wavefunctions
 cease to overlap, the particle's position can serve as a "meter" for the
variable to be measured, the spin along
cease to overlap, the particle's position can serve as a "meter" for the
variable to be measured, the spin along  . However, until the particle
hits the screen, or is subjected to uncontrolled or unknown magnetic fields, the
meter-variable entanglement is still reversible, and a "measurement" has not
been made.
. However, until the particle
hits the screen, or is subjected to uncontrolled or unknown magnetic fields, the
meter-variable entanglement is still reversible, and a "measurement" has not
been made.
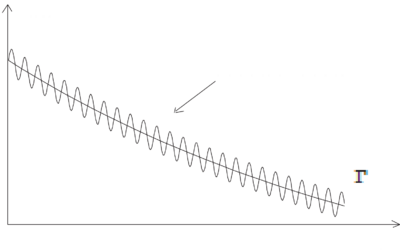
Quantum Beats
Quantum beats can be thought of as a two-level effect, though they are observed
in multilevel atoms. They allow one to measure level spacings with high
resolution when a narrowband excitation source (narrowband laser) is not
available.
\begin{figure}
\centering
\caption{Multiple levels within energy  from ground state,
from ground state,  ,\ldots}
,\ldots}
\end{figure}
Consider the scenario of Figure \ref{fig:qb-levels}, where we have multiple
excited levels in a narrow energy interval  , all decaying to a
common ground state. If we excite with a pulse of duration
, all decaying to a
common ground state. If we excite with a pulse of duration  , we cannot resolve the levels, and they will be populated
according to the coupling strength to the ground state
, we cannot resolve the levels, and they will be populated
according to the coupling strength to the ground state  for the given
excitation method:
for the given
excitation method:

and for times  ,
the state vector is
,
the state vector is

It follows that, in directions where the radiation from the levels  interferes, there will be oscillating terms at frequencies
interferes, there will be oscillating terms at frequencies  on top of the excited state decay.
\begin{figure}
\centering
on top of the excited state decay.
\begin{figure}
\centering
\caption{Quantum beats}
\end{figure}
This allows one to measure excited-state splittings in spite of the lack of a
sufficiently narrow excitation source. Compared to our initial example of a
two-level atom, here the coherence is initially purely between the excited
states (definite excitation phase between them), and no coherence between
 and
and  exists initially. Of course, as the atom
decays, coherence between
exists initially. Of course, as the atom
decays, coherence between  and
and  (e.g. dipole
moments) build up, and the coherence between the emitted fields of the different
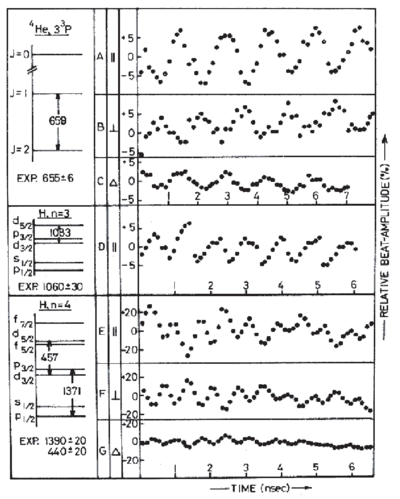
dipoles gives rise to the observed effect. Figures \ref{fig:qb-ideal} and
\ref{fig:qb-data} show respectively an idealized quantum beat signal and real
data from an experimental demonstration of the technique.
\begin{figure}
\centering
(e.g. dipole
moments) build up, and the coherence between the emitted fields of the different
dipoles gives rise to the observed effect. Figures \ref{fig:qb-ideal} and
\ref{fig:qb-data} show respectively an idealized quantum beat signal and real
data from an experimental demonstration of the technique.
\begin{figure}
\centering
\caption{Schematic level diagrams and observed quantum beats of  at
475 keV/atom; H, n=3, and H, n=4 at 133 keV/atom. \cite{Andra1970}}
at
475 keV/atom; H, n=3, and H, n=4 at 133 keV/atom. \cite{Andra1970}}
Notes

























![{\displaystyle \langle {\hat {\sigma }}_{x}\rangle =Tr[\rho {\hat {\sigma }}_{x}]=Tr\left({\begin{array}{cc}{\frac {1}{2}}e^{-i\omega _{L}t}&{\frac {1}{2}}\\{\frac {1}{2}}&{\frac {1}{2}}e^{i\omega _{L}t}\end{array}}\right)=\cos \omega _{L}t.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2a9d0aea923dce7dc4a7623215831fbc0af38ef5)