Difference between revisions of "Entangled Photons"
imported>Atsommer m (→Definition) |
imported>Ichuang |
||
| Line 375: | Line 375: | ||
reduced density matrix of one-half of an entangled bi-partite state, | reduced density matrix of one-half of an entangled bi-partite state, | ||
in the following way. | in the following way. | ||
| + | |||
| + | ==== Partial Traces ==== | ||
| + | |||
Given <math>|\psi_{AB}{\rangle}</math>, the partial trace over <math>B</math> is the density matrix | Given <math>|\psi_{AB}{\rangle}</math>, the partial trace over <math>B</math> is the density matrix | ||
:<math>\begin{array}{rcl} | :<math>\begin{array}{rcl} | ||
| Line 389: | Line 392: | ||
</math> | </math> | ||
the completely mixed state. | the completely mixed state. | ||
| + | |||
| + | ==== Von Neumann Entropy ==== | ||
| + | |||
The randomness of a quantum state <math>\rho</math> is measured by the {\em Von | The randomness of a quantum state <math>\rho</math> is measured by the {\em Von | ||
Neumann entropy}, <math>S(\rho)</math>, defined as | Neumann entropy}, <math>S(\rho)</math>, defined as | ||
| Line 402: | Line 408: | ||
Note that the entropy of a pure state is zero. For the completely | Note that the entropy of a pure state is zero. For the completely | ||
mixed state in the example above, <math>S(\rho_A) = 1</math> bit. | mixed state in the example above, <math>S(\rho_A) = 1</math> bit. | ||
| + | |||
| + | ==== The Entanglement ==== | ||
| + | |||
The Entanglement <math>E(|\psi_{AB} \rangle )</math> of a bi-partite quantum state | The Entanglement <math>E(|\psi_{AB} \rangle )</math> of a bi-partite quantum state | ||
<math>|\psi_{AB}{\rangle}</math> is defined as being the entropy of one of the reduced | <math>|\psi_{AB}{\rangle}</math> is defined as being the entropy of one of the reduced | ||
| Line 411: | Line 420: | ||
Since <math>|\psi_{AB}{\rangle}</math> is a pure state, these two entropies must be | Since <math>|\psi_{AB}{\rangle}</math> is a pure state, these two entropies must be | ||
equal. | equal. | ||
| + | |||
The Entanglement of the Bell states is the maximum possible for any | The Entanglement of the Bell states is the maximum possible for any | ||
two-qubit state: <math>E(|\Phi^\pm \rangle ) = E(|\Psi^\pm \rangle ) = 1</math>. In fact, we | two-qubit state: <math>E(|\Phi^\pm \rangle ) = E(|\Psi^\pm \rangle ) = 1</math>. In fact, we | ||
Revision as of 01:38, 27 February 2009
Entanglement is the essence of the most "quantum" nature of quantum states of light. Albert Einstein studied it, together with Boris Podolsky and Nathan Rosen, and argued that properties of entangled quantum states demonstrated that quantum mechanics could not be a complete theory of nature, because of violations of intuition. Experiments now validate such violations, and we appreciate that intuition from classical mechanics is what is at fault. Indeed, John Bell proved that correlations produced by entangled quantum states are distinctly beyond what is possible with classical mechanics. Today, we also understand that entanglement is useful for many things. Ideally, it can allow measurement with precision greater than with classical states; entanglement can also be used to increase communication rates through noisy channels, and indeed, even make it possible when noise rates are otherwise too high. Entanglement also enables exponential speedup of the solution of certain mathematical problems, through quantum computation. And, as we have seen, it allows new protocols such as teleportation; entanglement also makes possible new games, sometimes providing more optimal Nash equilibria in important multi-party economic scenarios such as the tragedy of the commons.
In this section, we define entanglement, describe some of its non-classical properties, illustrate an application of entanglement to speeding up communication, and show how pure entangled states can be purified from imperfect ones. We then conclude by describing some measures of entanglement.
Contents
Definition
A bi-partite (or, in the language of light, "two-mode") state Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi \rangle _{AB}} of a composite system is entangled if and only if there do not exist Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi \rangle _A} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi \rangle _B} such that
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi \rangle _{AB} = |\psi \rangle _A \otimes |\psi \rangle _B \,. }
Note that "Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \otimes} " denotes a tensor product. Being a composite system means that there should be a tensor product structure to the Hilbert space -- more on this point later. Some examples will help illustrate this definition:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |00 \rangle = |0{\rangle}\otimes|0{\rangle}} is unentangled
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{|00{\rangle}+|11{\rangle}}{\sqrt{2}}} is entangled (verify this for yourself!)
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{|00{\rangle}+|01{\rangle}+|10{\rangle}+|11{\rangle}}{{2}}} is unentangled, because it is equal to Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{|0{\rangle}+|1{\rangle}}{\sqrt{2}}\otimes \frac{|0{\rangle}+|1{\rangle}}{\sqrt{2}}}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{|00{\rangle}+|01{\rangle}+|11{\rangle}}{\sqrt{3}}} is what? Work this out.
A similar definition holds for mixed states. A bi-partite mixed state of a composite system is entangled if and only if there do not exist states Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \rho_{A,k}} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \rho_{B,k}} of and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle B} , and probabilities , satisfying Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \sum_k p_k = 1} , such that
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \rho_{AB} = \sum_{k} p_k \rho_{A,k} \otimes \rho_{B,k} \,. }
Mixed state entanglement is considerably more difficult to study than the pure state case, however, so we will focus our attention on developing an understanding by studying pure state entanglement.
The most basic entangled state in Nature is that of two two-dimensional systems. One such entangled state is the well known singlet state,
This state, or states with essentially the same properties, can be produced with photons. However, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi_{EPR}{\rangle}} is not just a single photon. Indeed, one photon input a beamsplitter can easily produce the state Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (|01{\rangle}-|10 \rangle )/\sqrt{2}} , as we have previously seen, but in that situation is a vacuum state, with no photons present.
The EPR state Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi_{EPR}{\rangle}} must be of {\em two physically distinct systems}. This is because the definition of entanglement specifies that entanglement is a property of a composite system, made of two (or more) parts, rather than just a single system. It is important that in principle, the two parts can be separately manipulated and measured, for entanglement to be meaningfully defined.
For example, the two electrons in the ground state of a helium atom are naturally in a singlet Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (|01{\rangle}+|10 \rangle )/\sqrt{2}} ; does this mean helium atoms are all entangled? Absolutely not, under normal circumstances. Without an experimental situation in which the two electrons in the atom can be individually manipulated, they have no meaningful entanglement.
A meaningful photon representation of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi_{EPR}{\rangle}} is given, for example, by the polarization of two photons. Two photons can be generated by down-conversion, such that their polarizations have the state
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi_{EPR} \rangle = \frac{|HV \rangle + |VH{\rangle}}{\sqrt{2}} \,, }
where and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V} indicate horizontal and vertical polarization.
Another example is two photons, each of which can exist in one of two modes, such that we may identify Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |0_L \rangle = |01{\rangle}} , and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |1_L \rangle = |10{\rangle}} . The state
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi_{EPR} \rangle = \frac{|0_L 1_L \rangle + |1_L 0_L{\rangle}}{\sqrt{2}} }
is a meaningfully entangled state, since each photon can be manipulated and measured separately.
EPR and the Bell inequality
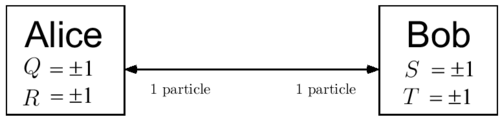
What about entanglement is uniquely quantum-mechanical? This question can be answered with the following experiment, which measures correlations produced by measurements of an EPR pair. Let there be two parties, Alice and Bob, who are each given one photon from some state Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\Psi{\rangle}} (which may be quantum or classical). Alice and Bob each simultaneously measure in random bases: Alice measures either Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q} or Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle R} , and Bob measures either or Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T} , obtaining Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \pm1} for their results:
Classical statistics places a bound on the correlation of the measurement outcomes, independent of , given the fact that the choice of measurements is random. In particular, consider the quantity Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle QS+RS+RT-QT} . Notice that
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} QS+RS+RT-QT & = & (Q+R)S +(R-Q)T \,. \end{array}} (3.1)
Because Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle R, Q = \pm 1} it follows that either Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (Q+R)S = 0} or Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (R-Q)T = 0} . In either case, it is easy to see from (3.1) that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle QS+RS+RT-QT = \pm 2} .
Suppose next that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle p(q,r,s,t)} is the probability that, before the measurements are performed, the system is in a state where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q=q, R=r, S = s} , and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T=t} . These probabilities may depend on how Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\Psi{\rangle}} is prepared, and on experimental noise. Letting denote the mean value of a quantity, we have
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} E (QS+RS+RT-QT) & = & \sum_{qrst} p(q,r,s,t) ( qs+rs+rt-qt ) \\ & \leq & \sum_{qrst} p(q,r,s,t) \times 2 \\ & = & 2. \end{array}} (3.2)
Also,
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} E (QS+RS+RT-QT) & = & \sum_{qrst} p(q,r,s,t) qs + \sum_{qrst} p(q,r,s,t) rs \\ & & + \sum_{qrst} p(q,r,s,t) rt - \sum_{qrst} p(q,r,s,t) qt \\ & = & E (QS)+ E (RS) + E (RT)- E (QT). \end{array}} (3.3)
Comparing (3.2) and (3.3) we obtain the Bell inequality,
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E (QS)+ E (RS)+ E (RT)- E (QT) \leq 2 } (3.4)
This result is also often known as the CHSH inequality after the initials of its four discoverers. It is part of a larger set of inequalities known generically as Bell inequalities, since the first was found by John Bell.
When an experiment is performed, however, a result violating this inequality is obtained for certain input states. Specifically, suppose that the state distributed to Alice and Bob is the entangled two-photon state Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\Psi \rangle = |\psi_{EPR}{\rangle}} . Also, let Alice's measurement operators be
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} Q &=& |0 \rangle \langle 0| - |1 \rangle \langle 1| \\ R &=& |0 \rangle \langle 1| + |1 \rangle \langle 0| \end{array}}
and Bob's be
Physically, if the EPR pair is represented by photon polarizations, so that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi_{EPR} \rangle = \frac{|HV \rangle + |VH{\rangle}}{\sqrt{2}}} , then Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle R} correspond to measurements of linear and circular photon polarization, while and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T} are the same measurements but only after rotating the polarization by Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 45^\circ} . In the dual-rail representation, these measurement operators correspond to four well defined configurations of beamsplitters and phase shifters, followed by photodetectors.
Denoting expectation values using Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle {\langle}\cdots \rangle = {\langle}\psi_{EPR}|\cdots|\psi_{EPR}{\rangle}} , we find that
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} \langle QS \rangle = \frac{1}{\sqrt 2}; \,\, \langle RS \rangle = \frac{1}{\sqrt 2}; \,\, \langle RT \rangle = \frac{1}{\sqrt 2}; \,\, \langle QT \rangle = -\frac{1}{\sqrt 2} \,. \end{array}}
Thus,
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} \langle QS \rangle + \langle RS \rangle + \langle RT \rangle - \langle QT \rangle = 2 \sqrt 2 \,. \end{array}}
This result is in direct contradiction with the bound established by classical statistics, Eq.(3.4), which predicts that the average value of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle QS} plus the average value of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle RS} plus the average value of minus the average value of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle QT} can never exceed two.
Yet here, quantum mechanics predicts that this sum of averages yields Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2 \sqrt 2} , and indeed, this is observed experimentally! The main implication of this result is that one of the assumptions behind the classical bound was wrong. The two most often questioned are
- The assumption that the physical properties of the state have definite values Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q, R, S, T} which exist independent of observation. This is sometimes known as the assumption of realism.
- The assumption that Alice performing her measurement does not influence the result of Bob's measurement (and vice versa). This is sometimes known as the assumption of locality.
These two together are known as the assumptions of local realism. An important lesson from experiments with EPR states is that either or both of locality and realism must be dropped from our view of the world if we are to develop a good intuitive understanding of quantum mechanics.
Superdense coding
The fact that entanglement has distinctly non-classical properties has inspired a great search to discover useful tasks which can be accomplished more efficiently using entangled states than by using just classical resources. Teleportation is one example. Another, example is superdense coding, a protocol through which Alice can communicate two bits of classical information to Bob, by sending him just one qubit, given prior shared entanglement. This works as follows. Let Alice and Bob each have half of the entangled state
This state is presumed to have been distributed to each party, long before Alice obtains the classical information she wishes to communicate to Bob. Let the information Alice wishes to send be the two bits Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k_1k_0 = \{00,01,10,11\}} . She performs one of four rotations to her local quantum state, depending on Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k_1k_0} , as follows:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} k_1k_0 = 00 &\longrightarrow& I \\ k_1k_0 = 01 &\longrightarrow& X \\ k_1k_0 = 10 &\longrightarrow& Z \\ k_1k_0 = 11 &\longrightarrow& Y \,, \end{array}}
where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle X} , Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Y} , and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Z} are the usual Pauli matrices (they happen to also be unitary transforms, and that is how they are used here). The state after Alice's operation is thus (suppressing normalization factors, and labeling states by Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |ba{\rangle}} , so Alice's state is written on the right):
Alice now sends her state to Bob, so that he ends up with both halves. Now, since these four states are mutually orthogonal, they can be distinguished from each other by projective measurements. To be explicit, it is useful to introduce a common operation and some common nomenclature used in quantum information. The two-level systems we have been working with are known as being "qubits," and as we saw in Section 2.3, using dual-rail photon representations of qubits, one qubit can be used as a control to flip another qubit (eg using a nonlinear Mach-Zehnder interferometer). Such a "controlled-{\sc not}" operation implements the following unitary transform:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} |00 \rangle &\rightarrow& |00{\rangle} \\ |01 \rangle &\rightarrow& |01{\rangle} \\ |10 \rangle &\rightarrow& |11{\rangle} \\ |11 \rangle &\rightarrow& |10{\rangle} \,. \end{array}}
Mathematically, this is easy to remember as being the map Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |x,y{\rangle} \rightarrow |x,x\oplus y{\rangle}} . The procedure Bob uses to distinguish his four measurements can then be described as being a controlled-{\sc not} operation (also known as a "{\sc cnot}"), followed by a Hadamard gate Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H} on the control qubit, which we recall performs
Specifically, for the four states, the controlled-{\sc not} gives:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} k_1k_0 = 00 &:& |00{\rangle}+|11 \rangle \longrightarrow |00{\rangle}+|10{\rangle} \\ k_1k_0 = 01 &:& |01{\rangle}+|10 \rangle \longrightarrow |01{\rangle}+|11{\rangle} \\ k_1k_0 = 10 &:& |00{\rangle}-|11 \rangle \longrightarrow |00{\rangle}-|10{\rangle} \\ k_1k_0 = 11 &:& |01{\rangle}-|10 \rangle \longrightarrow |01{\rangle}-|11{\rangle} \,. \end{array}}
Note that these four states factorize, eg Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |00{\rangle}+|10 \rangle = (|0{\rangle}+|1 \rangle )|0{\rangle}} . Bob next performs a Hadamard gate to the left qubit, obtaining
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} k_1k_0 = 00 &:& |00{\rangle}+|10 \rangle \longrightarrow |00{\rangle} \\ k_1k_0 = 01 &:& |01{\rangle}+|11 \rangle \longrightarrow |01{\rangle} \\ k_1k_0 = 10 &:& |00{\rangle}-|10 \rangle \longrightarrow |10{\rangle} \\ k_1k_0 = 11 &:& |01{\rangle}-|11 \rangle \longrightarrow |11{\rangle} \,, \end{array}}
such that a final measurement in the usual ("computational") basis returns the two classical bits which Alice sent.
Purification
Entangled states are useful. So far, however, we've mainly encountered a few particular entangled states in our applications, teleportation, and superdense coding, and in violating Bell's inequality. These are the maximally entangled states, and there are four standard ones (known as "Bell state") used as basis states:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} |\Phi^\pm \rangle &=& \frac{|00{\rangle}\pm|11{\rangle}}{\sqrt{2}} \\ |\Psi^\pm \rangle &=& \frac{|01{\rangle}\pm|10{\rangle}}{\sqrt{2}} \,. \end{array}}
On the other hand, we've noticed that there are many entangled two-qubit states. In fact, generically Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a|00 \rangle + b|11{\rangle}} is entangled for all non-trivial values of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} . Going even further, it turns out most multi-partite states, drawn randomly, are entangled! In a moment, we will try to quantify how entangled each are. But first, it is useful to motivate this quest by showing that in fact, arbitrary entangled qubit pairs can be turned into a standard Bell state, at some cost. Suppose that Alice and Bob have a large supply of poorly entangled states
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi \rangle = a|00 \rangle + b|11{\rangle} \,, }
with some unknown (but constant) value of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} . They may turn these into useful Bell pairs by doing the following. Take two poor entangled states,
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} |\psi{\rangle}\otimes |\psi{\rangle} &=& \left[ { a|00{\rangle}+b|11 \rangle } \right] \left[ { a|00{\rangle}+b|11 \rangle } \right] \\ &=& a|00 \rangle \left[ { a|00{\rangle}+b|11 \rangle } \right] + b|11 \rangle \left[ { a|00{\rangle}+b|11 \rangle } \right] \,. \end{array}}
Now Alice and Bob each perform a controlled-{\sc not} operation on the two qubits they locally possess. This transforms Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |1100{\rangle}\rightarrow |1111{\rangle}} , and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |1111{\rangle}\rightarrow |1100{\rangle}} , and leaves all other states unchanged, giving a new state
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} a|00 \rangle \left[ { a|00{\rangle}+b|11 \rangle } \right] + b|11 \rangle \left[ { a|11{\rangle}+b|00 \rangle } \right] = \left[ { a^2|00 \rangle + b^2|11 \rangle } \right] |00 \rangle + ab \left[ { |00 \rangle +|11 \rangle } \right] |11{\rangle} \,. \end{array}}
Now Alice and Bob each measure the qubits in the target pair. With probability , they will obtain Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 11} as their result, in which case the post-measurement state is , a perfect Bell pair. Otherwise, if they obtain , they throw away the result and start again. This protocol allows Alice and Bob to purify Bell states from poorly entangled states, albeit rather inefficiently. Efficient procedures do exist, and they can be read about in the literature (see, for example, Section 12.5 of {\em Quantum Computation and Quantum Information}, by Nielsen and Chuang).
Measures
There are several good measures of how entangled a pure state is. The basic idea is that entangled qubits are correlated, so that the individual qubits of an entangled pair are highly random. Moreover, the more entangled a pair is, the more random the separated qubits should be.
This randomness can be measured by computing the entropy of the reduced density matrix of one-half of an entangled bi-partite state, in the following way.
Partial Traces
Given , the partial trace over Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle B} is the density matrix
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} \rho_A &=& {\rm Tr}_B |\psi_{AB}{\rangle}{\langle}\psi_{AB}| \\ &=& \sum_k \,_B \langle k|\psi_{AB}{\rangle}{\langle}\psi_{AB}|k \rangle _B \,. \end{array}}
Similarly, is the partial trace over Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle A} . For example, for Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\Phi^+{\rangle}} ,
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \rho_A = \rho_B = \frac{1}{2} \left[ \begin{array}{cc}{1}&{0}\\{0}&{1}\end{array}\right] \,, }
the completely mixed state.
Von Neumann Entropy
The randomness of a quantum state is measured by the {\em Von Neumann entropy}, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S(\rho)} , defined as
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} S(\rho) &=& -{\rm Tr}(\rho \log_2 \rho) \\ &=& -\sum_k \lambda_k \log_2 \lambda_k \,, \end{array}}
where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda_k} are the eigenvalues of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \rho} . Note that this entropy is measured in units of bits, since the logs are taken to have base . Note that the entropy of a pure state is zero. For the completely mixed state in the example above, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S(\rho_A) = 1} bit.
The Entanglement
The Entanglement Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E(|\psi_{AB} \rangle )} of a bi-partite quantum state Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi_{AB}{\rangle}} is defined as being the entropy of one of the reduced density matrices:
Since Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi_{AB}{\rangle}} is a pure state, these two entropies must be equal.
The Entanglement of the Bell states is the maximum possible for any two-qubit state: Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E(|\Phi^\pm \rangle ) = E(|\Psi^\pm \rangle ) = 1} . In fact, we use the Bell states to define the unit of entanglement, the {\em ebit}. Recall from the last subsection that less-entangled quantum states can be purified to produce pure ebits. In fact, the Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E(|\psi \rangle )} turns out to be the rate at which can be purified to become ebits. Entanglement is thus fungible, and can act much like a currency, useful for a variety of applications, and convertible into various denominations. It truly is thus a physical resource.