Difference between revisions of "Non-classical states of light"
imported>Wikipost m |
imported>Ichuang |
||
| Line 1: | Line 1: | ||
| − | |||
== Non-classical light == | == Non-classical light == | ||
| Line 255: | Line 254: | ||
a_0 &=& U_{bs} a U_{bs}^\dagger = \frac{a+b}{\sqrt{2}} | a_0 &=& U_{bs} a U_{bs}^\dagger = \frac{a+b}{\sqrt{2}} | ||
\\ | \\ | ||
| − | a_0 &=& U_{bs} a U_{bs}^\ | + | a_0 &=& U_{bs} a U_{bs}^\dagg |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Revision as of 14:41, 18 March 2007
Contents
Non-classical light
One of the most special properties of the coherent state is that its fluctuations in Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle P} and are equal, and minimal, meaning that it satisfies the Heisenberg limit . However, satisfying this limit does not require equal noise in the two conjugate variables; it is certainly permissible for noise in one to be larger than the other. Such states can, in principle, be quite useful, for example, if a measurement only involves , and excess noise in can be disregarded. In this section, we define such squeezed states, and explore their physical properties. We begin with classical squeezing, then define squeezed states of a quantum simple harmonic oscillator, describe how squeezing can be experimentally detected, then show how it can be useful in a quantum experiment: teleportation.
Classical squeezing
We are interested in the dynamics of the simple harmonic oscillator, and it is useful to obtain some intuition about what is possible from considering the classical oscillator. Consider a particle in the potential
The equations of motion for this particle, have the solution
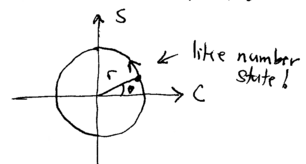
where , , and are constant. This motion is circular:
Suppose a small, "parametric" driving force is added, analogous to kicking while on a swing. For a swinging pendulum, this force could be applied by tugging gently up and down on the string as the pendulum oscillates back and forth. Let this force be applied at twice the frequency of the natural harmonic motion, such that the potential becomes
where is small. substituting Eq.(\ref{eq:c2-ansatz}) into the equation of motion
approximating , and averaging away terms rapidly oscillating at , we obtain new approximate equations of motion
with solutions
This shows that the circular motion in phase space evolves under the parametric drive to become elliptical:
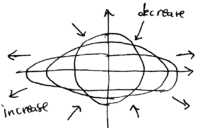
What is the ultimate limit of this classical squeezing effect? The answer turns out to be quantum noise, which enforces the Heisenberg uncertainty limit, .
Squeezed states: quantum
A quantum simple harmonic oscillator excited by a parametric drive at will also generate squeezed states. Physically, such a process corresponds to a "nonlinear" interaction which involves photons interacting with each other, via the medium they are transported through or generated from. One important physical process that generates squeezed states of light is known as the optical parametric oscillator, which we may think of as being an atomic system that is driven at , and produces two photons at and , due to cascaded decay from two equaly spaced energy levels:
Such a process can be described by the Hamiltonian
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H = aa b^\dagger + a^\dagger a^\dagger b \,, }
where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} acts on the output mode at frequency , and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} the input mode, at frequency Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2\omega_0} . If the input light is a strong coherent field, then to a good approximation, we may replace Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b^\dagger } by a classical variable in this Hamiltonian, obtaining
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H = \frac{r}{2} \left( { e^{-i\phi} a^2 + e^{i\phi} { a^\dagger }^2 } \right) \,, }
where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle r} denotes the strength of the input pump light, and for simplicity, we fix Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \phi} in the following. Motivated by this Hamiltonian for the optical parametric oscillator, we may define a mathematical operator which produces squeezed states:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S(r) = \exp \left[ { -\frac{r}{2}(a^2 - { a^\dagger }^2) } \right] \,. }
Note that the operator in the exponent has the form , where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle A} is Hermitian, so Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S(r) = \exp[iA]} is manifestly a unitary transform. What does Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S(r)} do? A useful mathematical technique for dealing with Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S(r)} is to understand how it transforms operators in the Heisenberg picture. For example,
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} S(r) x S(r)^\dagger &=& e^{iA} x e^{-iA} \\ &=& x + [iA,x] + \frac{[iA,[iA,x]]}{2!} + \cdots \,, \end{array}}
Let us use
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x = \frac{a+ a^\dagger }{2} ~~~~~~~ p = \frac{a- a^\dagger }{\sqrt{2i}} \,, }
as dimensionless Hermitean operators for position and momentum. Under the squeezing operator, using the above expansion, it is straightforward to show that position and momentum transform to become:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} S(r) x S(r)^\dagger &=& x e^r \\ S(r) p S(r)^\dagger &=& p e^{-r} \,. \end{array}}
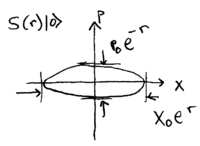
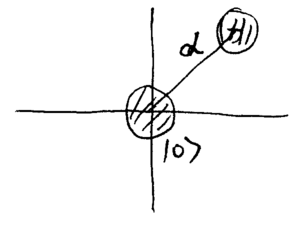
This shows that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S(r)} squeezes noise from the position, and adds noise to the momentum, of a harmonic oscillator state. Consider, for example, the effect of this operator on the vauum state, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S(r)|0{\rangle}} , which we call the "squeezed vacuum" state. The Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q(\alpha)} plot of this squeezed vacuum is:
\noindent Note that since Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S(r)} is unitary, it leaves Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta x\,\Delta p} invariant, so Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S(r)|0{\rangle}} is a minumim uncertainty state, since Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |0{\rangle}} is. We can explicitly compute what Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S(r)|0{\rangle}} is as follows:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} S(r)|0 \rangle &=& \sum_{k=0}^\infty \frac{1}{k!} \left[ { -\frac{r}{2} (a^2-{ a^\dagger }^2)} \right] ^k |0{\rangle} \\ &=& \frac{1}{\sqrt{\cosh r}} \sum_{n=0}^\infty \frac{\sqrt{(2n)!}}{2^n n!} (\tanh r)^n |2n{\rangle} \,. \end{array}}
Note that this state only has nonzero probability amplitude to have an even number of photons! Another usful representation for the squeezed vacuum is in terms of coherent states:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S(r)|0 \rangle = \frac{1}{\sqrt{\pi}} \frac{e^{r/2}}{\sqrt{e^{2r}-1}} \int_{-\infty}^{\infty} d\alpha\; e^{-\frac{\alpha^2}{e^{2r}-1}}|\alpha{\rangle} \,. }
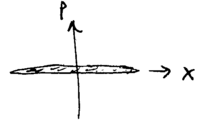
This representation allows us to depict useful limits, such as when , the inifinite squeezing limit, giving a state we may call Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |p=0{\rangle}} :
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |p=0 \rangle = \lim_{r\rightarrow\infty} S(r)|0{\rangle} \propto \int_{-\infty}^\infty d\alpha\; |\alpha{\rangle} \,, }
and similarly, we may define
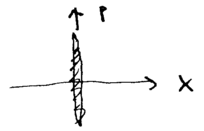
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |x=0{\rangle} \propto \int_{-\infty}^\infty d\alpha\; |i\alpha{\rangle} \,. }
These two states have Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q(\alpha)} plots which are of infinite extent, horizontally (for Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle p} ) and vertically (for Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} ), indicating that infinitely squeezed states are position and momentum eigenstates:
How can squeezing produce finite valued position and momentum eigenstates? Physically, a simple harmonic oscillator such as an oscillating pendulum is given a finite momentum or position by displacing the pendulum, and the same is done to produce squeezed states of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \langle p{\rangle}\neq 0} and . We define the mathematical displacement operator Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D(\alpha)} as
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D(\alpha) = \exp \left[ { \alpha a^\dagger - \alpha^* a } \right] \,. }
It is straightforward to show (by expanding the exponential, for example), that this operator has the property that
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} D(\alpha)a D(\alpha)^\dagger &=& a-\alpha \\ D(\alpha)^\dagger a D(\alpha) &=& a+\alpha \,, \end{array}}
such that the displaced vacuum state satisfies
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{array}{rcl} a D(\alpha)|0 \rangle &=& D(\alpha) D(\alpha) ^\dagger a D(\alpha) |0{\rangle} \\ &=& D(\alpha) (a+\alpha) |0{\rangle} \\ &=& \alpha D(\alpha) |0{\rangle} \,. \end{array}}
Since is thus an eigenstate of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} , it must be the case that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D(\alpha) |0 \rangle = |\alpha{\rangle}} . The displacement operator displaces the vacuum state Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |0{\rangle}} to become a coherent state Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\alpha{\rangle}} :
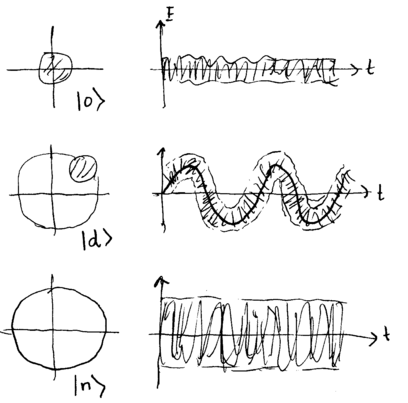
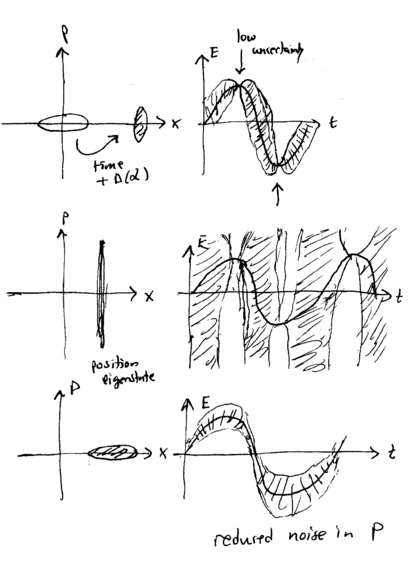
Using the displacement operator, squeezed states with finite momentum and position can thus be described, for example, by . What do these states look like, both in the Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q(\alpha)} representation, as well as their electric field? Recall that the vacuum, coherent state, and number states look as follows:
A displaced, partially squeezed state, a near-position eigenstate, and a near-momentum eigenstate correspondingly look like this:
Note how the squeezed states attain electric fields which are, at times, very low in either amplitude or phase uncertainty. By employing these states in the right kind of interferometer, as we shall see later, this reduced noise level can be used to improve the precision of certain measurements.
Homodyne detection
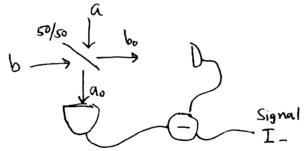
How can we experimentally detect if a state is squeezed? Ideally, squeezing could be detected by measuring the noise level in Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle p} . However, photodetectors are square-law detectors which sense light intensity, meaning photon number, and not quadrature field components. Nevertheless, the Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} and field components can be detected if the input light is first transformed by beating it with a reference signal of fixed phase. Intuitively, this follows the principle upon which many early radios worked: by mixing a signal Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V(t) = c(t) \cos \omega_0 t + s(t) \sin\omega_0 t} with a reference oscillator Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle B(t) = B_0 \cos(\omega_0 t+\delta)} , the cosine and sine components and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle s(t)} can be picked out. This method is known as homodyne detection, because it involves mixing with a reference at the same frequency as the signal. With radio frequency signals, a diode is used to mix signal and reference; the nonlinearity of the diode produces an output which is to first order, the product of the signal and reference. Mixing of light frequency signals is done with a beamsplitter. Consider a 50/50 beamsplitter with the signal input into port Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} , and the reference into port Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} , and detectors placed at the two outputs:
The beamsplitter mixes the two input ports, performing a unitary transform Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle U_{bs}} that relates the output port operators Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a_0} and to the input port operators Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} ,
- <math>\begin{array}{rcl}
a_0 &=& U_{bs} a U_{bs}^\dagger = \frac{a+b}{\sqrt{2}} \\ a_0 &=& U_{bs} a U_{bs}^\dagg





![{\displaystyle {\begin{array}{rcl}x(t)&=&r(t)\cos \left[{\omega _{0}t-\theta (t)}\right]\\&=&c(t)\cos \omega _{0}t+s(t)\sin \omega _{0}t\,,\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ed1126f0314b124b847a7002315b85c3ebe46531)



![{\displaystyle V(x)={\frac {1}{2}}m\omega _{0}^{2}x^{2}\left[{1+\epsilon \sin 2\omega _{0}t}\right]\,,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/edff86a2e3d12726d0213449d5ae7228eefca2d0)

![{\displaystyle {\ddot {x}}=-\omega _{0}^{2}\left[{1+\epsilon \sin 2\omega _{0}t}\right]x(t)\,,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c1e3676b6d9d447947628c0faa1a8c06be12dca6)