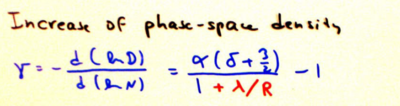Difference between revisions of "Techniques for cooling to ultralow temperatures"
imported>Zakven |
imported>Zakven (→Speed of Evaporation: Continuing to write the evaporation section) |
||
| Line 327: | Line 327: | ||
Where we have defined <math>\tau_{ev}=1/(n_0 \sigma \bar{v} \eta e^{-\eta}) </math>. This expression describes the rate at which atoms with energy greater than <math>\eta kT</math> are scattered out of that region of the energy distribution for atoms obeying a Maxwell-Boltzmann distribution. However, by the arguments above this also approximates the rate at which typical atoms are scattered into the high-energy region of the distribution for atoms that follow a Maxwell-Boltzmann distribution truncated at the energy <math>\eta kT</math>. Therefore, <math>\tau_{ev}</math> is the evaporation time constant. | Where we have defined <math>\tau_{ev}=1/(n_0 \sigma \bar{v} \eta e^{-\eta}) </math>. This expression describes the rate at which atoms with energy greater than <math>\eta kT</math> are scattered out of that region of the energy distribution for atoms obeying a Maxwell-Boltzmann distribution. However, by the arguments above this also approximates the rate at which typical atoms are scattered into the high-energy region of the distribution for atoms that follow a Maxwell-Boltzmann distribution truncated at the energy <math>\eta kT</math>. Therefore, <math>\tau_{ev}</math> is the evaporation time constant. | ||
| − | + | It is helpful to look at the ratio <math>\lambda=\tau_{ev}/\tau_{el}</math>, where <math>\tau_{el}</math> is the mean time between elastic collisions for typical atoms in the distribution. Therefore, <math>\lambda</math> is the typical number of collisions necessary to cause an atom to evaporate. For these atoms <math>v_{rel}=\sqrt{2}\, \bar{v}</math> and so <math>\tau_{el}=1/(n_0 \sigma \sqrt{2} \, \bar{v})</math>. This gives | |
| − | ::[[Image:Techniques_for_cooling_to_ultralow_temperatures-ecool6.png|thumb|400px|none|]] | + | :<math> |
| + | \lambda | ||
| + | =\frac{\sqrt{2} e^\eta}{\eta} | ||
| + | </math> | ||
| + | |||
| + | This allows us to write the simple formula | ||
| + | |||
| + | :<math> | ||
| + | \frac{\dot{N}}{N} | ||
| + | =\frac{-1}{\lambda \tau_{el}} | ||
| + | </math> | ||
| + | |||
| + | The consequences of large <math>\eta</math> are now clear. Increasing <math>\eta</math> increases the efficiency of the evaporation, <math>\alpha(\eta)</math>, so that more atoms are left at the end. However, increasing <math>\eta</math> also exponentially increases the number of collisions needed to evaporate an atom <math>\lambda(\eta)</math> which slows down the evaporation. If the evaporation is too slow, atoms are lost due to background collisions, etc. Therefore a value of <math>\eta</math> that makes a compromise between <math>\alpha(\eta)</math> and <math>\lambda(\eta)</math> must be chosen. | ||
| + | |||
| + | <!-- ::[[Image:Techniques_for_cooling_to_ultralow_temperatures-ecool5.png|thumb|400px|none|]] --> | ||
| + | |||
| + | <!-- ::[[Image:Techniques_for_cooling_to_ultralow_temperatures-ecool6.png|thumb|400px|none|]] --> | ||
| + | |||
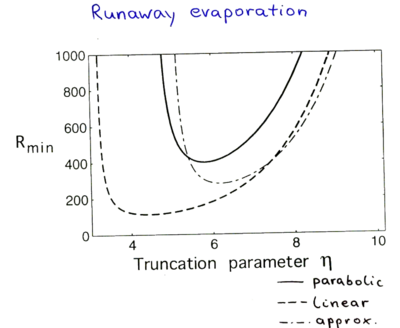
| + | === Runaway Evaporation === | ||
::[[Image:Techniques_for_cooling_to_ultralow_temperatures-ecool7.png|thumb|400px|none|]] | ::[[Image:Techniques_for_cooling_to_ultralow_temperatures-ecool7.png|thumb|400px|none|]] | ||
Revision as of 03:34, 17 April 2017
- Also see: Magnetic trapping and evaporative cooling (2009 Class Notes File:Magnetic trappping and evaporative cooling.pdf)
Magnetic confinement
The principle of magnetic trapping is deflection by magnetic fields. This is at the heart of the Stern-Gerlach experiment in 1925. In the 1960's it was realized that magnetic confinement could be realized. It was first realized for neutrons, in 1978 by Paul. The exciting 1980's was magnetic cooling and trapping, including work by Phillips, Pritchard, and Greytak, on sodium and other atoms.
Earnshaw theorem
Recall the Earnshaw theorem, previously discussed for the optical force, and for trapped ions. This states that trapping requires or . For a rigid charge distribution, with positions , where is the center of mass motion and is the relative position of charge , we find that
must satisfy . An arbitrary rigid charge distribution (with arbitrary multiple moments) cannot be kept in stable equilibrium at rest in free space in static electric fields. The same theorem applies for magnetic multipole distributions.
Circumventing the Earnshaw theorem
Sir James-Jeans, in 1925, pointed out a way around this limit. If the matter is polarizable, this can change the electric fields.
The displacement of the trapped particle or of the field generated by a particle which are energetically favorable are forbidden due to constraints. This leads to a loophole, as exemplified by magnet stabilized above a superconductor:
The constraint that no magnetic field may enter a superconductor causes shielding currents to be created which stabilize the trapping configuration for the levitated particle. This happens in a configuration which is not a minimum of the magnetic energies.
Of course, electronic feedback can be used to create a stable trapping potential, providing time varying fields.
Another example is provided by diamagnetic matter. Such matter expels an external field.
Yet another, important, example is if is constant. This constrains angular momentum; the dipole moment is not allowed to flip over to move into what might be a more energetically favorable configuration.
Trapping a magnetic moment
Now let us see how these ideas can allow trapping of an object with a magnetic moment. Let us consider when a magnetic moment might be constrained such that the potential depends only on the absolute value of the magnetic field, .
Wing's theorem
Wing's theorem (1983) says that in a region devoid of charges and currents, quasistatic electric of magnetic fields can have local minima, but not local maxima. The proof of this fact is by non-existence of a maximum by contradiction. Assume is a max. Then
It follows that
and the cross-term must be always negative. Without loss of generality, this requires, for example, . However, that presents a contradiction with the Laplace equation (recall Earnshaw's theorem). A minimum, on the other hand, is possible because of the term. There can thus be local minima, but not local maxima, in an electric field configuration.
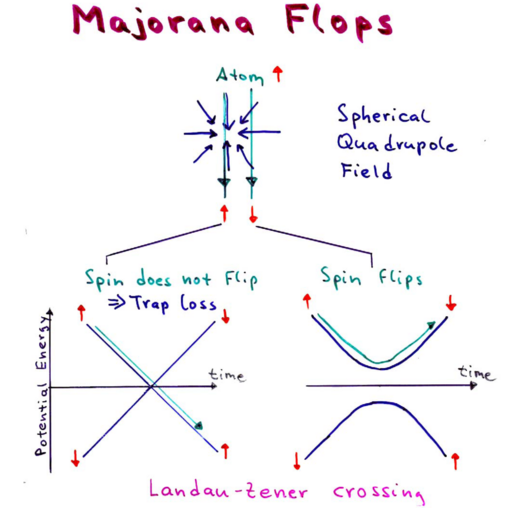
By Wing's theorem, since there can only be local minima (and not maxima) in a static magnetic field, to have a stable magnetic trap, must be antiparallel to . The limits to stability of magnetic traps include spin relaxation (involving nuclear magnetic moment changes), dipolar relaxation (involving angular momenta changes of atomic motion), and Majorana flops.
Classical magnetic moment in a trap
More specifically, to trap, must be antiparallel to only adiabatically, as follows. Consider the problem of trapping a classical magnetic moment. Such a magnetic moment precesses in a magnetic field:
What is needed is for the precession of the magnetic moment to follow and maintain the same angle with respect to the field, as the moment moves in an inhomogeneous field. If the field changes slowly, the precession will follow the field, but if the field changes too rapidly, the spin may no longer be antiparallel with respect to the field:
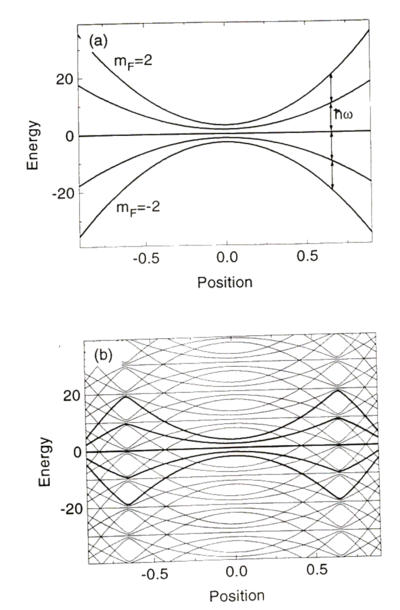
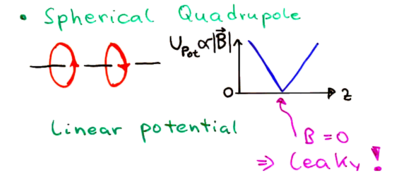
In the spherical quadrupole magnetic trap, made by anti-Helmholz coils, spins can flip in the following way, known as Majorana flops. As an atom moves through the field configuration:
it should flip its moment as it moves through the center of the field; however, there is a zero in the field at the center, at which point the spin can easily find itself aligned parallel with the field upon the atom's exit. This causes atoms to leak from the trap.
Levitron example
Counter-intuitively, spinning the top faster does not lead to a more stable trap. Rather, it causes increased instability; this is due to a process we may think of as being a classical analog of Majorana flops.
The levitron works in the following way. At ,
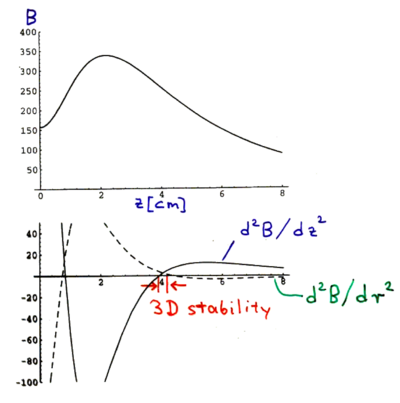
etc. The magnetic field above the center of the spinning magnet is thus
The second derviative of the magnetic field must give positive curvature along both the radial and axial directions, for the trap to be stable. This plot shows there is only a very small stability range for the trap.
Recall our trapping criterion: must stay anti-parallel to . This happens due to precession, as long as . The precession frequency of a gyroscope is given by the torque, so
where is the moment of inertia. is thus violated for large or . Around , one gets spin flips, referred to as Majorana flops in magnetic trapping of atoms.
Magnetic traps
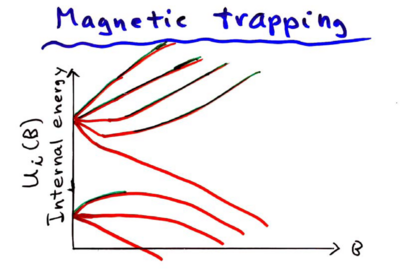
In magnetic traps, . can only have local minima, and not maxima, so we must ensure the angle of the field is such that the prefactors are positive. For Rubidium, we have these energy level dependencies on the field:
The only states which are trapped are thus which have positive energy versus magnetic field slope.
The spherical quadrupole trap has a linear field dependency versus position going through the center:
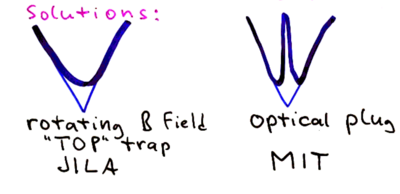
A plug is thus needed to close the hole at . Two strategies are (1) to use a repulsive dipole force, or (2) use a rapidly rotating magnetic field -- the TOP trap:
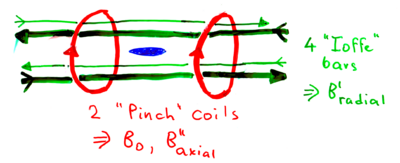
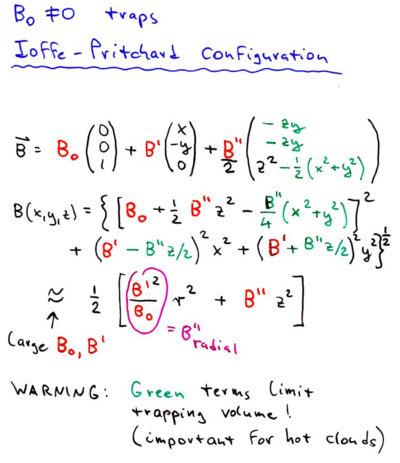
The TOP trap adds a linear bias field to displace the origin of the trap, such that the time-average potential produces no zero in the magnetic field. The Ioffe-Pritchard trap does this, with a constant field and a curvature created by four "Ioffe" bars, adding to the two "Pinch" coils:
The Ioffe bars create the quadrature field,
and the pinch field creates a linear field,
where the last term on the right is recognized to be . This configuration can be used with :
In practice the simple arrangement of wires described above is somewhat inconvenient for experiments because running the Ioffe bars through the vacuum chamber is a technical challenge. However the same magnetic trap (to leading order around the trap center) can be generated by wires arranged in a cloverleaf trap as depicted in the following photo.
The two pinch coils are visible on the left and the right depicts the four Ioffe bars which have been bent and wrapped into a circle.
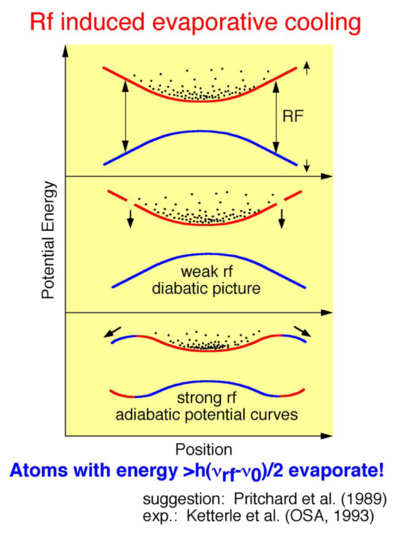
Evaporative cooling
In the 1980s and 1990s laser cooling developments were able to push phase space densities to closer and closer to one, which is the threshold for Bose-Einstein condensation. However, as the cold atom clouds reached higher densities, laser cooling schemes hit an issue. When the atomic clouds were not too dense, each atom interacted with the laser field independently and cooling schemes that were designed to cool single atoms were able to cool clouds with multiple atoms. However, when the clouds became more dense, this was no longer the case. At high densities, atoms that entered an excited state while scattering a photon would interact strongly with a nearby atom. This would cause both atoms to fly out of the trap in a process called a light-assisted collision. This put a serious limit on the atomic densities that were achieved with laser cooling schemes. Therefore, atomic physicists wanted a final cooling technique to push their cold dense atomic clouds to condensation without scattering photons as in laser cooling schemes. This led to the development of a conceptually simple solution: evaporative cooling.
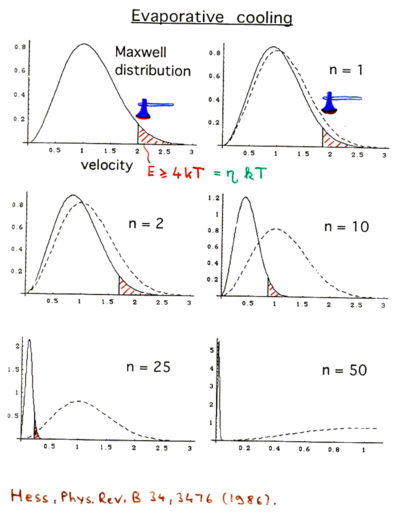
Evaporation allows cooling atoms to nanokelvin, or even subnanokelvin, temperatures even at high densities. It is the scheme that lead to the first BECs and remains a critical tool for nearly all degenerate gas experiments. To get a simple qualitative understanding for how it works, it's best to start by modeling the cooling as a series of discrete steps as in the figure below.
We start with a thermal cloud of atoms at a temperature , and then release all the atoms with energy greater that , where is an adjustable parameter of the cooling scheme. For this figure we've chosen . Since we've released the hottest atoms, the remaining atoms are have lower energy on average. We then wait for the cloud to thermalize and we're left with a gas that has fewer atoms and a colder temperature . The process then starts again now releasing all atoms with energy greater than . As the number for steps taken increases, the total atom number decreases, but the atomic velocities decrease, corresponding to a cooler cloud. This magnitude of this effect can be clearly seen by comparing the distributions to the initial distribution represented with a dashed line. In practice this process is not done by releasing the hottest atoms at discrete times. Instead the trap is adjusted to allow atoms with energy above a threshold energy to escape. This threshold energy can then be adjusted as the atoms evaporate to keep roughly constant.
Power Law Scalings
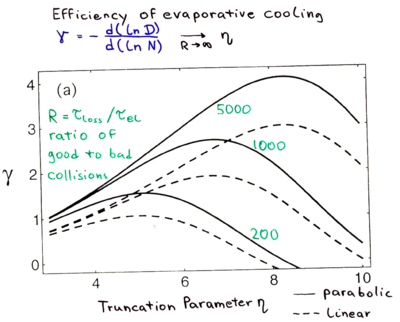
We see that evaporative cooling gives us the ability to trade atom number for lower temperatures. This begs the question, how good is this tradeoff? In the evaporative process a fraction of the atoms are traded for a fractional decrease of the temperature. This means that the relevant quantities are related by power laws and we should look at the derivatives of the logs of different quantities. For example, consider , the ratio of the fractional change in temperature to the fractional change in atom number, which therefore characterizes how efficient this atom-number-for-temperature trade is:
Now let's examine the case where the the trap potential is given by a power law
where is the number of dimensions and is chosen to give the correct scaling. For example, we'd have and for a harmonic trap in three dimensions. By the equipartition theorem, the kinetic energy and therefore temperature also scale the same way which we can invert to get . This leads to , and above we found that and so we can write . In this way we can write many interesting quantities of this system as power laws of with different exponents. The table below summarizes some results
| Quantity | Scaling Exponent | |
| Number of Atoms | ||
| Temperature | ||
| Volume | ||
| Number Density | ||
| Phase-Space Density | ||
| Elastic Collision Rate |
From this chart, the importance of is very clear; it characterizes all of the scalings for the thermodynamic quantities. Therefore we are interested in finding in terms of the experimentally controlled parameter . As depicted in the figure below, atoms with energy at least escape the trap, taking with them of energy with .
From the equipartition theorem, the total energy in the system is (assuming 3D motion). The total energy before and after the evaporation of atoms can then be related as (note that and are negative)
This can be rewritten as
Glancing back at the definition for above gives the result
Because the odds of an atom reaching a high energy state is exponentially suppressed, we can approximate that so that each atom takes of energy when it evaporates.
Speed of Evaporation
From this expression it seems that the evaporation can be arbitrarily efficient, just make as large as desired. The most extreme case of this would be to wait until one atom reaches so far into the high energy tail of the thermal distribution that it contains the entire energy of the system. In that case, simply evaporating one atom would leave the system at zero temperature. However, in practice extremely efficient evaporation like this is not feasible because the time necessary to evaporate increases with and the available time is limited due to other loss processes, such as background gas collisions, trap heating, or grad student life time. Therefore it is necessary to consider how quickly the evaporation takes place to determining the maximum achievable. To explore this further we examine the case of evaporation at constant .
To determine the rate of evaporation we must calculate the rate at which collisions produce atoms with energy greater than . The simplest way to do this is to approximate and invoke the concept of detailed balance. Consider the velocity distribution of the atoms at the center of the trap of the trap where we take the potential energy to be zero, so that the atom's kinetic energy is equal to its total energy. When the atom's velocity distribution for this trap of finite depth is well approximated by a Maxwell-Boltxman truncated at the trap depth . We then need to figure out that rate at which atoms collide to produce atoms with energy greater than the trap depth. It is difficult to calculate this for the truncated distribution directly, however we can easily calculate it for the untruncated distribution and make the approximation that the rate is same for both distributions, which is clearly true in the limit .
For the untruncated distribution, the principle of detailed balance states that the rate at which atoms are scattered into the region is equal to the rate at which atoms already in that region are scattered out. Because atoms in that region have much larger than average energy, they almost always lose a significant amount of energy when they collide with other atoms and are therefore scattered out of that region of the distribution almost every time they collide. From this we see that the rate at which atoms in the high energy region are scattered out is approximately the number of atoms in that region divided by their mean time between collisions. For large the fraction of atoms with energy above is approximately . We can also approximate their typical velocity as where denotes the average speed of all atoms. This is an ok approximation since, again, the odds of having an energy much greater than are exponentially suppressed. For an atom with velocity , the mean time between collisions is where is the collision cross section, is the atomic number density, and is the typical relative velocity with respect to the other atoms. For the high energy atoms we may approximate . Combining these results shows that the rate at which atoms leave this high energy region of the distribution is given by
Where we have defined . This expression describes the rate at which atoms with energy greater than are scattered out of that region of the energy distribution for atoms obeying a Maxwell-Boltzmann distribution. However, by the arguments above this also approximates the rate at which typical atoms are scattered into the high-energy region of the distribution for atoms that follow a Maxwell-Boltzmann distribution truncated at the energy . Therefore, is the evaporation time constant.
It is helpful to look at the ratio , where is the mean time between elastic collisions for typical atoms in the distribution. Therefore, is the typical number of collisions necessary to cause an atom to evaporate. For these atoms and so . This gives
This allows us to write the simple formula
The consequences of large are now clear. Increasing increases the efficiency of the evaporation, , so that more atoms are left at the end. However, increasing also exponentially increases the number of collisions needed to evaporate an atom which slows down the evaporation. If the evaporation is too slow, atoms are lost due to background collisions, etc. Therefore a value of that makes a compromise between and must be chosen.
Runaway Evaporation
References
- Further reading on magnetic trapping: W. K., D.S. Durfee, D.M. Stamper-Kurn, Varenna Lecture Notes 1999, pp. 80-89
- Further reading on evaporative cooling:: W. Ketterle and N.J. van Druten, Adv. At. Mol. Opt. Phys. 37, 181-236 (1986). Relevant pages: pp. 181-193


























































































![{\displaystyle {\dot {N}}=-N{\bigg [}2e^{-\eta }{\sqrt {\frac {\eta }{\pi }}}{\bigg ]}{\bigg [}\sigma n_{0}{\sqrt {\pi \eta }}{\frac {\bar {v}}{2}}{\bigg ]}=-Nn_{0}\sigma {\bar {v}}\eta e^{-\eta }={\frac {-N}{\tau _{ev}}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d719d1c2b5558f706eb780f1235945b84d86503a)