Difference between revisions of "Magnetic resonance of a classical magnetic moment"
imported>Ketterle |
imported>Ketterle |
||
| (26 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| + | == Magnetic Resonance: The Classical Magnetic Moment in a Spatially Uniform Field == | ||
| + | |||
| + | === Harmonic oscillator versus two-level systems === | ||
| + | |||
Now, unlike in classical mechanics, most resonances studied in atomic physics | Now, unlike in classical mechanics, most resonances studied in atomic physics | ||
are not harmonic oscillators, but two-level systems. Unlike harmonic | are not harmonic oscillators, but two-level systems. Unlike harmonic | ||
| Line 26: | Line 30: | ||
the motion of a classical magnetic moment in a uniform field provides a model | the motion of a classical magnetic moment in a uniform field provides a model | ||
that captures almost all features of the quantum-mechanical two-level system, | that captures almost all features of the quantum-mechanical two-level system, | ||
| − | the exception | + | the exception being the projection onto one of the two possible outcomes in a |
measurement. | measurement. | ||
| − | + | ||
| − | |||
=== Magnetic Moment in a Static Field === | === Magnetic Moment in a Static Field === | ||
The interaction energy of a magnetic moment <math> {\bf{\mu}} </math> with a magnetic field | The interaction energy of a magnetic moment <math> {\bf{\mu}} </math> with a magnetic field | ||
| Line 57: | Line 60: | ||
<blockquote> | <blockquote> | ||
| + | |||
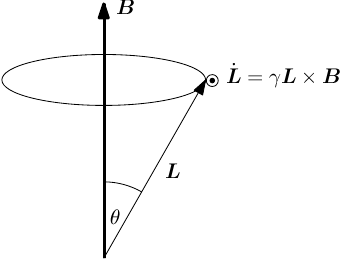
| + | ::[[Image:resonances-static-precession.png|thumb|400px|none|]] | ||
{fig:static_precession} | {fig:static_precession} | ||
| − | |||
Precession of a the magnetic moment and associated angular momentum | Precession of a the magnetic moment and associated angular momentum | ||
about a static field <math> {\bf{B}} </math>. | about a static field <math> {\bf{B}} </math>. | ||
| Line 73: | Line 77: | ||
for protons <math>\gamma_e=2\pi\times\unit{4.2}{\kilo\hertz\per G}</math>. | for protons <math>\gamma_e=2\pi\times\unit{4.2}{\kilo\hertz\per G}</math>. | ||
| − | === | + | === Transformation to a Rotating Coordinate System === |
A vector <math> {\bf{A}} </math> rotating at constant angular velocity <math> {\bf{\Omega}} </math> is | A vector <math> {\bf{A}} </math> rotating at constant angular velocity <math> {\bf{\Omega}} </math> is | ||
described by | described by | ||
| Line 111: | Line 115: | ||
=== Larmor's Theorem for a Charged Particle in a Magnetic Field === | === Larmor's Theorem for a Charged Particle in a Magnetic Field === | ||
The vanishing of the torque on a magnetic moment when viewed in the correct | The vanishing of the torque on a magnetic moment when viewed in the correct | ||
| − | rotating frame is reminiscent of Larmor's | + | rotating frame is reminiscent of Larmor's theorem for the motion of a charged |
particle in a magnetic field, which we now present. | particle in a magnetic field, which we now present. | ||
| + | |||
| + | According to answers.com, Lamor's theorem can be stated as | ||
| + | For a system of charged particles, all having the same ratio of charge to mass, moving in a central field of force, the motion in a uniform magnetic induction B is, to first order in B, the same as a possible motion in the absence of B except for the superposition of a common precession of angular frequency equal to the Larmor frequency. | ||
| + | |||
If the Lorentz force acts in an inertial frame, | If the Lorentz force acts in an inertial frame, | ||
:<math> | :<math> | ||
| Line 156: | Line 164: | ||
for example a neutral atom can have a net magnetic moment, and the neutron possesses a | for example a neutral atom can have a net magnetic moment, and the neutron possesses a | ||
magnetic moment in spite of being neutral - and it experiences no net force in a uniform | magnetic moment in spite of being neutral - and it experiences no net force in a uniform | ||
| − | magnetic field. Furthermore, the rotating co-ordinate transformation is exact for a | + | magnetic field. Furthermore, the rotating co-ordinate transformation is exact for a magnetic moment, whereas Larmor's theorem for the motion of a charged particle is only valid when the <math> B^2 </math> term is neglected. |
| − | + | ||
| − | when the <math> B^2 </math> term is neglected. | + | Note: A breakdown of Larmor's theorem occurs for free electrons. Free electrons orbit in a magnetic field at the cyclotron frequency <math>\omega_c=qB/m</math> which is ''twice'' Larmor's frequency. The reason is that the orbiting velocity is now given by <math>v=\omega_c r </math>. As can be seen in the equation above, in this case, the centrifugal force equals minus one half of the Coriolis force, and therefore twice the frequency of the rotating frame is needed to cancel the effects of the applied magnetic field. |
=== Rotating Magnetic Field on Resonance === | === Rotating Magnetic Field on Resonance === | ||
<blockquote> | <blockquote> | ||
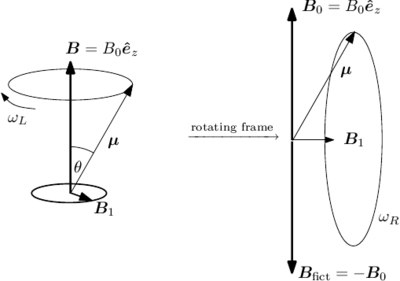
| − | [[Image:resonances-rotating-frame.png|thumb|400px|none|]] | + | ::[[Image:resonances-rotating-frame.png|thumb|400px|none|]] |
| − | + | {fig:rotating_frame} Field and moment vectors in the static and rotating frames for the case | |
| − | of resonant drive. | + | of resonant drive. |
</blockquote> | </blockquote> | ||
| Line 185: | Line 193: | ||
A magnetic moment initially along the <math>- {\bf{\hat e}} _z</math> axis will point along | A magnetic moment initially along the <math>- {\bf{\hat e}} _z</math> axis will point along | ||
the <math>+ {\bf{\hat e}} _z</math> axis at a time <math>T</math> given by <math>\omega_r T=\pi</math>, while a | the <math>+ {\bf{\hat e}} _z</math> axis at a time <math>T</math> given by <math>\omega_r T=\pi</math>, while a | ||
| − | magnetic moment parallel or antiparallel to applied magnetic field <math> {\bf{B}} _1</math> | + | magnetic moment parallel or antiparallel to the applied magnetic field <math> {\bf{B}} _1</math> |
does not precess in the rotating frame. | does not precess in the rotating frame. | ||
| − | + | ||
| − | Assume the magnetic moment is initially pointing | + | '''Question:''' {q:rabi_freq_blue_detuned} Assume the magnetic moment is initially pointing |
along the <math>- {\bf{\hat e}} _z</math> axis. Assume that a rotating field <math>B_1\ll B_0</math> is | along the <math>- {\bf{\hat e}} _z</math> axis. Assume that a rotating field <math>B_1\ll B_0</math> is | ||
applied, but that it rotates at a frequency <math>\omega_1>\omega_0</math>, | applied, but that it rotates at a frequency <math>\omega_1>\omega_0</math>, | ||
| Line 194: | Line 202: | ||
Compared to the on-resonant case, <math>\omega_1=\omega_0</math>, is the oscillation frequency of | Compared to the on-resonant case, <math>\omega_1=\omega_0</math>, is the oscillation frequency of | ||
the magnetic moment. | the magnetic moment. | ||
| − | |||
* larger | * larger | ||
* the same | * the same | ||
* smaller | * smaller | ||
| − | + | '''Question:''' Same question as \ref{q:rabi_freq_blue_detuned} but for <math>\omega_1<\omega_0</math>. | |
| − | Same question as \ref{q:rabi_freq_blue_detuned} but for <math>\omega_1<\omega_0</math>. | ||
| − | |||
=== Rotating Magnetic Field Off-Resonance === | === Rotating Magnetic Field Off-Resonance === | ||
| Line 237: | Line 242: | ||
<blockquote> | <blockquote> | ||
| − | + | ||
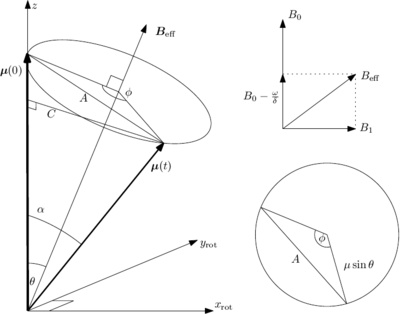
| − | [[Image:resonances-classical-rabi-construction.png|thumb|400px|none|]] | + | ::[[Image:resonances-classical-rabi-construction.png|thumb|400px|none|]] |
| − | + | {fig:rotating_coord_construction} Geometrical relations for the spin in combined static and rotating | |
magnetic fields, viewed in the frame co-rotating with the drive field | magnetic fields, viewed in the frame co-rotating with the drive field | ||
<math> {\bf{B}} _1</math>. At lower right is a view looking straight down the | <math> {\bf{B}} _1</math>. At lower right is a view looking straight down the | ||
| − | <math> {\bf{B}} _\text{eff}</math> axis. | + | <math> {\bf{B}} _\text{eff}</math> axis. |
</blockquote> | </blockquote> | ||
| Line 267: | Line 272: | ||
\end{align}</math> | \end{align}</math> | ||
so that | so that | ||
| + | :{eq:classical_rabi_flopping} | ||
:<math>\begin{align} | :<math>\begin{align} | ||
\cos \alpha | \cos \alpha | ||
| Line 288: | Line 294: | ||
quantum-mechanical result. | quantum-mechanical result. | ||
| − | === "Rapid" Adiabiatic Passage === | + | If we define the quantity <math> P= \frac{\mu_z(t)-\mu_z(0)}{2\mu_z(0)} </math>, we obtain |
| + | |||
| + | <math> P= \frac{\omega_R^2}{\Omega_R^2} \sin^2 \frac{\Omega_R t}{2} </math> | ||
| + | |||
| + | In the quantum mechanical treatment (see below), <math> P </math> is the probability to find the system in the second state, or the spin flip probability. | ||
| + | |||
| + | === "Rapid" Adiabiatic Passage (classical treatment) === | ||
Rapid adiabatic passage is a technique for inverting a spin by (slowly) sweeping | Rapid adiabatic passage is a technique for inverting a spin by (slowly) sweeping | ||
the detuning of a drive field through resonance. "Slowly" means slowly | the detuning of a drive field through resonance. "Slowly" means slowly | ||
compared to the Larmor frequency <math>\gamma B_\text{eff}</math> about the effective | compared to the Larmor frequency <math>\gamma B_\text{eff}</math> about the effective | ||
| − | static field in the rotating frame for all times. | + | static field in the rotating frame for all times ("Rapid" means fast compared to relaxation processes which we neglect here). |
| − | follows. Assume the detuning is initially negative (<math>\delta<0</math>, | + | |
| + | The physical picture is as follows. Assume the detuning is initially negative (<math>\delta<0</math>, | ||
<math> \left| \delta\gg\omega_R\right| </math>). Since | <math> \left| \delta\gg\omega_R\right| </math>). Since | ||
:<math> | :<math> | ||
| Line 306: | Line 319: | ||
<blockquote> | <blockquote> | ||
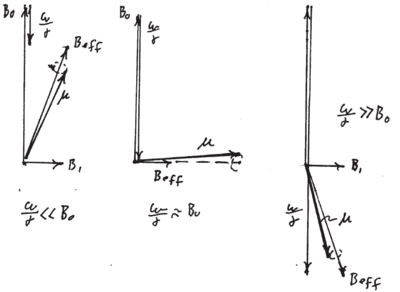
| − | + | ::[[Image:resonances-adiab.png|thumb|400px|none|]] | |
| − | [[Image:resonances-adiab.png|thumb|400px|none|]] | + | {fig:rapid_adiabatic_passage} {Motion of the spin during rapid adiabatic passage, viewed in the frame |
| − | |||
rotating with <math> {\bf{B}} _1</math>. The spin's rapid precession locks it to the | rotating with <math> {\bf{B}} _1</math>. The spin's rapid precession locks it to the | ||
direction of <math> {\bf{B}} _\text{eff}</math> and thus it is dragged through an angle <math>\pi</math> | direction of <math> {\bf{B}} _\text{eff}</math> and thus it is dragged through an angle <math>\pi</math> | ||
| − | as the frequency is swept through resonance. | + | as the frequency is swept through resonance. |
</blockquote> | </blockquote> | ||
| Line 326: | Line 338: | ||
smallest and equal to the resonant Rabi frequency <math>\omega_R</math> at <math>\delta=0</math>, the | smallest and equal to the resonant Rabi frequency <math>\omega_R</math> at <math>\delta=0</math>, the | ||
adiabatic requirement is most severe there, i.e. near <math>\theta=\pi/2</math>. | adiabatic requirement is most severe there, i.e. near <math>\theta=\pi/2</math>. | ||
| − | Near <math>\theta=\pi/2</math> we have, with <math>B_z=B_0- | + | Near <math>\theta=\pi/2</math> we have, with <math>B_z=B_0-\omega(t)/\gamma</math>, |
:<math> | :<math> | ||
\left| \dot\theta\right| =\frac{ \left| \dot{B}_z\right| }{B_1}=\frac{ \left| \dot\omega\right| }{\gamma B_1} | \left| \dot\theta\right| =\frac{ \left| \dot{B}_z\right| }{B_1}=\frac{ \left| \dot\omega\right| }{\gamma B_1} | ||
| Line 337: | Line 349: | ||
<math>\omega</math> per Rabi period <math>T=2\pi/\omega_R</math>, | <math>\omega</math> per Rabi period <math>T=2\pi/\omega_R</math>, | ||
<math> \left| \Delta\omega\right| = \left| \dot\omega\right| T= \left| \dot\omega\right| /\omega_R 2\pi</math>, | <math> \left| \Delta\omega\right| = \left| \dot\omega\right| T= \left| \dot\omega\right| /\omega_R 2\pi</math>, | ||
| − | must be small compared to the Rabi frequency <math>\omega_R</math>. | + | must be small compared to the Rabi frequency <math>\omega_R</math>. |
| − | + | ||
| − | + | Note that the inversion of the spin is independent of whether <math>\omega</math> is swept up or down through the resonance. | |
| − | |||
| − | |||
| − | </math> | ||
| − | |||
Latest revision as of 14:45, 17 February 2010
Contents
- 1 Magnetic Resonance: The Classical Magnetic Moment in a Spatially Uniform Field
- 1.1 Harmonic oscillator versus two-level systems
- 1.2 Magnetic Moment in a Static Field
- 1.3 Transformation to a Rotating Coordinate System
- 1.4 Larmor's Theorem for a Charged Particle in a Magnetic Field
- 1.5 Rotating Magnetic Field on Resonance
- 1.6 Rotating Magnetic Field Off-Resonance
- 1.7 "Rapid" Adiabiatic Passage (classical treatment)
Magnetic Resonance: The Classical Magnetic Moment in a Spatially Uniform Field
Harmonic oscillator versus two-level systems
Now, unlike in classical mechanics, most resonances studied in atomic physics are not harmonic oscillators, but two-level systems. Unlike harmonic oscillators, two-level systems show saturation. When a harmonic oscillator is driven longer or faster, higher and higher excited states are populated: the oscillator amplitude can become arbitrarily large. In contrast, the amplitude of oscillation in a two-level system is limited to one half in the appropriate dimensionless units.\footnote{An analogous dimensionless amplitude for the harmonic oscillator would be the amplitude measured in units of the oscillator ground state size.} Why, and under what conditions, do classical harmonic oscillator models of a two-level system work? A two-level system of energy difference can be approximated by a harmonic oscillator of frequency when saturation effects in the two-level system are negligible, i.e. when the population of the second excited state in the harmonic oscillator problem is negligible, or equivalently when the population ratio of the first excited state to the the ground state is small: . This is the basis for the classical Lorentz model of the electron bound in the atom that describes many linear atomic properties (for instance the refractive index) very well. See "The origin of the refractive index" in chapter 31 of the Feynman Lectures on Physics \cite{Feynman1963}. When saturation comes into play, i.e. when the initial ground state is appreciably depleted, the harmonic oscillator ceases to be a good model. The limit on the oscillation amplitude in a two-level system suggests that a classical system with periodic evolution and a limit on the amplitude, namely rotation, could provide a better classical model of a two-level system. Indeed, the motion of a classical magnetic moment in a uniform field provides a model that captures almost all features of the quantum-mechanical two-level system, the exception being the projection onto one of the two possible outcomes in a measurement.
Magnetic Moment in a Static Field
The interaction energy of a magnetic moment with a magnetic field is given by
In a uniform field the force
vanishes, but the torque
does not. For an angular momentum the equation of motion is then
where we have introduced the gyromagnetic ratio as the proportionality constant between angular momentum and magnetic moment, as shown in Figure \ref{fig:static_precession}.
{fig:static_precession} Precession of a the magnetic moment and associated angular momentum about a static field .
This describes the precession of the magnetic moment about the magnetic field with angular frequency
is known as the Larmor frequency. For electrons we have Failed to parse (unknown function "\unit"): {\displaystyle \gamma_e=2\pi\times\unit{2.8}{\mega\hertz\per G}} , for protons Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \gamma_e=2\pi\times\unit{4.2}{\kilo\hertz\per G}} .
Transformation to a Rotating Coordinate System
A vector rotating at constant angular velocity is described by
Then the rates of change of measured in a coordinate system rotating at and in an inertial system are related by
This follows immediately from the following facts:
- If is constant in the rotating system then .
- If then .
- Coordinate rotation is a linear transformation.
This transformation is sometimes abbreviated as the schematic rule
It follows that the angular momentum in a rotating frame obeys
If we choose , then is constant in the rotating frame. Often it is useful to think of a fictitious magnetic field that appears in a rotating frame.
Larmor's Theorem for a Charged Particle in a Magnetic Field
The vanishing of the torque on a magnetic moment when viewed in the correct rotating frame is reminiscent of Larmor's theorem for the motion of a charged particle in a magnetic field, which we now present.
According to answers.com, Lamor's theorem can be stated as For a system of charged particles, all having the same ratio of charge to mass, moving in a central field of force, the motion in a uniform magnetic induction B is, to first order in B, the same as a possible motion in the absence of B except for the superposition of a common precession of angular frequency equal to the Larmor frequency.
If the Lorentz force acts in an inertial frame,
then in the rotating frame, according to the rule \ref{eqn:rot_frame_transformation} we have
resulting in a force in the rotating frame given by
where we have used . Choosing
yields
if the field is not too large. Thus the Lorentz force approximately disappears in the rotating frame.
Although Larmor's theorem is suggestive of the rotating co-ordinate transformation, it is important to realize that the two transformations, though identical in form, apply to fundamentally different systems. A magnetic moment is not necessarily charged- for example a neutral atom can have a net magnetic moment, and the neutron possesses a magnetic moment in spite of being neutral - and it experiences no net force in a uniform magnetic field. Furthermore, the rotating co-ordinate transformation is exact for a magnetic moment, whereas Larmor's theorem for the motion of a charged particle is only valid when the term is neglected.
Note: A breakdown of Larmor's theorem occurs for free electrons. Free electrons orbit in a magnetic field at the cyclotron frequency which is twice Larmor's frequency. The reason is that the orbiting velocity is now given by . As can be seen in the equation above, in this case, the centrifugal force equals minus one half of the Coriolis force, and therefore twice the frequency of the rotating frame is needed to cancel the effects of the applied magnetic field.
Rotating Magnetic Field on Resonance
{fig:rotating_frame} Field and moment vectors in the static and rotating frames for the case of resonant drive.
Consider a magnetic moment precessing about a field with in spherical coordinates, where . Assume that we now apply an additional field , in the -plane rotating at . To solve the resulting problem it is simplest to go into the rotating frame (Figure \ref{fig:rotating_frame}). Then is stationary, say along , and there is an additional fictitious field which cancels the field . So in the rotating frame we are left just with the static field , and the magnetic moment precesses about at the Rabi frequency
A magnetic moment initially along the axis will point along the axis at a time given by , while a magnetic moment parallel or antiparallel to the applied magnetic field does not precess in the rotating frame.
Question: {q:rabi_freq_blue_detuned} Assume the magnetic moment is initially pointing along the axis. Assume that a rotating field is applied, but that it rotates at a frequency , where is the Larmor frequency for the static field . Compared to the on-resonant case, , is the oscillation frequency of the magnetic moment.
- larger
- the same
- smaller
Question: Same question as \ref{q:rabi_freq_blue_detuned} but for .
Rotating Magnetic Field Off-Resonance
If the rotation frequency of does not equal the Larmor frequency associated with the static field , then in the frame rotating with at frequency the static field is not completely cancelled by the fictitious field , but a difference along remains, giving rise to a total effective field in the rotating frame
The effective field is static, lies at an angle with the z
and is of magnitude
The magnetic moment precesses around it with an effective (sometimes called generalized) Rabi frequency
where is the Rabi frequency associated with , and is the detuning from resonance with the Larmor frequency .
Geometrical Solution for the Classical Magnetic Moment in Static and Rotating Fields
{fig:rotating_coord_construction} Geometrical relations for the spin in combined static and rotating magnetic fields, viewed in the frame co-rotating with the drive field . At lower right is a view looking straight down the axis.
Referring to Figure \ref{fig:rotating_coord_construction}, we have
On the other hand
so that
- {eq:classical_rabi_flopping}
With the Larmor frequency of the static field, the detuning, the resonant and the generalized Rabi frequencies. Note that the precession is faster, but the amplitude smaller for an off-resonant field than for the resonant case. The above result is also the correct quantum-mechanical result.
If we define the quantity , we obtain
In the quantum mechanical treatment (see below), is the probability to find the system in the second state, or the spin flip probability.
"Rapid" Adiabiatic Passage (classical treatment)
Rapid adiabatic passage is a technique for inverting a spin by (slowly) sweeping the detuning of a drive field through resonance. "Slowly" means slowly compared to the Larmor frequency about the effective static field in the rotating frame for all times ("Rapid" means fast compared to relaxation processes which we neglect here).
The physical picture is as follows. Assume the detuning is initially negative (, ). Since
the effective magnetic field initially points of a small angle relative to the axis. If the detuning is increased slowly compared to the Larmor frequency, the spin will continue to precess tightly around , which for points along the x axis, and for along the axis (see Figure \ref{fig:rapid_adiabatic_passage}).
{fig:rapid_adiabatic_passage} {Motion of the spin during rapid adiabatic passage, viewed in the frame rotating with . The spin's rapid precession locks it to the direction of and thus it is dragged through an angle as the frequency is swept through resonance.
Thus the magnetic moment, starting out along , ends up pointing along . Note that in the rotating frame remains always (almost) parallel to the effective field . A similar precess is used in magnetic traps for atoms, but there is a real, spatially dependent field constant in time. As the atom moves in this field, the fast precession of the magnetic moment about the local field keeps its direction locked to the local field, whose direction varies in the lab frame. Returning to rapid adiabatic passage, since the generalized Rabi frequency is smallest and equal to the resonant Rabi frequency at , the adiabatic requirement is most severe there, i.e. near . Near we have, with ,
where the exclamation point in indicates a requirement which we impose. Consequently, if the evolution is to be adiabatic, we must have . This means that the change of rotation frequency per Rabi period , , must be small compared to the Rabi frequency .
Note that the inversion of the spin is independent of whether is swept up or down through the resonance.