imported>Ketterle |
imported>Tailin |
| (2 intermediate revisions by 2 users not shown) |
| Line 29: |
Line 29: |
| | the beamsplitter; in particular, | | the beamsplitter; in particular, |
| | :<math> | | :<math> |
| − | \left[ \begin{array}{c}{a-ib}\\{b-ia}\end{array}\right] = B \left[ \begin{array}{c}{a}\\{b}\end{array}\right] | + | \frac{1}{\sqrt{2}} \left[\begin{array}{c}{a-ib}\\{b-ia}\end{array}\right] = B \left[ \begin{array}{c}{a}\\{b}\end{array}\right] |
| | \,. | | \,. |
| | </math> | | </math> |
| Line 191: |
Line 191: |
| | Given this, the uncertainty in <math>\phi</math> is | | Given this, the uncertainty in <math>\phi</math> is |
| | :<math> | | :<math> |
| − | \Delta\phi = \frac{\Delta\phi} | + | \Delta\phi = \frac{\Delta P} |
| | {\left| \frac{dP}{d\phi} \right|} = \frac{1}{\sqrt{n}} | | {\left| \frac{dP}{d\phi} \right|} = \frac{1}{\sqrt{n}} |
| | \,. | | \,. |
The Poisson distribution of photon number in coherent (laser) light
contributes an uncertainty of  to optical
measurements. It is therefore reasonable to anticipate that with
to optical
measurements. It is therefore reasonable to anticipate that with  photons, the uncertainty
photons, the uncertainty  with which an unknown phase
with which an unknown phase
 can be determined might be bounded below by
can be determined might be bounded below by  , based on the heuristic inequality
, based on the heuristic inequality  . Such a limit is known as being due to shot noise, arising
from the particle nature of photons, as we shall now see rigorously.
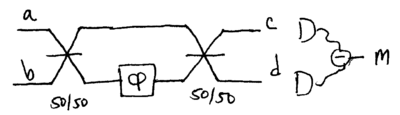
Consider a Mach-Zehnder interferometer constructed from two 50/50
beamsplitters, used to measure
. Such a limit is known as being due to shot noise, arising
from the particle nature of photons, as we shall now see rigorously.
Consider a Mach-Zehnder interferometer constructed from two 50/50
beamsplitters, used to measure  :
:
Let us analyze this interferometer, first by using a traditional
quantum optics approach in the Heisenberg picture, and second by using
single photons in the Schrodinger picture.
Sensitivity limit for Mach-Zehnder interferometer
Previously, we've defined the unitary transform for a quantum
beamsplitter as being a rotation about the  axis, so as to
avoid having to keep track of factors of
axis, so as to
avoid having to keep track of factors of  . For variety, let's now
use a different definition; nothing essential will change.
Let the 50/50 beamsplitter transformation be
. For variety, let's now
use a different definition; nothing essential will change.
Let the 50/50 beamsplitter transformation be
![{\displaystyle B={\frac {1}{\sqrt {2}}}\left[{\begin{array}{cc}{1}&{-i}\\{-i}&{1}\end{array}}\right]\,.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4e2d43ef535ef4f670f31b7ce4cfe7a15c1890f8)
This acts on ![{\displaystyle [a,b]^{T}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/dcbe0f3bd3d712a823544cc4985e4dbd35b6967d) to produce operators describing the output of
the beamsplitter; in particular,
to produce operators describing the output of
the beamsplitter; in particular,
![{\displaystyle {\frac {1}{\sqrt {2}}}\left[{\begin{array}{c}{a-ib}\\{b-ia}\end{array}}\right]=B\left[{\begin{array}{c}{a}\\{b}\end{array}}\right]\,.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3bcdd91d0e9a05a80b3165137ecd92a63b40bc81)
Similarly, the phase shifter acting on the mode operators performs
![{\displaystyle P=\left[{\begin{array}{cc}{1}&{0}\\{0}&{e^{i\phi }}\end{array}}\right]\,.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3f0a39e65796a1cc92d0a2ea729186ca5aca7a58)
The Mach-Zehnder transform is thus
![{\displaystyle {\begin{array}{rcl}U&=&BPB\\&=&{\frac {1}{2}}\left[{\begin{array}{cc}{1}&{-i}\\{-i}&{1}\end{array}}\right]\left[{\begin{array}{cc}{1}&{0}\\{0}&{e^{i\phi }}\end{array}}\right]\left[{\begin{array}{cc}{1}&{-i}\\{-i}&{1}\end{array}}\right]\\&=&-ie^{i\phi /2}\left[{\begin{array}{cc}{\sin(\phi /2)}&{\cos(\phi /2)}\\{\cos(\phi /2)}&{-\sin(\phi /2)}\end{array}}\right]\,.\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4aff63aa7808a03191a5315b3f548834ce336f41)
The way we have defined these transformations here, the output modes
of the interferometer,  and
and  , are
, are
![{\displaystyle \left[{\begin{array}{c}{c}\\{d}\end{array}}\right]=U\left[{\begin{array}{c}{a}\\{b}\end{array}}\right]\,.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c9786a4cebe3997ae8ab2e2a99556bd27a67b002)
We are interested in the difference between the photon numbers
measured at the two outputs,  , where the
extra factor of two is introduced for convenience. We find
, where the
extra factor of two is introduced for convenience. We find

The measurement result  is thus
is thus

Define  , and
, and  . Recognizing that
. Recognizing that
 is the difference in photon number between the two output arms,
and recalling that this is the main observable result from changing
is the difference in photon number between the two output arms,
and recalling that this is the main observable result from changing
 , we identify the signal we wish to see as being
, we identify the signal we wish to see as being  .
.
Ideally,
the output signal should go as  . The signal due to
. The signal due to  goes
as
goes
as  , and we shall see that this is the noise on the signal.
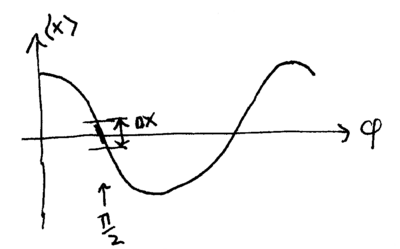
The average output signal
, and we shall see that this is the noise on the signal.
The average output signal  , as a function of
, as a function of  , looks like this:
, looks like this:
Note that if our goal is to maximize measurement sensitivity to
changes in  , then the best point to operate the interferometer
at is around
, then the best point to operate the interferometer
at is around  , since the slope
, since the slope  is largest
there. At this operating point, if the interferometer's inputs have
laser light coming into only one port, then the outputs have equal
intensity; thus, the interferometer is sometimes said to be
"balanced" when
is largest
there. At this operating point, if the interferometer's inputs have
laser light coming into only one port, then the outputs have equal
intensity; thus, the interferometer is sometimes said to be
"balanced" when  .
.
What is the uncertainty in our measurement of  , derived from the
observable
, derived from the
observable  ? By propagating uncertainties, this is
? By propagating uncertainties, this is

where

Let  , such that
, such that  , and
, and  .
.
Limit for coherent state input
For a coherent state input,  , we find
, we find

if we define  as the input state mean photon number.
Also,
as the input state mean photon number.
Also,

This is consitent with our intuition: the signal should go as  , and the undesired term goes as
, and the undesired term goes as  , so it is good that is small on
average. However, there are nontrivial fluctuations in
, so it is good that is small on
average. However, there are nontrivial fluctuations in  , because
, because

and  is nonzero for the
coherent state! Specifically, the noise in
is nonzero for the
coherent state! Specifically, the noise in  is
is

and thus the variance in the measurement result is

From Eq.(\ref{eq:l7-dphi}), it follows that the uncertainty in  is therefore
is therefore

This is a very reasonable result; as the number of photons used
increases, the accuracy with which  can be determined increases
with
can be determined increases
with  . The improvement arises because greater laser power
allows better distinction between the signals in
. The improvement arises because greater laser power
allows better distinction between the signals in  and
and  .
.
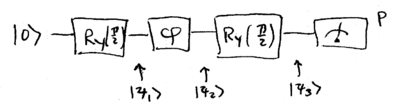
Limit for single photons
Another way to arrive at the same result, using single photons, gives
an alternate interpretation and different insight into the physics.
As we have seen previously, acting on the  ,
,  "dual-rail" photon state, a 50/50 beamsplitter performs a
"dual-rail" photon state, a 50/50 beamsplitter performs a
 rotation, and a phase shifter performs a
rotation, and a phase shifter performs a  rotation. The Mach-Zehnder interferometer we're using can thus be
expressed as this transform on a single qubit:
rotation. The Mach-Zehnder interferometer we're using can thus be
expressed as this transform on a single qubit:
where the probability of measuring a single photon at the output is
 . Walking through this optical circuit, the states are found to be
. Walking through this optical circuit, the states are found to be

such that

Repeating this  times (so that we use the same average number of
photons as in the coherent state case), we find that the standard
deviation in
times (so that we use the same average number of
photons as in the coherent state case), we find that the standard
deviation in  is
is

Given this, the uncertainty in  is
is

This is the same uncertainty as we obtained for the coherent state
input, but the physical origin is different. Now, we see the noise as
being due to statistical fluctuations of a Bernoulli point process,
one event at a time. The  noise thus comes from the amount
of time the signal is integrated over (assuming a constant rate of
photons). The noise is simply shot noise.
noise thus comes from the amount
of time the signal is integrated over (assuming a constant rate of
photons). The noise is simply shot noise.








![{\displaystyle B={\frac {1}{\sqrt {2}}}\left[{\begin{array}{cc}{1}&{-i}\\{-i}&{1}\end{array}}\right]\,.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4e2d43ef535ef4f670f31b7ce4cfe7a15c1890f8)
![{\displaystyle [a,b]^{T}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/dcbe0f3bd3d712a823544cc4985e4dbd35b6967d)
![{\displaystyle {\frac {1}{\sqrt {2}}}\left[{\begin{array}{c}{a-ib}\\{b-ia}\end{array}}\right]=B\left[{\begin{array}{c}{a}\\{b}\end{array}}\right]\,.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3bcdd91d0e9a05a80b3165137ecd92a63b40bc81)
![{\displaystyle P=\left[{\begin{array}{cc}{1}&{0}\\{0}&{e^{i\phi }}\end{array}}\right]\,.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3f0a39e65796a1cc92d0a2ea729186ca5aca7a58)
![{\displaystyle {\begin{array}{rcl}U&=&BPB\\&=&{\frac {1}{2}}\left[{\begin{array}{cc}{1}&{-i}\\{-i}&{1}\end{array}}\right]\left[{\begin{array}{cc}{1}&{0}\\{0}&{e^{i\phi }}\end{array}}\right]\left[{\begin{array}{cc}{1}&{-i}\\{-i}&{1}\end{array}}\right]\\&=&-ie^{i\phi /2}\left[{\begin{array}{cc}{\sin(\phi /2)}&{\cos(\phi /2)}\\{\cos(\phi /2)}&{-\sin(\phi /2)}\end{array}}\right]\,.\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4aff63aa7808a03191a5315b3f548834ce336f41)


![{\displaystyle \left[{\begin{array}{c}{c}\\{d}\end{array}}\right]=U\left[{\begin{array}{c}{a}\\{b}\end{array}}\right]\,.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c9786a4cebe3997ae8ab2e2a99556bd27a67b002)