Difference between revisions of "Squeezed light interferometry"
imported>Ichuang |
imported>Agrier |
||
| (8 intermediate revisions by one other user not shown) | |||
| Line 1: | Line 1: | ||
| − | + | Heisenberg-limited interferometry can also be accomplished using a | |
| + | variety of states of light, including [[Non-classical_states_of_light|squeezed states]] we studied earlier. Let us explore three configurations here. | ||
| − | + | ==== Vacuum squeezed state input ==== | |
| − | + | ||
| − | + | Because squeezed states can move | |
| − | |||
| − | Vacuum squeezed state input | ||
noise between the <math>x</math> and <math>p</math> quadratures, it is intuitively | noise between the <math>x</math> and <math>p</math> quadratures, it is intuitively | ||
reasonable that a state with low phase noise could be used to provide | reasonable that a state with low phase noise could be used to provide | ||
more accurate measurements of <math>\phi</math> than is possible with a coherent | more accurate measurements of <math>\phi</math> than is possible with a coherent | ||
state, which has equal noise in the two quadratures. | state, which has equal noise in the two quadratures. | ||
| + | |||
It might seem counter-intuitive, however, that we can get to the | It might seem counter-intuitive, however, that we can get to the | ||
Heisenberg limit by replacing not the coherent state input, but | Heisenberg limit by replacing not the coherent state input, but | ||
| Line 49: | Line 49: | ||
is an optimal amount of squeezing, at which point the minimum phase | is an optimal amount of squeezing, at which point the minimum phase | ||
uncertainty goes as <math>{\langle}\Delta\phi_{min} \rangle \sim 1/(n+n_b)</math>, which is | uncertainty goes as <math>{\langle}\Delta\phi_{min} \rangle \sim 1/(n+n_b)</math>, which is | ||
| − | close to the Heisenberg limit. See Caves, {\em Quantum-mechanical | + | close to the Heisenberg limit. See <refbase>184</refbase> |
| + | <!-- Caves, {\em Quantum-mechanical | ||
noise in an interferometer,} Phys. Rev. D, vol~23, p.~1693, 1981, for | noise in an interferometer,} Phys. Rev. D, vol~23, p.~1693, 1981, for | ||
| − | more on this subject. | + | more on this subject. --> |
| − | Yurke state input. | + | |
| + | ==== Yurke state input. ==== | ||
| + | |||
| + | The Heisenberg limit can also be reached | ||
using the Mach-Zehnder interferometer by replacing the input light | using the Mach-Zehnder interferometer by replacing the input light | ||
states with this unusual squeezed state | states with this unusual squeezed state | ||
| Line 79: | Line 83: | ||
\end{array}</math> | \end{array}</math> | ||
In calculating <math> \langle Y{\rangle}</math>, the terms with <math>|n-2{\rangle}</math> drop out, leaving us with | In calculating <math> \langle Y{\rangle}</math>, the terms with <math>|n-2{\rangle}</math> drop out, leaving us with | ||
| − | :<math> | + | :<math> |
\langle Y \rangle = \frac{ \langle n,n-1|Y|n-1,n \rangle + \langle n-1,n|Y|n,n-1{\rangle}}{2} = n | \langle Y \rangle = \frac{ \langle n,n-1|Y|n-1,n \rangle + \langle n-1,n|Y|n,n-1{\rangle}}{2} = n | ||
\,. | \,. | ||
| − | + | </math> | |
Similarly, it is straightforward to show that | Similarly, it is straightforward to show that | ||
:<math>\begin{array}{rcl} | :<math>\begin{array}{rcl} | ||
\langle X \rangle &=& 0 | \langle X \rangle &=& 0 | ||
\\ | \\ | ||
| − | \langle X^2 \rangle &=& \langle n_a^2 - 2n_an_b + n_b^2 \rangle = | + | \langle X^2 \rangle &=& \langle n_a^2 - 2n_an_b + n_b^2 \rangle = 1 |
\,. | \,. | ||
\end{array}</math> | \end{array}</math> | ||
Thus, the uncertainty in <math>\phi</math> is | Thus, the uncertainty in <math>\phi</math> is | ||
:<math> | :<math> | ||
| − | {\langle}\Delta\phi \rangle = \frac{ | + | {\langle}\Delta\phi \rangle = \frac{1}{n} |
\,, | \,, | ||
</math> | </math> | ||
| − | which is | + | which is the Heisenberg limit. |
| + | |||
| + | ==== Yurke state: Experiment? ==== | ||
| + | |||
Given how useful the Yurke state could be for interferometry, it is | Given how useful the Yurke state could be for interferometry, it is | ||
meaningful to consider how such a state might be made. One | meaningful to consider how such a state might be made. One | ||
| Line 104: | Line 111: | ||
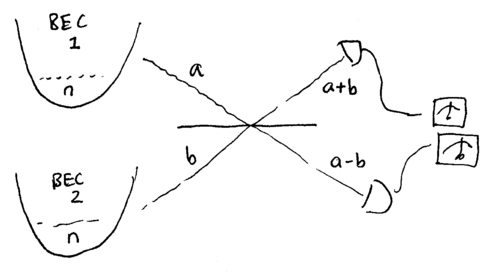
are placed to look for a single atom at the outputs. This is sketched | are placed to look for a single atom at the outputs. This is sketched | ||
below: | below: | ||
| − | ::[[Image:chapter2-quantum-light-part-5-interferometry-l7-yurke-state-bec.png|thumb| | + | ::[[Image:chapter2-quantum-light-part-5-interferometry-l7-yurke-state-bec.png|thumb|500px|none|]] |
If the top detector clicks, then one atom has left the condensates; | If the top detector clicks, then one atom has left the condensates; | ||
however, it is unknown from which it came. The post-measurement | however, it is unknown from which it came. The post-measurement | ||
| Line 117: | Line 124: | ||
is | is | ||
:<math> | :<math> | ||
| − | |\psi \rangle = \frac{|n-1{\rangle}|n \rangle + \sqrt{n}|n{\rangle}|n-1{\rangle}}{\sqrt{2}} | + | |\psi \rangle = \frac{\sqrt{n} |n-1{\rangle}|n \rangle + \sqrt{n}|n{\rangle}|n-1{\rangle}}{\sqrt{2}} |
\,. | \,. | ||
</math> | </math> | ||
This is the Yurke state. If the bottom detector had clicked instead, | This is the Yurke state. If the bottom detector had clicked instead, | ||
we would have obtained <math>|n-1{\rangle}|n{\rangle}-|n{\rangle}|n-1{\rangle}</math> instead, which is also | we would have obtained <math>|n-1{\rangle}|n{\rangle}-|n{\rangle}|n-1{\rangle}</math> instead, which is also | ||
| − | useful. Similar techniques, involving beamsplitter mixed detection of | + | useful. |
| + | |||
| + | Similar techniques, involving beamsplitter mixed detection of | ||
spontaneous emission, can be used to entangle atoms (as we shall see | spontaneous emission, can be used to entangle atoms (as we shall see | ||
later). More about this BEC entanglement method can be found in the | later). More about this BEC entanglement method can be found in the | ||
| − | literature; see, for example, Castin & Dalibard, Phys. Rev. A | + | literature; see, for example, <refbase>185</refbase> |
| − | vol.~55, p.~4330, 1997, for the proposal to create Yurke states; | + | <!-- Castin & Dalibard, Phys. Rev. A |
| − | Vogels, Chin, and Ketterle, Phys. Rev. Lett., vol.~90, p.~030403, 2003, | + | vol.~55, p.~4330, 1997 -->, for the proposal to create Yurke states; |
| + | <refbase>186</refbase><!--Vogels, Chin, and Ketterle, Phys. Rev. Lett., vol.~90, p.~030403, 2003-->, | ||
for an experiment in which a squeezed BEC state was generated, and | for an experiment in which a squeezed BEC state was generated, and | ||
| − | Bouyer and Kasevitch, Phys. Rev. A, vol.~56, p.~R1083, 1997, for a | + | <refbase>187</refbase><!-- Bouyer and Kasevitch, Phys. Rev. A, vol.~56, p.~R1083, 1997-->, for a |
proposal to do Heisenberg limited spectroscopy with BECs. | proposal to do Heisenberg limited spectroscopy with BECs. | ||
| − | |||
Latest revision as of 20:00, 25 February 2009
Heisenberg-limited interferometry can also be accomplished using a variety of states of light, including squeezed states we studied earlier. Let us explore three configurations here.
Vacuum squeezed state input
Because squeezed states can move noise between the and quadratures, it is intuitively reasonable that a state with low phase noise could be used to provide more accurate measurements of than is possible with a coherent state, which has equal noise in the two quadratures.
It might seem counter-intuitive, however, that we can get to the Heisenberg limit by replacing not the coherent state input, but rather, the vacuum state, in the Mach-Zehnder interferometer. This works because at the balanced operating point, the noise in the output is due to fluctuations entering in at the vacuum port. Before, we used as input. Let us now replace this by
where is a squeezed vacuum state. Recall that for the balanced interferometer, the final uncertainty in the phase measurement is
where , and . For the squeezed vacuum + coherent state input, we find
Thus, the uncertainty in the phase measurement is approximately
In the limit of large squeezing, ie , the squeezed vacuum has nonzero average photon number, , so this expression does not vanish to zero. Rather, there is an optimal amount of squeezing, at which point the minimum phase uncertainty goes as , which is close to the Heisenberg limit. See <refbase>184</refbase>
Yurke state input.
The Heisenberg limit can also be reached using the Mach-Zehnder interferometer by replacing the input light states with this unusual squeezed state
This is known as the Yurke state. It happens to be balanced already, and thus instead of operating our interferometer at , we operate it at , such that the output measurement gives , and , and the final uncertainty in the phase measurement is
For the Yurke state,
In calculating , the terms with drop out, leaving us with
Similarly, it is straightforward to show that
Thus, the uncertainty in is
which is the Heisenberg limit.
Yurke state: Experiment?
Given how useful the Yurke state could be for interferometry, it is meaningful to consider how such a state might be made. One interesting proposal starts with two Bose-Einstien condenstates, prepared in a state of definite atom number, which we may model as two number eigenstates . The two condensates are weakly linked through a tunnel, which we may model as a beamsplitter, and detectors are placed to look for a single atom at the outputs. This is sketched below:
If the top detector clicks, then one atom has left the condensates; however, it is unknown from which it came. The post-measurement state, after this single click, is thus
written unnormalized. Normalized, the proper post-measurement state is
This is the Yurke state. If the bottom detector had clicked instead, we would have obtained instead, which is also useful.
Similar techniques, involving beamsplitter mixed detection of spontaneous emission, can be used to entangle atoms (as we shall see later). More about this BEC entanglement method can be found in the literature; see, for example, <refbase>185</refbase> , for the proposal to create Yurke states; <refbase>186</refbase>, for an experiment in which a squeezed BEC state was generated, and <refbase>187</refbase>, for a proposal to do Heisenberg limited spectroscopy with BECs.














![{\displaystyle |\psi _{in}\rangle ={\frac {1}{\sqrt {2}}}\left[{|n-1{\rangle }|n\rangle +|n{\rangle }|n-1\rangle }\right]\,.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/fddaf8eca4a41d65a799f1448a487eafc95e3022)